If →B1,→B2 and →B3 are the magnetic field due to l1,l2 and l3, then in Ampere's circuital law ∮→B.→dl=μ0l,→B is
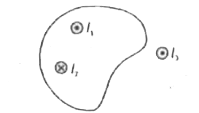
→B=→B1−→B2
→B=→B1+→B2+→B3
→B=→B1−→B2+→B3
→B=→B3
The correct Answer is:B
Topper's Solved these Questions
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTECHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION C
48 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTECHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTECHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - ASSIGNMENT (SECTION A)
34 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)
2 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTECHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)
5 Videos
Similar Questions
Knowledge Check
The base vectors →a1,→a2 and →a3 are given in terms of base vectors →b1,→b2 and →b3 as →a1=2→b1+3→b2−→b3, →a−(2)=→b1−2→b2+2→b3 and →a3=−2→b1+→b2−2→b3, if →F=3→b1−→b2+2→b3, then vector →F in terms of →a1,→a2 and →a3 is
A→F=3→a1+2→a2+5→a3B→F=3→a1−5→a2−2→a3C→F=3→a1+5→a2+3→a3Dnone of theseA: In any magnetic field region the line integral ∮→B.→dl along a closed loop is always zero.
R: The magnetic field →B in the expressioin ∮→B.→dl is due to the currents enclosed only by the loop.AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion then mark (1)BIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion, then mark 2.CIf assertion is true statement but Reason is false, then mark (3)DIf both Assertion and Reason are false statements, then mark (4)
The base vectors →a1,→a2 and →a3 are given in terms of base vectors →b1,→b2 and →b3 as →a1=2→b1+3→b2−→b3, →a2=→b1−2→b2+2→b3 and →a3=2→b1+→b2−2→b3, if →F=3→b1−→b2+2→b3, then vector →F in terms of →a1,→a2 and →a3 is
View SolutionA: In any magnetic field region the line integral ∮→B.→dl along a closed loop is always zero.
R: The magnetic field →B in the expressioin ∮→B.→dl is due to the currents enclosed only by the loop.
View SolutionExplain how Biot-Savart's law enables one to express the Ampere's circuital law in the integral form , viz.,
∮→B.→dl=μ0I
where I is the total current passing through the surface.
View SolutionIf →a,→band→c are any three non-coplanar vectors, then prove that points l1→a+m1→b+n1→c,l2→a+m2→b+n2→c,l3→a+m3→b+n3→c,l4→a+m4→b+n4→c are coplanar if ∣∣ ∣ ∣ ∣∣l1l2l3l4m1m2m3m4n1n2n3n41111∣∣ ∣ ∣ ∣∣=0
View SolutionIf →a,→band→c are any three non-coplanar vectors, then prove that points l1→a+m1→b+n1→c,l2→a+m2→b+n2→c,l3→a+m3→b+n3→c,l4→a+m4→b+n4→c are coplanar if ∣∣ ∣ ∣ ∣∣l1l2l3l4m1m2m3m4n1n2n3n41111∣∣ ∣ ∣ ∣∣=0
View SolutionFind the values of ∮→B.→dl for the loops L1,L2andL3 in Fig. (The sense of →dl is mentioned in the figure)

View Solution

_PHY_E06_049_Q01.png)