The vertices of a parallelogram $A B C D$ are $A (3, 1), B (13, 6), C (13, 21),$ and $D (3, 16) .$ If a line passing through the origin divides the parallelogram into two congruent parts, then the slope of the line is $\frac{11}{12}$ (b) $\frac{11}{8}$ (c) $\frac{25}{8}$ (d) $\frac{13}{8}$

A

$11 / 12$

B

$11 / 8$

C

$25 / 8$

D

$13 / 8$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Slope of line thorugh origin is,
$m = \frac{21 - x}{13} = \frac{x + 1}{3}$
or $63 - 3 x = 13 x + 13$
or $16 x = 50$
or $x = \frac{25}{8}$
Hence, $m = (\frac{25}{8} + 1) \times \frac{1}{3}$
$= \frac{33}{24} = \frac{11}{8}$

|

Updated on:21/07/2023

Class 12 MATHS THREE DIMENSIONAL GEOMETRY

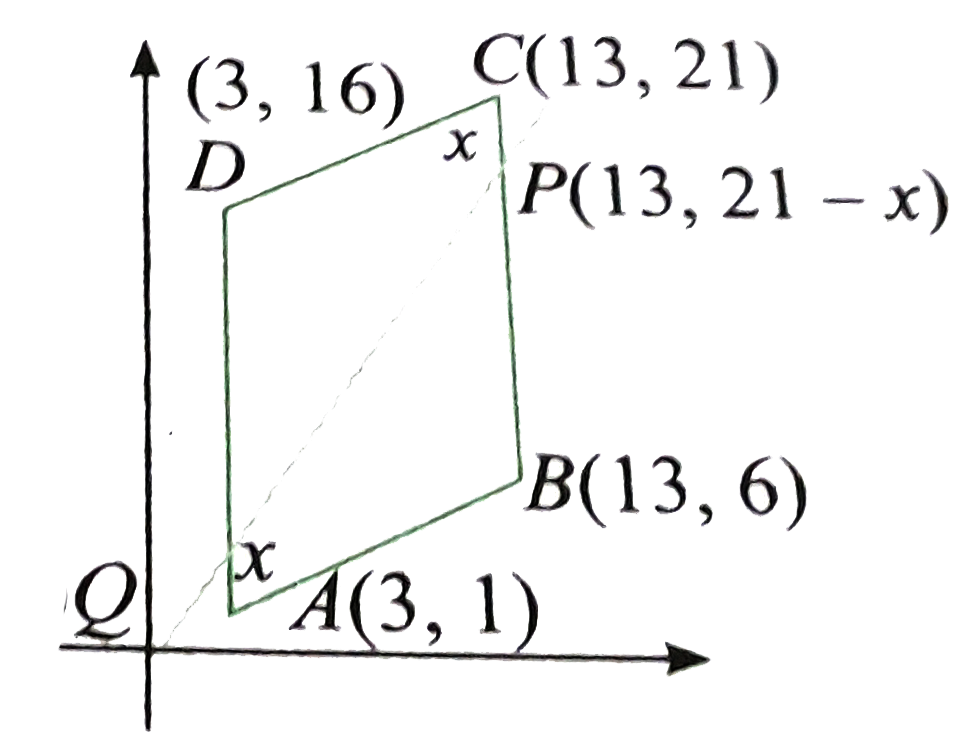
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The vertices of a parallelogram in order are $A (1, 2), B (4, y), C (x, 6)$ and $D (3, 5)$ . Then $(x, y)$ is :
A $(6, 3)$
B $(3, 6)$
C $(5, 6)$
D $(1, 4)$
Question 2 - Select One
The fourth vertex D of a parallelogram $A B C D$ whose three vertices are $A (- 2, 3)$ , $B (6, 7)$ and $C (8, 3)$ is
A $(0, 1)$
B $(0, - 1)$
C $(- 1, 0)$
D $(1, 0)$

CENGAGE-COORDINATE SYSYEM -Exercise (Single)

Home
Ask Doubt
Profile