A uniform rod of mass m and length L lies on the x-axis with its centre of mass at the origin. The locus of the point P in the x-y plane, such that moment of intertia of the rod about an axis parallel to the Z axis passing through P remains constant, is

A

Straight line

B

circle

C

ellipse

D

parabola

Video Solution

|

Answer

Step by step video & image solution for A uniform rod of mass m and length L lies on the x-axis with its centre of mass at the origin. The locus of the point P in the x-y plane, such that moment of intertia of the rod about an axis parallel to the Z axis passing through P remains constant, is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The locus of all the points on theX- Y plane, about which the moment of inertia of the rod along an axis parallel to z axis is same as that about O is [The rod is lying inXZplane]:

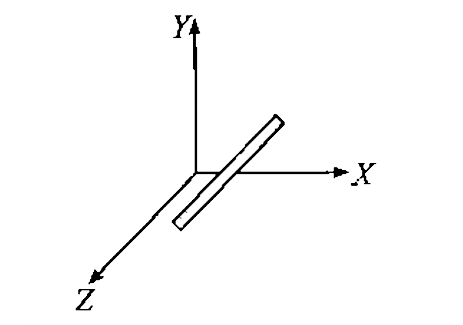AStraight line
BCircle
CParanola
DEllipse
Question 2 - Select One
Two rods of equal lengths(l) and equal mass M are kept along x and y axis respectively such that their centre of mass lie at origin. The moment of inertia about an line y = x, is
A $\frac{M L^{2}}{3}$
B $\frac{m l^{2}}{4}$
C $\frac{m l^{2}}{12}$
D $\frac{m l^{2}}{6}$
Question 3 - Select One
The moment of inertia of a thin uniform rod of mass $M$ and length $L$ about an axis perpendicular to the rod, through its centre is $I$ . The moment of inertia of the rod about an axis perpendicular to rod through its end point is
A $\frac{I}{4}$
B $\frac{I}{2}$
C $2 I$
D $4 I$

BANSAL-TEST PAPERS-PHYSICS

Home
Ask Doubt
Profile