A soild uniform ball of volume V and density ρ floats on the interface of two immiscible liquids as shown in the figure. The density of the upper liquid is ρ1 and that of the lower liquid is ρ2 (ρ2>ρ>ρ1). What fraction of the volume of the ball will be in the upper liquid and what fraction will be in the lower one?

Let V be the volume of the ball and a fraction x of its volume is in the upper liquid. Then, volume of the ball inside the upper liquid = xV and volume of the ball inside the lower liquid = V - xV = (1 - x)V
Due to buoyancy, the net force on the ball in upward direction = [xρ1+(1−x)ρ2]Vg
At equilibrium,
or, xρ1+(1−x)ρ2=ρ
or, x(ρ2−ρ1)=ρ2−ρ
Hence, the fraction of the volume of the ball inside the upper liquid,
x=ρ2−ρρ2−ρ1
The fraction of the ball inside the lower liquid
=1−x=1−ρ2−ρρ2−ρ1=ρ−ρ1ρ2−ρ1
Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - SECTION RELATED QUESTIONS
12 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
37 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
A solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the upper liquid is
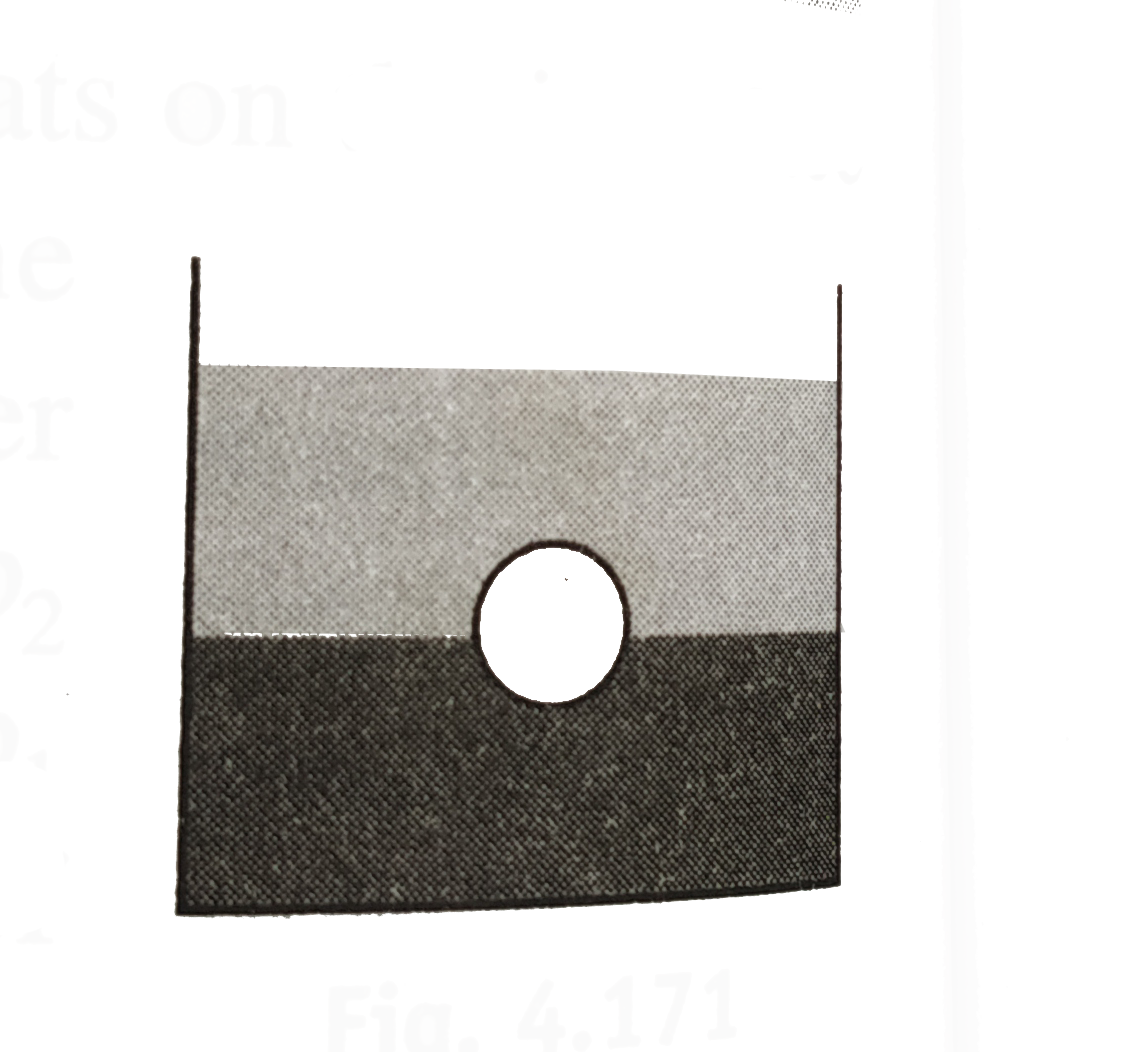
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is

View Solution
Knowledge Check
A solid uniform ball having volume V and density ρ floats at the interface of two unmixable liquids as shown in the figure. The densities of the upper and the lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2 . What fraction of the volume of the ball will be in the lower liquid ?
 Aρ−ρ2ρ1−ρ2Bρρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2
Aρ−ρ2ρ1−ρ2Bρρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2A solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is
 Aρ2ρ1Bρ2−ρρ2−ρ1Cρ−ρ1ρ2−ρ1Dρ1ρ2
Aρ2ρ1Bρ2−ρρ2−ρ1Cρ−ρ1ρ2−ρ1Dρ1ρ2A solid uniform ball having volume V and density ρ floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2. What fractio9n of the volume of the ball will be in the lower liquid.
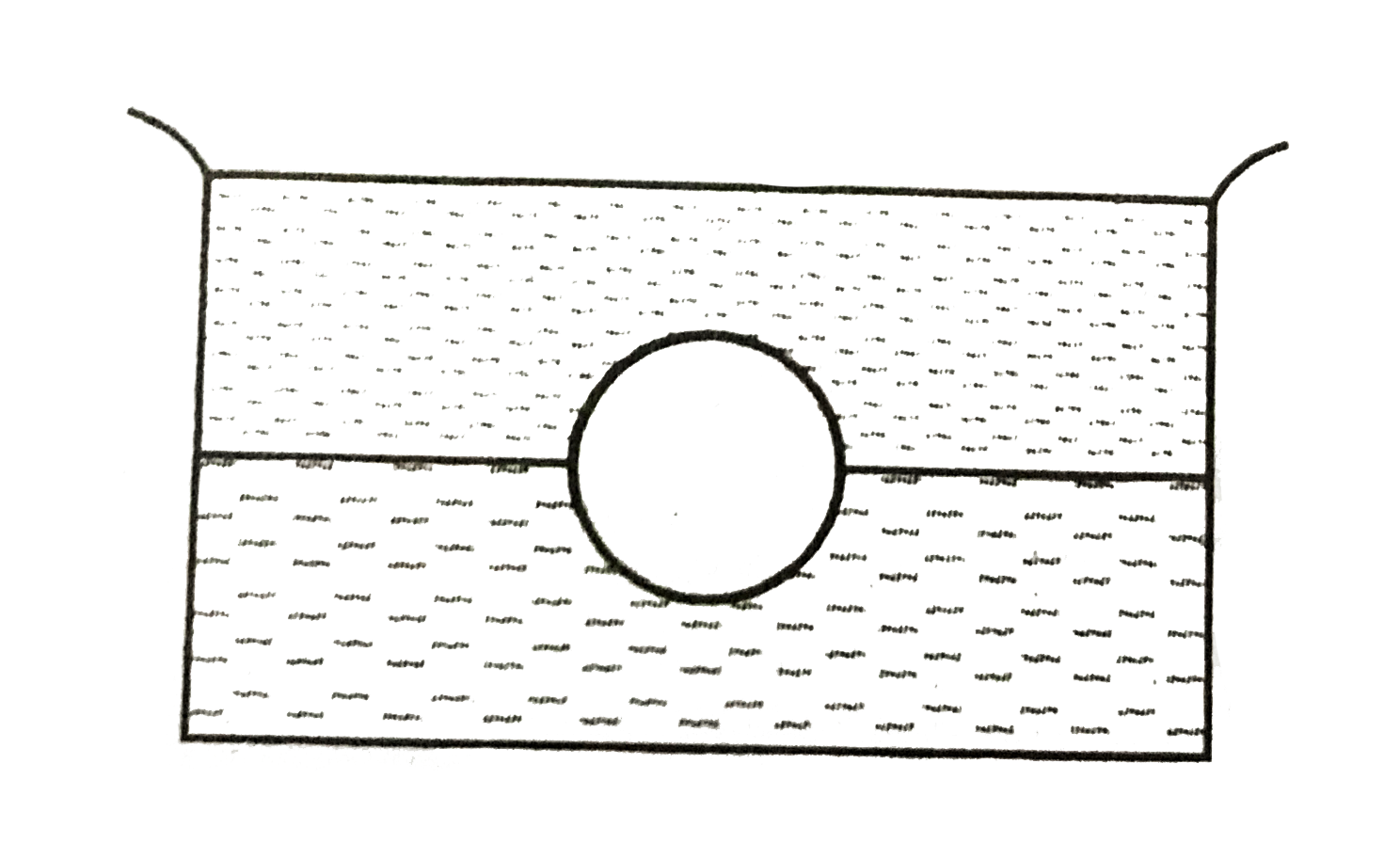 Aρ−ρ2ρ1−ρ2Bρ1ρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2
Aρ−ρ2ρ1−ρ2Bρ1ρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2
A solid uniform ball having volume V and density ρ floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2. What fractio9n of the volume of the ball will be in the lower liquid.

View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids [The specific gravity of the upper liquid is γ/2 and that of the lower liquid is 2γ, where γ is th especific gracity of the solid ball.] The fraction of the volume of the ball that will be in upper liquid is
View SolutionA solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densites ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
View SolutionA Solid uniform ball of volume V floats on the interface of two immiscible liquids . [The specific gravity of the upper liquid is γ/2 and that of the lower liquid is 2γ, where γ is the specific gravity of the solid ball.] The fraction of the volume of the ball that will be in upper liquid is
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids [The specific gravity of the upper liquid is γ/2 and that of the lower liquid is 2γ, where γ is th especific gracity of the solid ball.] The fraction of the volume of the ball that will be in upper liquid is
View Solution
