To what height should a cylindrical vessel be filled with a homogeneous liquid such that the force exerted by the liquid on the wall of the vessel be equal to the force exerted by the liquid on the bottom of the vessel?
Let h be the height of the liquid of density ρ in the cylinder of radius r. The pressure of the liquid column at the bottom of the cylinder is hρg. Therefore, the force exerted by the liquid column on the bottom of the cylinder is F1=pressure×area of the bottom
=hρg×πr2
The pressure of the liquid column on the wall of the vessel varies from zero at the top of the column to hρg at the bottom. Thus, the average pressure on the wall is 0+hρg2=12hρg
Therefore, the force exerted by the liquid column on the wall of the cylinder is
F2=pressure×area of wall=12hρg×2πrh
For F2=F1 we should have
12hρg×2πrh=hρg×πr2
or, h = r
i.e., the height of the liquid column in the cylinder should be equal to the radius of the cylinder.
Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - SECTION RELATED QUESTIONS
12 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
37 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
To what height should a cyclindrical vessel be filled with a homogeneous liquid to make the force with which the liquid pressure on the sides of the vessel equal to the force exerted by the liquid on the bottom of the vessel ?
View SolutionTo what height h should a cylindrical vessel of diameter d be filled with a liquid so that the total force on the vertical surface of the vessel be equal to the force on the bottom-
View Solution
Knowledge Check
To what height should a cyclindrical vessel be filled with a homogeneous liquid to make the force with which the liquid pressure on the sides of the vessel equal to the force exerted by the liquid on the bottom of the vessel ?
Aequal to the radiusBless than radiusCmore than radiusDfour times of radiusTo what height should a cyclindrical vessel be filled with a homogeneous liquid to make the force with which the liquid pressure on the sides of the vessel equal to the force exerted by the liquid on the bottom of the vessel ?
Aequal to the radiusBless than radiusCmore than radiusDfour times of radiusThe height to which a cylindrical vessel be filled with a homogenous liquid, to make the average force with which the liquid presses the side of the vessel equal to the force exerted by the liquid on the bottom of the vessel, is equal to.
Ahalf of the radius of the vesselBradius of the vesselCone-fourth of the radius of the vesselDthree-fourth of the radius of the vessel.
To what height should a cylindrical vessel be filled with a homogenous liquid to make to force on bottom equal to force on side? (R = radius of vessel )
View SolutionIn the following figure shown, a liquid is filled into the vessel up to a height h and the forces exerted against the liquid by the walls of the vessel are shown by arrows, the force being everywhere perpendicular to the walls of the vessel. For this situation mark out the correct statement(s).
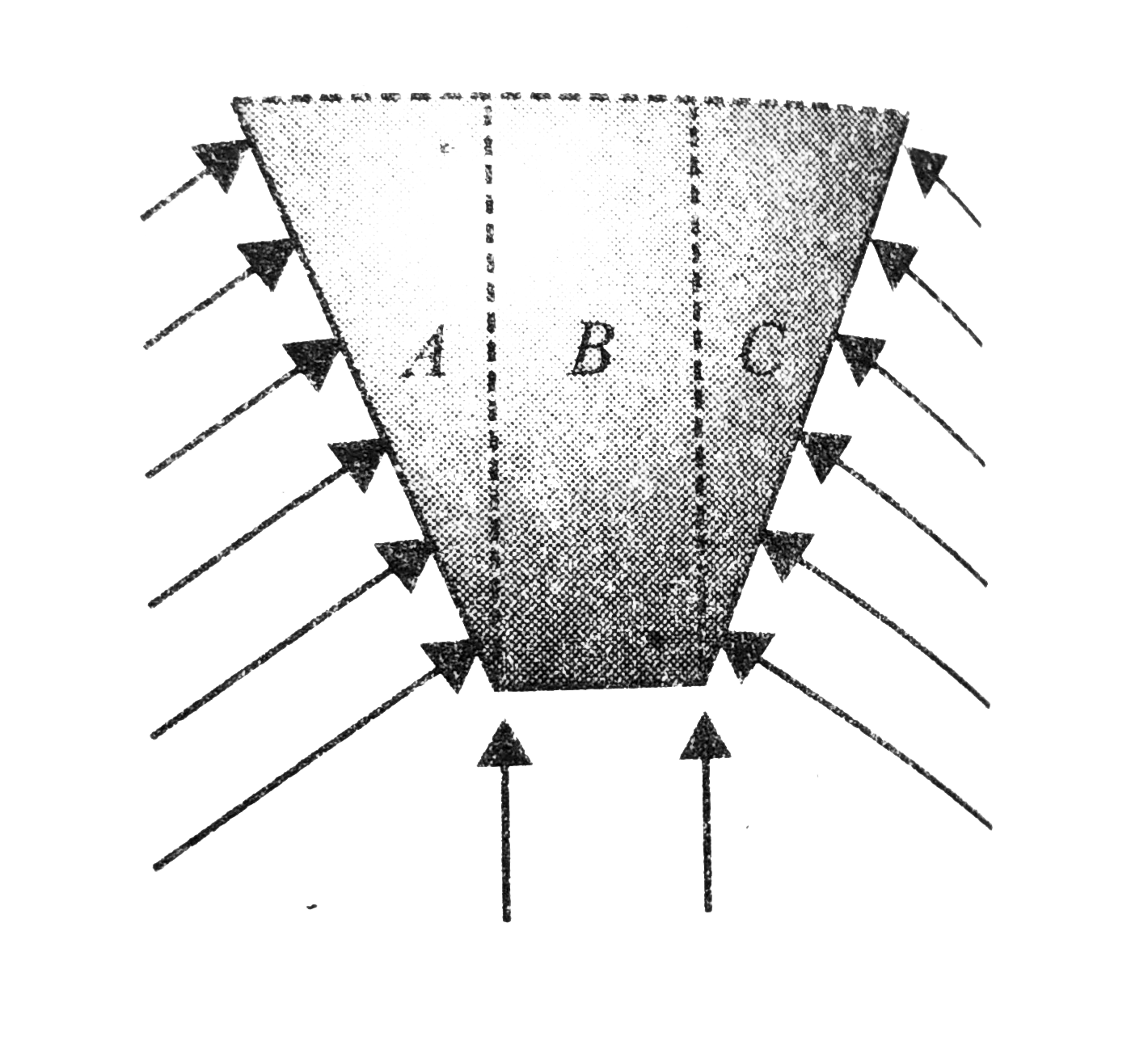
View SolutionTo what height h should a cylindrical vessel of diameter d be filled with a liquid so that the total force on the vertical surface of the vessel be equal to the force on the bottom-
View SolutionA cylindrical vessel of radius r is filled with a homogeneous liquid upto a height h. It is found that the force exerted by the liquid column on the bottom of the cylinder is equal to the force exerted by the liquid on the sides of the cylinder. What is the relation between r and h?
View SolutionIn the following figure shown, a liquid is filled into the vessel up to a height h and the forces exerted against the liquid by the walls of the vessel are shown by arrows, the force being everywhere perpendicular to the walls of the vessel. For this situation mark out the correct statement(s).

View Solution
