A body rests on a rough horizontal plane. A force is applied to the body directed towards the plane at an angle θ with the vertical. The body can be moved along the plane
only if θ is greater than the angle of friction
only if θ is lesser than the angle of friction
only if θ is equal to the angle of friction
for all values of θ
The correct Answer is:A
Topper's Solved these Questions
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - I (C.W)(NEWTON.S LAWS OF MOTION)
8 Videos
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - I (C.W)(IMPULSE)
6 Videos
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EVALUATE YOURSELF - 5
8 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
- MATHEMATICAL REVIEW & PHYSICAL WORLDBOOK - NARAYNACHAPTER - MATHEMATICAL REVIEW & PHYSICAL WORLDEXERCISE - C.U.Q
13 Videos
Similar Questions
A weight W rests on a rough horizontal plane,If the angle of friction is θ,the least force that can move the body along the plane will be
View SolutionA weight 'W' rests on a horizontal plane. What will be the least force required to move the body alone the plane,if the angle of friction is 0?
View Solution
Knowledge Check
A body rests a rough horizontal plane A force is applied to the body directed towards the plane at an angle θ with the vertical. The body can be moved along the plane .
Aonly if θ is greater than the angle of frictionBonly if θ is lesser than the angle of frictionConly if θ is equal to the angle of frictionDfor all values of θAt the moment t=0 force F=kt is applied to a small body of mass m resting on a smooth horizontal plane (k is a constant) The direction of this force always forms an angle θ with the horizontal as shown.
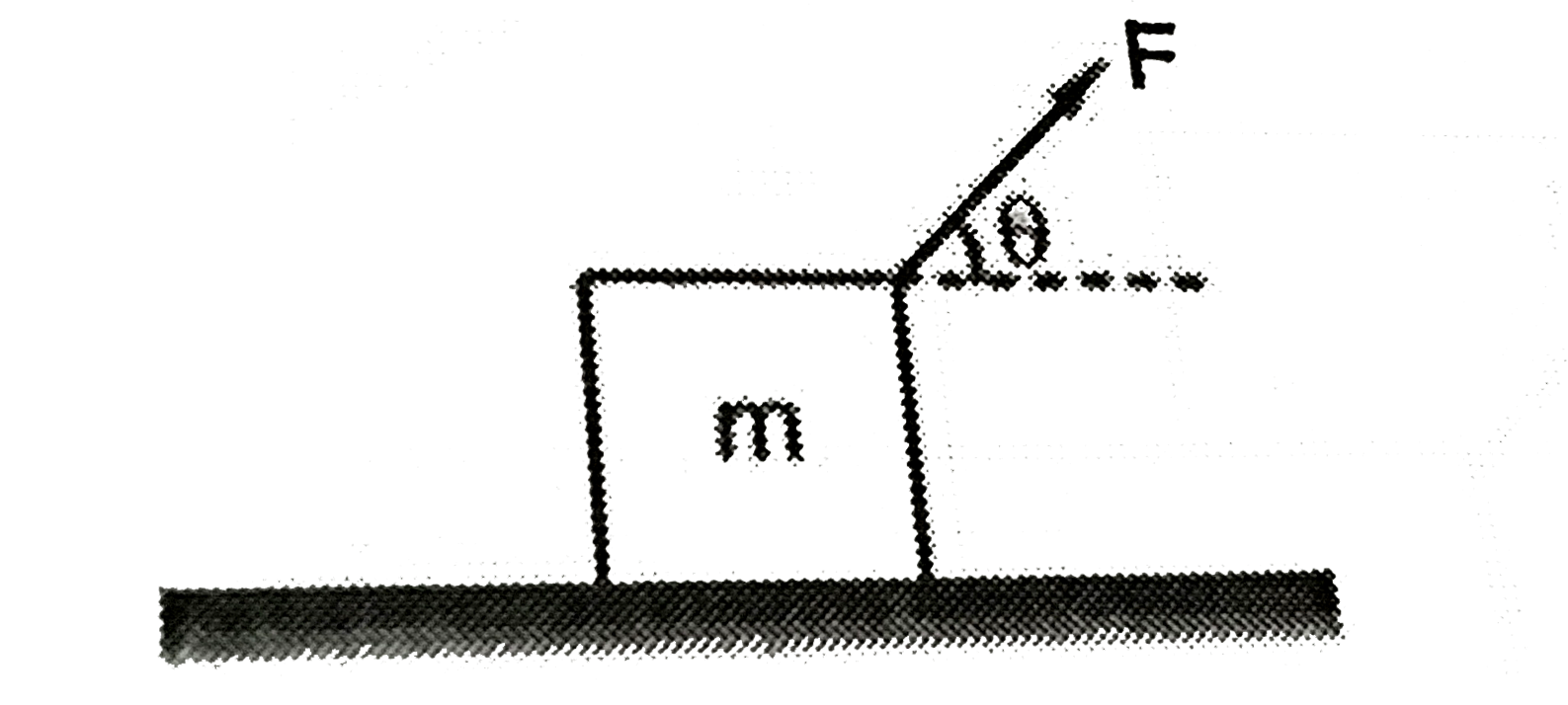
Find the time t at which moment, the body breaks off the plane:Amg2ksinθBmgksinθCmgksin2θD2mgksinθA weight W rests on a rough horizontal plane,If the angle of friction is θ,the least force that can move the body along the plane will be
AWcosθBWtanθCWcotθDWsinθ
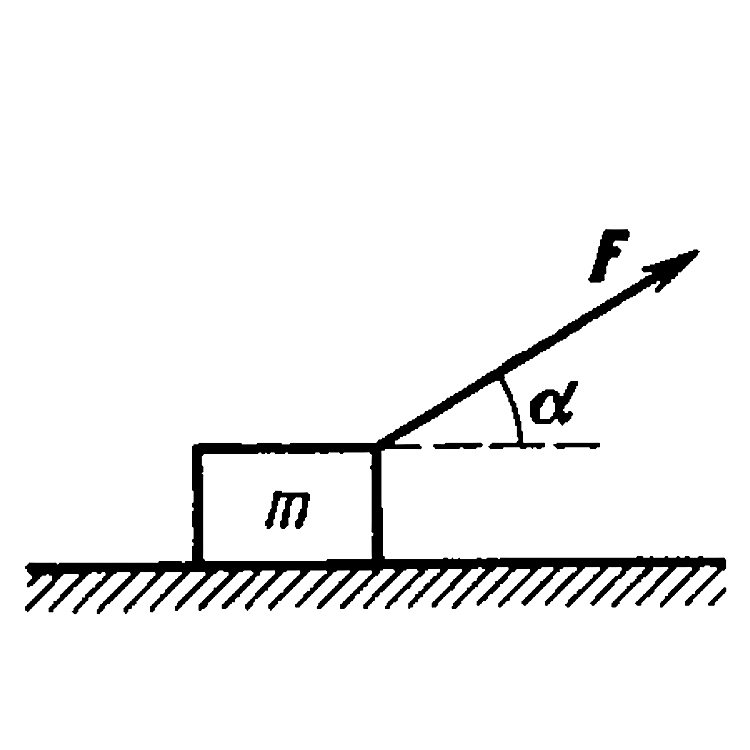
At the moment t=0 the force F=at is applied to a small body of mass m resting on a smooth horizontal plane (a is constant).
The permanent direction of this force forms an angle α with the horizontal (figure). Find:
(a) the velocity of the body at the moment of its breaking off the plane,
(b) the distance traversed by the body up to this moment.
View SolutionA body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the normal force between the block and the plane is,
View SolutionWhen a body is placed on a rough plane inclined at an angle θ to the horizontal, its acceleration is
View SolutionA body of mass 2 kg is placed on rough horizontal plane. The coefficient of friction between body and plane is 0.2 Then,

View SolutionA body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the normal force between the block and the plane is,

View Solution
