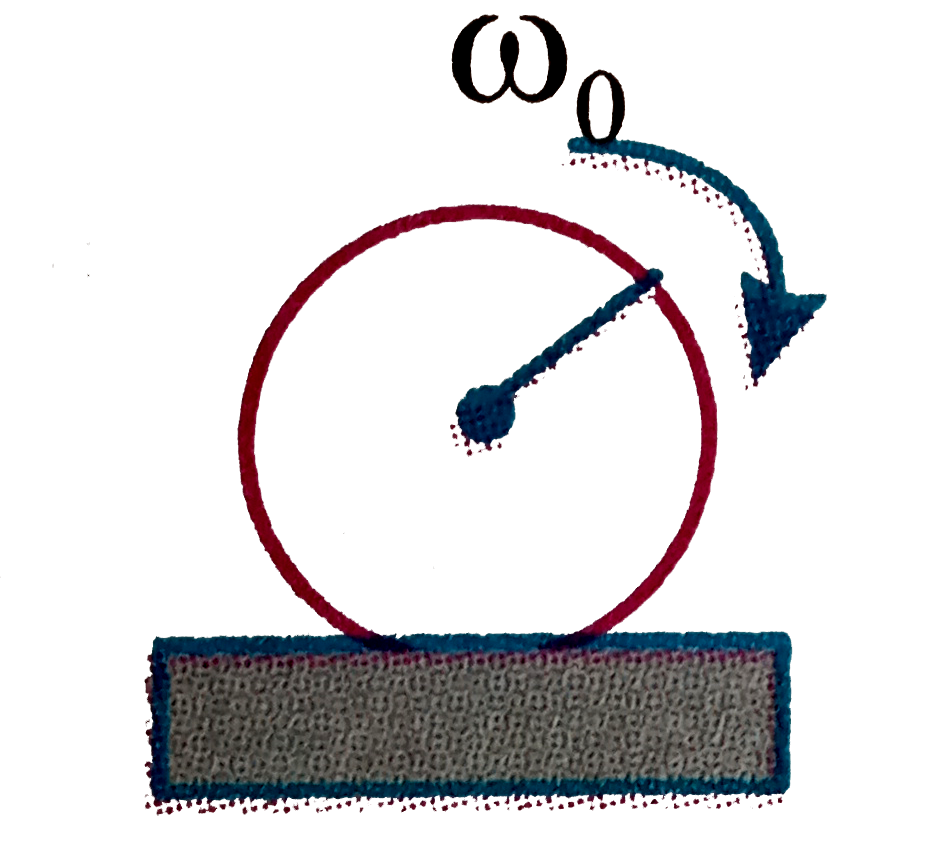
A uniform circular ring of radius R is first rotated about its horizontal axis with an angular velocity $ω_{0}$ and then carefully placed on a rough horizontal surface as hown . The coefficient of friction between the surface and the rings is $u$ . Time after which its angular speed is reduced to $0.5 ω_{0}$ is

A

$\frac{ω_{2} μ R}{2 g}$

B

$\frac{ω_{0} g}{2 μ R}$

C

$\frac{2 ω_{0} R}{μ g}$

D

$\frac{ω_{0} R}{2 μ g}$

Text Solution

Verified by Experts

The correct Answer is:D

Taking the $τ$ about C.M
, $μ g = R α \Rightarrow α = \frac{μ g}{R}$
$ω = ω_{0} - \frac{μ g}{R} t = \frac{ω_{0}}{2}$
$∴ t = \frac{ω_{0} R}{2 μ g}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A uniform circular ring of radius $R$ is first rotated about its horizontal axis with an angular velocity $ω_{0}$ and then carefully placed on a rough horizontal surface as shown. The coefficient of friction between the surface and the rings $μ$ . Time after which its angular speed is reduced to $0.5 ω_{0}$ is

A $\frac{ω_{0} μ R}{2 g}$
B $\frac{ω_{0} g}{2 μ R}$
C $\frac{2 ω_{0} R}{μ g}$
D $\frac{ω_{0} R}{2 μ g}$
Question 2 - Select One
A ring of radius $R$ is first rotated with an angular velocity $ω$ and then carefully placed on a rough horizontal surface. The coefficient of friction between the surface and the ring is $μ$ . Time after which its angular speed is reduced to half is
A $\frac{ω_{0} μ R}{2 g}$
B $\frac{ω_{0} g}{2 μ R}$
C $\frac{2 ω_{0} R}{μ g}$
D $\frac{ω_{0} R}{2 μ g}$
Question 3 - Select One
A ring of radius R is first rotated withan angular velocity w, and then carefully placed on a rough horizontal surface. The coefficient of friction between the.surface and the ring is u. Time after which its angular speed is reduces to half is
A $\frac{2 ω_{0} R}{μ g}$
B $\frac{ω_{0} R}{2 μ g}$
C $\frac{ω_{0} μ R}{2 g}$
D $\frac{ω_{0} R}{2 μ R}$

NARAYNA-SYSTEM OF PARTICLES AND ROTATIONAL MOTION -EXERCISE - II (H.W)

Home
Ask Doubt
Profile