A particle moves in a circular path of radius R with an angular velocity ω=a−bt, where a and b are positive constants and t is time. The magnitude of the acceleration of the particle after time 2ab is
a/R
a2R
R(a2+b)
R√a4+b2
The correct Answer is:D
Find Xcm&Ycm,¯rcm=Xcmˆi+Ycmˆj
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - III
Explore 43 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-VI
Explore 78 Videos
- THERMAL PROPERTIES OF MATTERBook:NARAYNAChapter:THERMAL PROPERTIES OF MATTERExercise:LEVEL - II (H.W.)
Explore 19 Videos
Similar Questions
The linear speed of a particle moving in a circle of radius R varies with time as v=v0−kt, where k is a positive constant. At what time the magnitudes of angular velocity and angular acceleration will be equal ?
View SolutionA particle moves from A to B in a circular path of radius R covering an angle θ as shown in figure. Find the ratio of magnitude of distance and magnitude of displacement of the particle.
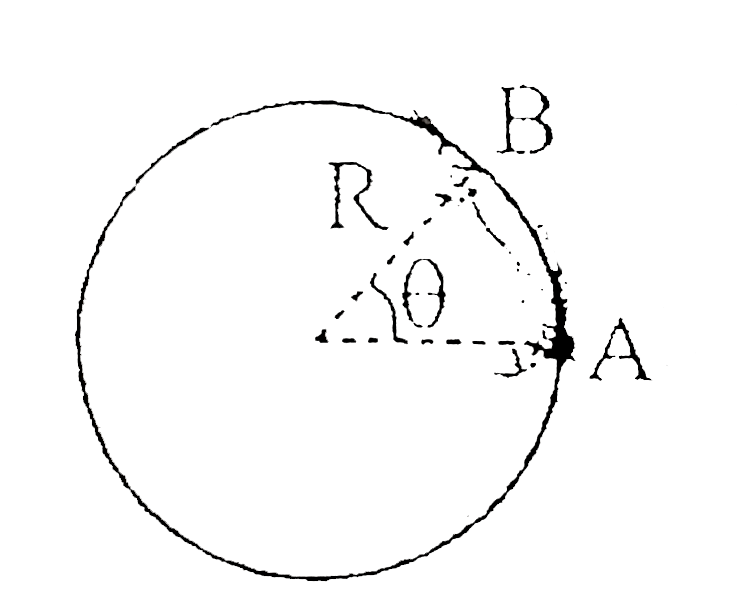
View Solution
Knowledge Check
A particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
Arω2Br/ωCzeroDω/rA particle moving in a circular path of radius (r) with velocity (V), Then centripetal acceleration of the particle is
AV2rBrV2CVrDV2rA particle moving in a circular path of radius (r) with velocity (V), Then centripetal acceleration of the particle is
AV2rBrV2CVrDV2r
the angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionA particle moves along a circle of radius R with a constant angular speed ω. Its displacement (only magnitude) in time t will be
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View SolutionA particle of mass m moves in a circular path of radius r, under the action of force which delivers it constant power p and increases its speed. The angular acceleration of particle at time (t) is proportional
View SolutionA particle of mass m is moving in a circle of radius r. The centripetal acceleration (ac) of the particle varies with the time according to the relation, ac=Kt2, where K is a positive constant and t is the time. The magnitude of the time rate of change of angular momentum of the particle about the centre of the circle is
View Solution