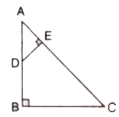
In triangle ABC,∠C=90∘,AC=6andBC=8. Points D and E are on ¯¯¯¯¯¯ABand¯¯¯¯¯¯BC , respectively, and ∠BED=90∘. If DE=4, then BD=
Step by step video & image solution for In triangle ABC, /_C = 90^@, AC = 6 and BC = 8. Points D and E are on bar(AB) and bar(BC) , respectively, and /_BED=90^@. If DE = 4, then BD = by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
In △ABC,∠B=90∘, P,Q and R are the mid-points of ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC and ¯¯¯¯¯¯AC respectively. Then which of the following is true?
AA,P,Q and R are concylic points.BB,P,R and Q are concylic points.CC,Q,P and R are concyclic points.DAll of theseABC is an equilateral triangle. P and Q are two points on ¯¯¯¯¯¯AB and ¯¯¯¯¯¯AC respectively such that ¯¯¯¯¯¯PQ∣∣¯¯¯¯¯¯BC. If ¯¯¯¯¯¯PQ=5cm, then area of ΔAPQ is :
A254 sq. cmB25√3 sq. cmC25√34 sq. cmD25√3 sq. cmIn triangle ABC,∠BAC=90∘and AD is perpendicular to BC. If AD=6cmand BD=4cm, then the length of BC is:
A10 cmB12 cmC13 cmD15 cm.
In △ ABC, D is the midpoint of BC. DFZAB and DE ⊥ AC, where points F and E lie on AB and AC respectively. If DF = DE, prove that /\ ABC is an isosceles triangle.
View SolutionIn a ABC,D and E are points on sides ABandAC respectively such that BD=CE. If ∠B=∠C, show that DEBC.
View SolutionIn the ΔABC, D and E are points on side AB and AC respectively such that DE II BC. If AE=2cm, AD=3cm and BD=4.5cm, then find CE.
View SolutionIn triangle ABC , sides AB and AC are extended to D and E, respectively, such that AB=BD and AC =CE,F∈dDE,ifBC=6 cm.
View SolutionIn ΔABC,∠B=90∘. If points D and E are on side BC such that BD=DE=EC, then which is true?
View Solution