Question

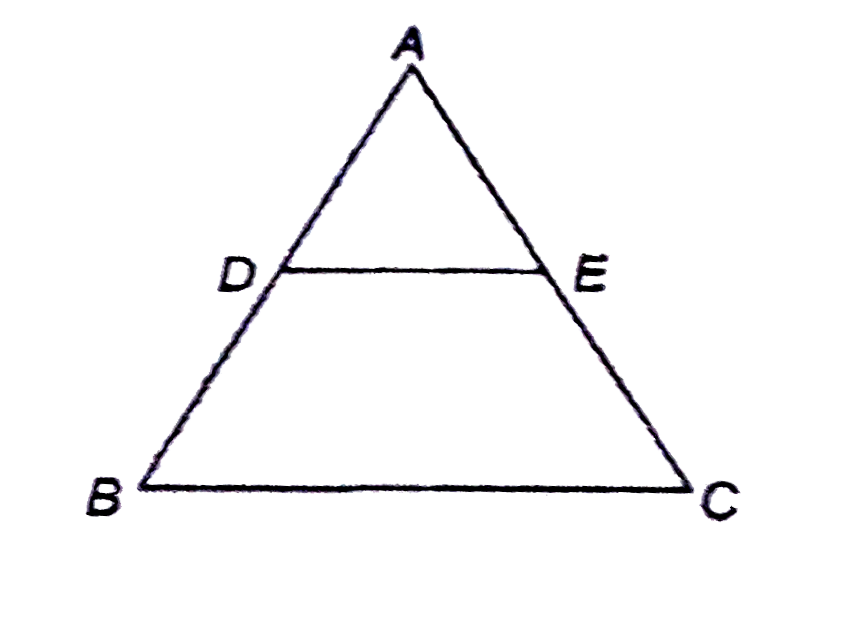
In the $\Delta$ABC, D and E are points on side AB and AC respectively such that DE II BC. If AE=2cm, AD=3cm and BD=4.5cm, then find CE.

Explanation

To solve the problem, we will use the concept of similar triangles. Since DE is parallel to BC, triangles ABC and ADE are similar.

1. Identify the Given Information:
- AE = 2 cm
- AD = 3 cm
- BD = 4.5 cm
- We need to find CE.

2. Use the Property of Similar Triangles:
Since DE || BC, we can say that triangles ABC and ADE are similar by the AA (Angle-Angle) criterion. This gives us the proportion:
\( \frac{AB}{AD} = \frac{AC}{AE} \)

3. Calculate AB:
We know that:
\( AB = AD + BD = 3 \text{ cm} + 4.5 \text{ cm} = 7.5 \text{ cm} \)

4. Set Up the Proportion:
From the similarity of triangles, we have:
\( \frac{AB}{AD} = \frac{AC}{AE} \)
Plugging in the values we know:
\( \frac{7.5}{3} = \frac{AC}{2} \)

5. Cross-Multiply to Solve for AC:
\( 7.5 \cdot 2 = 3 \cdot AC \)
\( 15 = 3 \cdot AC \)
\( AC = \frac{15}{3} = 5 \text{ cm} \)

6. Find CE:
Now we know that:
\( AC = AE + CE \)
Therefore:
\( 5 = 2 + CE \)
Solving for CE gives:
\( CE = 5 - 2 = 3 \text{ cm} \)

Final Answer:
CE = 3 cm