Question

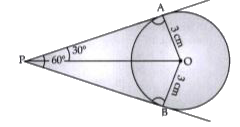
If two tangents are inclined at ${60}^{\circ }$ are drawn to a circle of radius 3cm then
find length of each tangent.
OR
PQ is a tangent to a circle with centre O at point P. If $\Delta$OPQ is an isosceles
triangle, then find $\angle$OQP.

Explanation

To solve the first part of the question, we need to find the length of each tangent drawn to a circle of radius 3 cm, where the two tangents are inclined at an angle of 60 degrees.

1. Understanding the Geometry:
- We have a circle with center O and radius 3 cm.
- Let A and B be the points where the tangents touch the circle, and C be the point from which the tangents are drawn.
- The angle between the two tangents (∠ACB) is given as 60 degrees.

2. Using the Properties of Tangents:
- The radius at the point of contact is perpendicular to the tangent. Therefore, ∠OAC = 90 degrees and ∠OBC = 90 degrees.
- Since the tangents are drawn from the same external point C, the lengths of the tangents CA and CB are equal.

3. Finding the Angles:
- Since the tangents are inclined at 60 degrees, we can divide this angle into two equal parts: ∠CAB = ∠CBA = 30 degrees.

4. Applying the Right Triangle:
- In triangle OAC, we have:
- OA = radius = 3 cm
- OC = length of the tangent (which we will denote as x)
- ∠OAC = 90 degrees
- ∠CAB = 30 degrees

5. Using Trigonometric Ratios:
- In triangle OAC, we can use the tangent function:
\( \tan(30^\circ) = \frac{OA}{AC} = \frac{3}{x} \)
- We know that \(\tan(30^\circ) = \frac{1}{\sqrt{3}}\), so we can set up the equation:
\( \frac{1}{\sqrt{3}} = \frac{3}{x} \)

6. Cross Multiplying:
- Cross multiplying gives us:
\( x = 3\sqrt{3} \text{ cm} \)

7. Conclusion:
- Therefore, the length of each tangent is \(3\sqrt{3}\) cm.