Three objects, A (a solid sphere), B (a thin circular disc) and C (a circular ring), each have the same mass M and radius R. They all spin with the same angular speed ω about their own symmetry axes. The amounts of work (W) required to bring them to rest, would satisfy the relation
WB>WA>WC
WA>WB>WC
WC>WB>WA
WA>WC>WB
The correct Answer is:C
According to work-energy theorem we can write
ΔW=ΔK.E.
ΔW=12Iω2
ΔW∝I
WA:WB:WC=25MR2:12MR2:MR2
=25:12:1=4:5:10
⇒WC>WB>WA
Hence option (c) is correct.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other State Boards for Engineering Entrance) )48videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS MULTIPLE CHOICE QUESTIONS (with more than one correct answer) )35videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Numerical Problems)20videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
One circular ring and one circular disc, both are having the same mass and radius. The ratio of their moments of inertia about the axes passing through their centres and perpendicular to their planes, will be
View SolutionA thin circular right of mass M and radius R is rotating about its axis with an angular speed ω0 two particles each of mass m are now attached at diametrically opposite points. Find new angular speed of the ring.
View Solution
Knowledge Check
- Question 1 - Select One
There object, A : (a solid sphere), B : (a thin circular disk) and C : (a circular ring), each have the same mass M and radius R. They all spin with the same angular speed ω about their own symmetry axes. The amount of work (W) required ot bring them to rest, would satisfy the relation
AWB>WA>WCBWA>WB>WCCWC>WB>WADWA>WC>WB - Question 2 - Select One
There object, A : (a solid sphere), B : (a thin circular disk) and C : (a circular ring), each have the same mass M and radius R. They all spin with the same angular speed ω about their own symmetry axes. The amount of work (W) required ot bring them to rest, would satisfy the relation
AWB>WA>WCBWB>WC>WBCWA>WB>WCDWC>WA>WA - Question 3 - Select One
A thin circular ring of mass per unit length p and radius r is rotating at an angular speed ω as shown in figure. The tension in the ring is
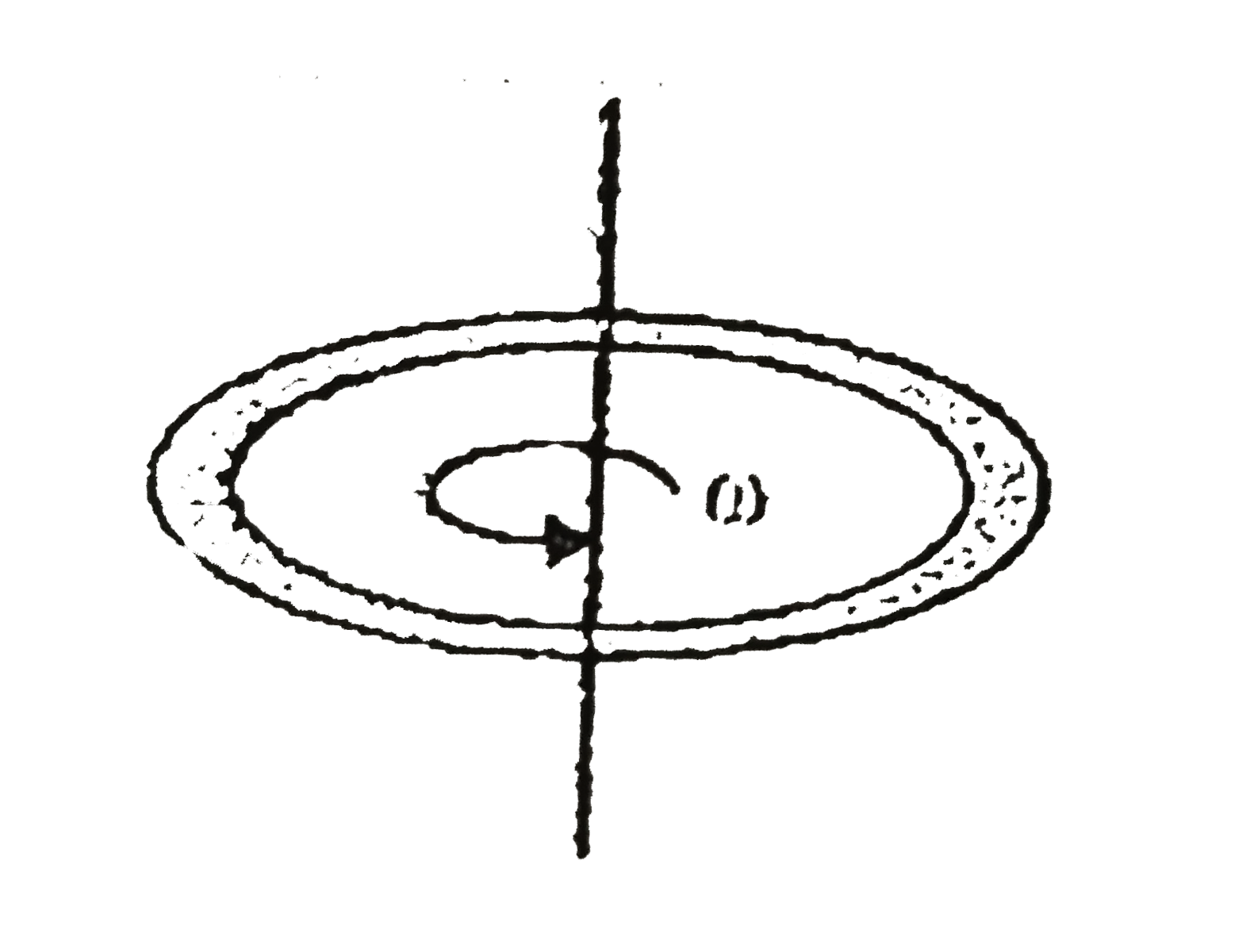 Apω2r2/2Bpω2r2Cpω2r2/3DNone
Apω2r2/2Bpω2r2Cpω2r2/3DNone
A thin circular ring of mass M and radius r is rotating about its ais with an angular speed ω. Two particles having mas m each are now attached at diametrically opposite points. The angular speed of the ring will become
View SolutionA circular ring and circular disc of same radius have equal moments of inertia about axes through their centres and perpendicular to their planes. The ratio of their masses is
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
View SolutionA rough disc of mass m rotates freely with an angular velocity ω. If another rough disc of mass m2 and same radius but spinning in opposite sense with angular speed ω is kept on the first disc. Then:
View SolutionA circular coil of radius r is placed in a uniform magnetic field B. The magnetic field is normal to the plane of the coil is rotated at an angular speed of ω, about its own axis , then the induced emf in the coil is __
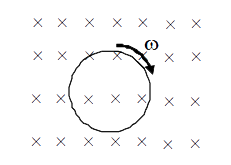 View Solution
View Solution

