In the figure given below there is one parallel plate capacitor with two types of dielectric materials in between the plates. Calculate net capacitance of the given system.
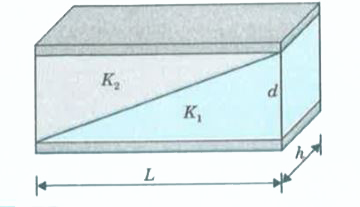
We can see that thickness of each dielectric material between the plates is continuously changing as we move from one end to the other and we need to apply math of intergration to get the capacitance of the system.
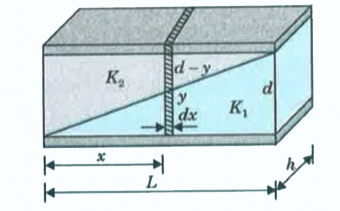
Let us select a segment of width dx at a distance x from the left end of the capacitor as shown in the figure. Let y represent thickness of dielectric material with dielectric constant K1 for this capacitor segment and d-y the thickness of dielectric material with dielectric constant K2. We can easily understand that capacitor segment can be further divided in two segments dC1 and dC2 connected is series with each other to represent the complete capacitor segment as shown in the figure.
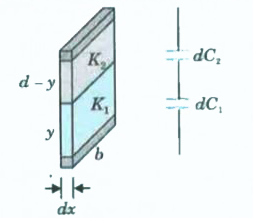
Let dC be the capacitance of selected capacitor segment then we can write the following:
1dC=1dC1+1dC2 ...(i)
further we can write:
dC1=K1ε0(bdx)y&dC2=K2ε0(bdx)d−y ...(ii)
Substituting the valuco of dC1anddC2 from equation (ii) in equation (i) we get the following:
1dC=yK1ε0(bdx)+d−yK2ε0(bdx)
⇒1dC=K2y+K1(d−y)K1K2ε0(bdx)=K1d+(K2−K1)yK1K2ε0(bdx)
⇒dC=K1K2ε0(bdx)K1d+(K2−K1)y ...(iii)
All capacitor segments are supposed to be connected in parallel to each other so we can integrate dC in equation (iii) to get the overall capacitance of this system. But before we can actually integrate equation (iii), we first need to decide on the variable of integration. Is it x or y? Both are related to each other but using geometry we can wirte the following equation:
dL=yx⇒dx=Lddy ...(iv)
We can substitue dx in terms of dy from equation (iv) into equation (iii) to get the following equation.
dC=K1K2ε0bLd(dyK1d+(K2−K1)y) ...(v)
We can understand that variable y changes from zero to d as x changes from 0 to L. Now using suitable limits of integration we can integrate equation (v) to get the net capacitance of this system.
C=K1K2ε0bLd∫y=dy=0dyK1d+(K2−K1)y
⇒C=K1K2ε0bLd[1K2−K1ln{K1d+(K2+K1)y}]d0
⇒C=K1K2ε0bLd(K2−K1)lnK2K1
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Textbook Exercise)
11 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Additional Exercises)
23 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Conceptual Questions
37 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
Define capacitance of a parallel plate capacitor,Derive an expression for the capacitance of a parallel plate capacitor with dielectric of thickness "t," where "(t
View SolutionTwo parallel plate of area A and separated by two different dielectric as shown in the figure. The net capacitance is

View Solution
Knowledge Check
A parallel plate capacitor is filled with dielectrics. What is capacitance?
 A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)A parallel plate capacitor with air between plates (dielectric constant K =2) has a capacitance C. If the air is removed, then capacitance of the capacitor is
AC√2BC2C√2CD2CA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
Abecomes zeroBremains the sameCdecreasesDincreases
Two parallel plate of area A and separated by two different dielectric as shown in the figure. The net capacitance is

View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionIn figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?

View SolutionAssertion (A): When the distance between the parallel plates of a parallel plate capacitor is halved and the dielectric constant of the dielectric used is made three times, then the capacitance becomes three times.
Reason (R ): Capacitance does not depend on the nature of material.
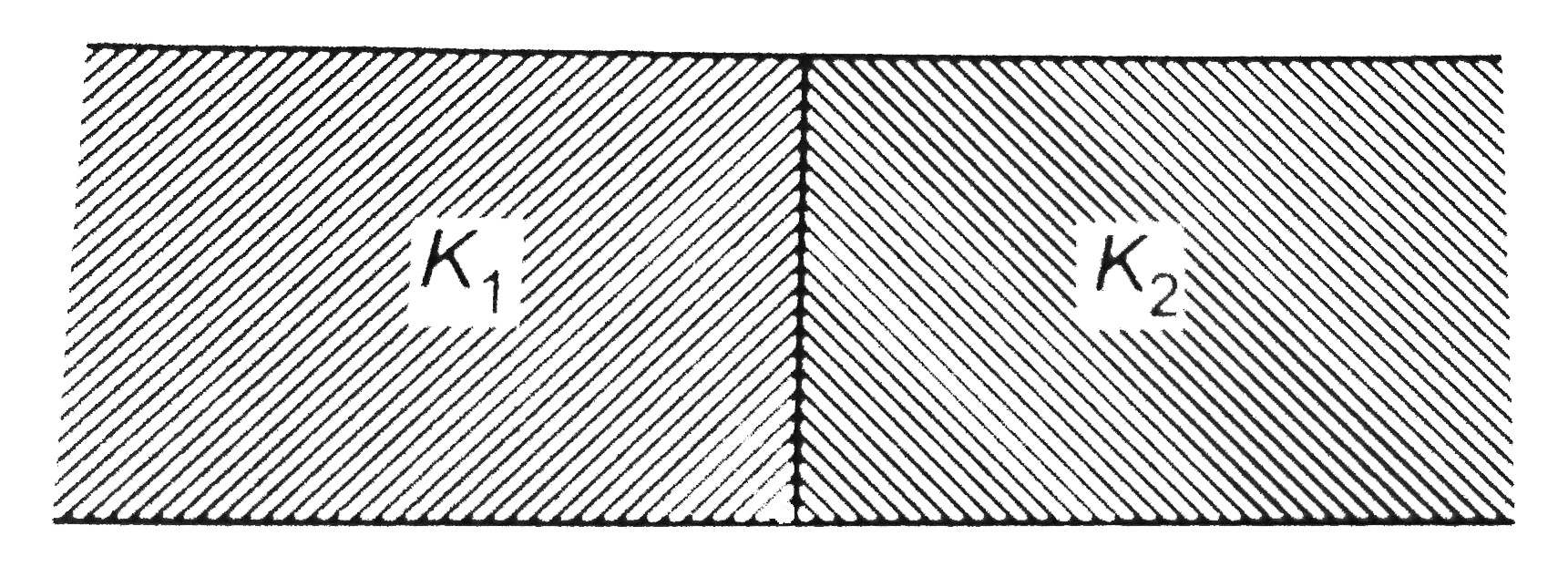
View SolutionTwo material having the dielectric constans K1 and K2 are filled between two parallel plates of a capacitor.
where area of each plate is A and the distance between the plates is d.
The capacitor of the capacitor is:
View Solution
