An observer in a laboratory starts with $N_{0}$ nuclei of a radioactive sample and keeps on observing the number (N) of leftover nuclei at regular intervals of 10 minutes each. She prepares the following table on the basis of her observation.

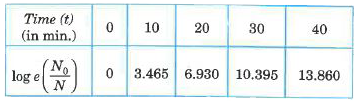
Use this data to plot a graph of ${log}_{e} (N_{0} / N)$ vs time (t) and calculate the
(a) decay constant
(b) half-life of the given sample.

Video Solution

Text Solution

Verified by Experts

The graph between ${log}_{e} (N_{0} / N)$ and time t is plotted as below:

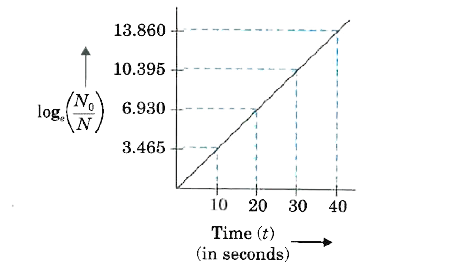
(a) We know that
$\frac{{log}_{e} N_{0}}{N} = λ t$
$\Rightarrow λ = \frac{1}{t} log e \frac{N_{0}}{N}$
When t = 10 min.
${log}_{e} (\frac{N_{0}}{N}) = 3.465$ (from graph)
$∴ λ = \frac{1}{10} \times 3.465 = 0.3465 - 1 min$
(b) Half-life,
$T_{1 / 2} = \frac{0.693}{λ} = \frac{0.693}{0.3465} = 2$ minutes.

Updated on:21/07/2023

A radioactive sample has initial concentration $N_{0}$ of nuclei. Then,
Athe number of undecayed nuclei present in the sample decays exponentially with time
Bthe activity (R ) of the sample at any instant is directly proportional to the number of undecayed nuclei present in the sample at that tiem
Cthe number of decayed nuclei grows exponentially with time
Dthe number of decayed nuclei grows lineraly with time
For a radioactive sample, at given instant, number of active nuclei is N and its decay constant is $λ$ then the incorrect relation is–
A $N λ$ = activity at given instant
B $λ =$ decay probability per unit time of nucleus
CAfter the next $\frac{1}{λ}$ time interval active nuclei in the sample will be $N (1 - \frac{1}{e})$
DThe half life of the sample $= \frac{l n 2}{λ}$
If $N_{0}$ is the number of radioactive nuclei initially present , then the number of nuclei remaining undecayed at the end of nth half life is
A $2^{- n} N_{0}$
B $2^{- n} N_{0}$
C $2^{- n} N_{0}$
D $2^{- n} N_{0}$