A uniform solid disc of radius R and mass m is rotating about its horizontal axis AB with a uniform angular speed of ω0. It is placed on the horizontal table in contact with its surface. If the coefficient of kinetic frinction is μk, calculate the time taken for the rolling to begin.

Rω0μkg(1+mR2I)
ω0μkgR(1+mR2I)
gRω0μk(1+mR2I)
Rω0μkg(mR2I)
The correct Answer is:A
Here the acceleration produced in C.M. due to friction is given by
a=Fm=μkmgm=μkg
Also the angular acceleration produced by the torque due to force of friction will be
α=τI=μkmgRI
If v is the velocity of C.M.
then v=u+at
=0+μkg.t
Also ω=ω0+αt
=ω0−μkmgRI.t
Now for Rolling without slipping
ω=v/R
∴vR=ω0−μgRI.t
or μkgtR=ω0−μkmgRI.t(∵v=μkgt)
Now ω0=μkgtR+μkmgRI.t
=t.{μkgR(1+mR2I)}
∴t=ω0μkgR[1+mR2I]
=ω0Rμkg(1+mR2I)
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - LEVEL-III (MCQ)
10 Videos
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - RCQ
8 Videos
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - RCQ
8 Videos
- RAY OPTICSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICSEXERCISE - RECENT COMPETITIVE QUESTION
32 Videos
- SOLIDS & SEMICONDUCTOR DEVICESBOOK - MODERN PUBLICATIONCHAPTER - SOLIDS & SEMICONDUCTOR DEVICESEXERCISE - Revision Test
28 Videos
Similar Questions
Knowledge Check
A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
AωoμkR2gBωog2μkRC2ωoRμkgDωoR2μkgA ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μkR2gBω0g2μkRC2ω0RμkgDω0R2μkgA ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μ1R2gBωaR2μgRC2ω0RωkgDω0R2μgg
A uniform disc of mass m and radius R is rotated about an axis passing through its center and perpendicular to its plane with an angular velocity ω. It is placed on a rough horizontal plane with the axis of the disc keeping vertical. Coefficient of friction between the disc and the surface is μ, find
(a). The time when disc stops rotating
(b). The angle rotated by the disc before stopping.
View SolutionA disc of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a horizontal table. The coefficient of kinetic friction is μk.
(a) What was the velocity of its centre of mass before being brought in contact with the table ?
(b) What happens to the linear velocity of a point on its rim when placed in contact with the table ?
(c ) What happens to the linear speed of the centre of mass when disc is placed in contact with the table ?
(d) Which force i sresponsible for the effects in (b) and (c ).
(e) What condition should be satisfied for rolling to begin ?
(f) Calculate the time taken for the rolling to begin.
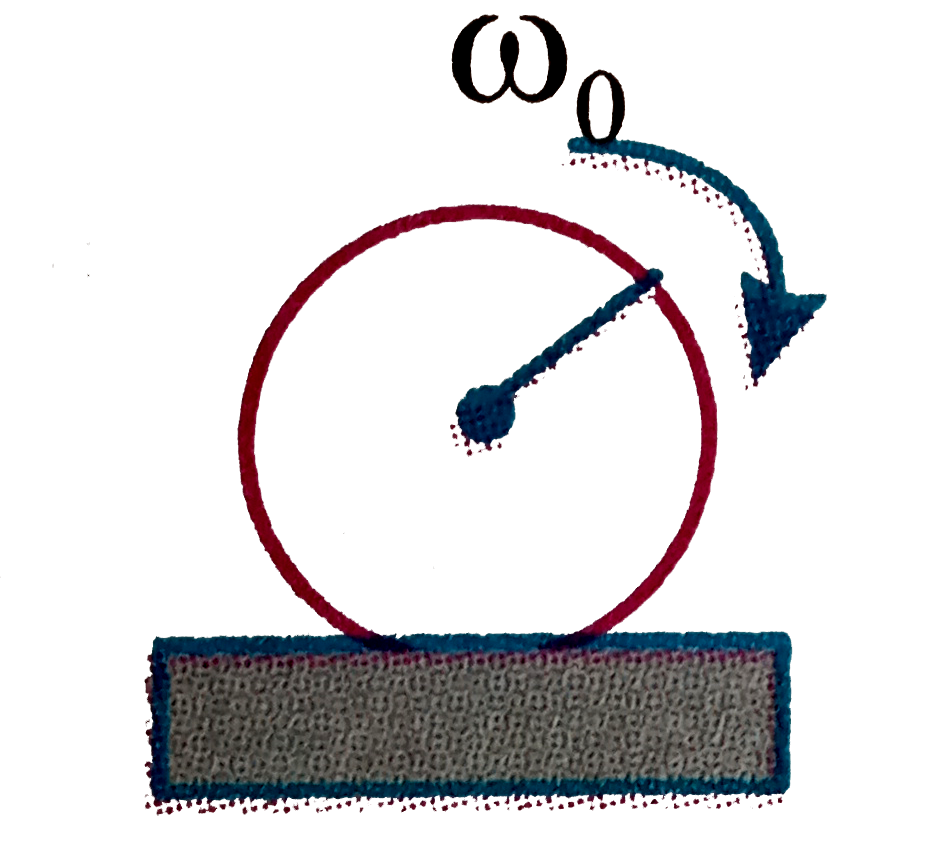
View SolutionA uniform circular ring of radius R is first rotated about its horizontal axis with an angular velocity ω0 and then carefully placed on a rough horizontal surface as shown. The coefficient of friction between the surface and the rings μ. Time after which its angular speed is reduced to 0.5ω0 is
View SolutionIf a disc of mass 400 gm is rolling on a horizontal surface with uniform speed of 2 m/s, then its rolling kinetic energy will be
View SolutionA solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
View Solution
