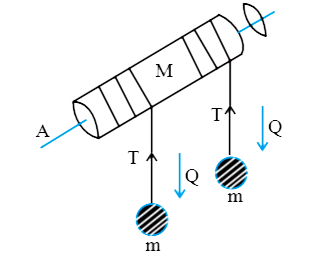
A uniform solid cylinder having mass M and radius R rotates about a horizontal frictionless axle AB. With the help of massless inextensible two ropes are suspended two masses with equal mass m by wrapping the ropes on the cylinder as shown. What will be the tension in each of the rope when masses are released from height 'h' above the ground?

MmgM+4m
MmgM+3m
MmgM+2m
None of these
The correct Answer is:A
Here the equation of motion for children will be
2mg−2T=2ma ...(i)
Also the torque acting is
τ=T.R+T.R=2TR
2TR=Iα=12MR2.aR
or 4TM=a ...(ii)
from (i) T=ma=mg
or T+m×4TM=mg
MT+4mT=Mmg
or T(M+4m)=Mmg
T=MmgM+4m
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - LEVEL-III (MCQ)
10 Videos
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - RCQ
8 Videos
- ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - ROTATIONAL MOTIONEXERCISE - RCQ
8 Videos
- RAY OPTICSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICSEXERCISE - RECENT COMPETITIVE QUESTION
32 Videos
- SOLIDS & SEMICONDUCTOR DEVICESBOOK - MODERN PUBLICATIONCHAPTER - SOLIDS & SEMICONDUCTOR DEVICESEXERCISE - Revision Test
28 Videos
Similar Questions
A uniform solid cylinder of mass M and of radius R rotates about a frictionless axle. Two masses are suspended from a rope wrapped arround the cylinder. If the system is released from rest, the tension in each rope is?
View SolutionA solid cylinder of mass M and of radius R is fixed on a frictionless axle over a well. A rope negligible mass is wrapped around the cylinder. A bucket of uniform mass m is suspended from it. The linear acceleration bucket will:
View Solution
Knowledge Check
A uniform solid cylinder of mass M and of radius R rotates about a frictionless axle. Two masses are suspended from a rope wrapped arround the cylinder. If the system is released from rest, the tension in each rope is?
 AMmgM+mBMmgM+2mCMmgM+3mDMmgM+4m
AMmgM+mBMmgM+2mCMmgM+3mDMmgM+4mA solid cylinder of mass M and of radius R is fixed on a frictionless axle over a well. A rope negligible mass is wrapped around the cylinder. A bucket of uniform mass m is suspended from it. The linear acceleration bucket will:
A4mgM+2mB4mgM+4mC2mgM+mD2mgM+2mA solid cylinder of mass M and of radius R is fixed on a frictionless axle over a well. A rope negligible mass is wrapped around the cylinder. A bucket of uniform mass m is suspended from it. The linear acceleration bucket will:
AmgM+mB2mgM+mC2mgM+2mD2mg2M+m
A cylinder of mass M and radius R is to be raised on a horizontal step of height h=R3. Cylinder is pulled by a rope as shown in figure. Assuming no slipping, the minimum value of force F to raise the cylinder is
_PHY_E05_246_Q01.png)
View SolutionA solid uniform cylinder of mass M and radius R is pivoted at its centre free to rotate about horizontal axis. A massless inextensible string is wrapped around it, and attached to a block of mass m which is initially at a height h above the floor. The acceleration due to gravity is g, directed downward. The block is released from rest. By what total angle does it rotate Δθ (in radians)
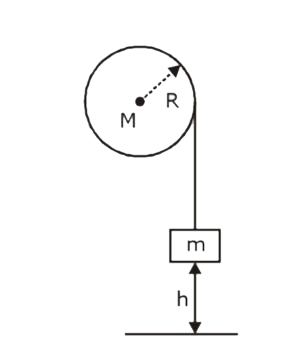
View Solution
A block of mass 2 m is pulled along a horizontal frictionless surface by a rope of mass m as shown in the figure given above. What force is exerted by the rope on the block ?
View SolutionA block of mass M is pulled along a horizontal frictionless surface by a rope of mass m as shown in A horizontal force F is applied to one end of the rope. Find
(a) the acceleration of the rope and block
(b) the force that the rope exerts on the block
(c) the tension in the rope at its mid point

View SolutionA block of mass 2 m is pulled along a horizontal frictionless surface by a rope of mass m as shown in the figure given. What force is exerted by the rope on the block ?

View Solution
