The velocity -time graph of harmonic oscillator is shown in the given figure. The frequency of oscillation is :

25 Hz
50 Hz
12.25 Hz
none of these.
The correct Answer is:A
Velocity becomes maximum to zero in time T/4.
∴ T4=0.01 s.
T=0.04 s.
v=1T=10.04=25 Hz
Hence correct choice is (a).
Topper's Solved these Questions
- OSCILLATIONSBook:MODERN PUBLICATIONChapter:OSCILLATIONSExercise:MCQ (LEVEL-II)
Explore 62 Videos
- OSCILLATIONSBook:MODERN PUBLICATIONChapter:OSCILLATIONSExercise:MCQ (LEVEL-III) Questions From AIEEE/JEE Examination
Explore 10 Videos
- MOCK TEST-3Book:MODERN PUBLICATIONChapter:MOCK TEST-3Exercise:MCQs
Explore 49 Videos
- PROPERTIES OF MATTERBook:MODERN PUBLICATIONChapter:PROPERTIES OF MATTERExercise:RECENT COMPETITIVE QUESTIONS
Explore 17 Videos
Similar Questions
Knowledge Check
The velocity-time diagram of a harmonic oscillator is shown in the adjoining figure. The frequency of oscillation is
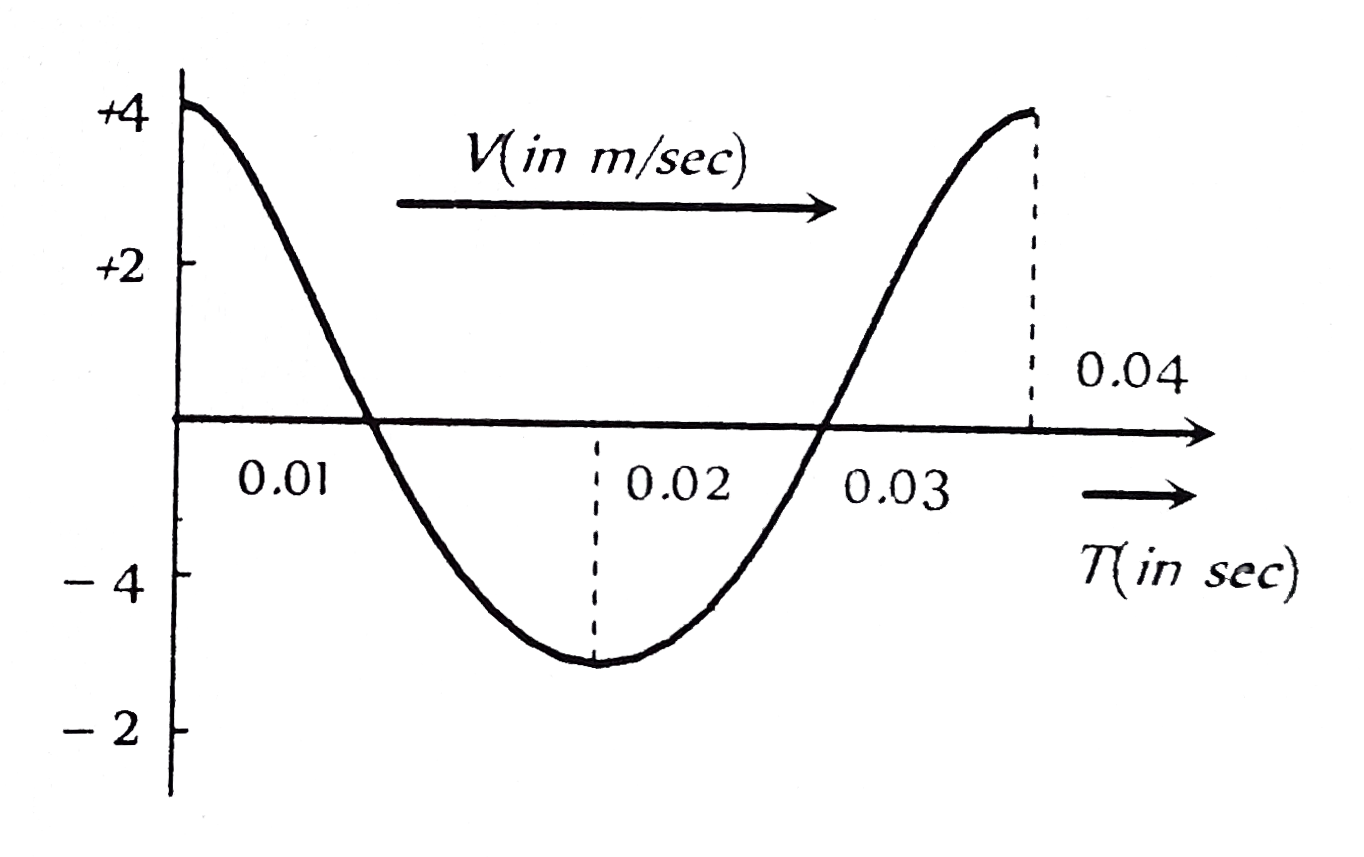 A25 HzB50 HzC12.25 HzD33.3 Hz
A25 HzB50 HzC12.25 HzD33.3 HzThe frequency of LC oscillation is given by
Af=12π√LCBf=2π√LCCf=1π√LCDf=14πε0√LCFrom the given figure find the frequency of oscillation of the mass m
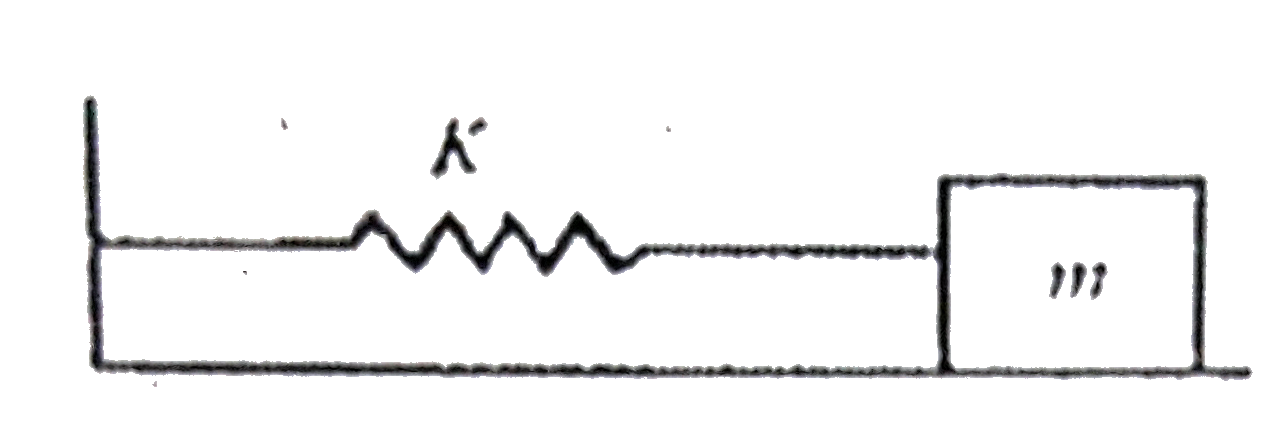 An=12π√KmBn=12π√K22mCn=2π√m2KDn=12n√K2m
An=12π√KmBn=12π√K22mCn=2π√m2KDn=12n√K2m
The acceleration-displacement graph of a particle executing simple harmonic motion is shown in the figure. The frequency of oscillation is
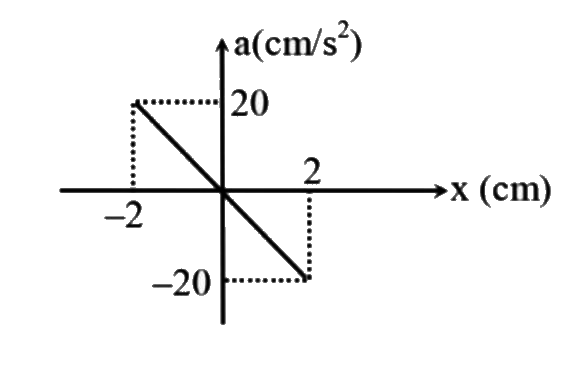
View SolutionThe acceleration-displacement graph of a particle executing simple harmonic motion is shown in the figure. The frequency of oscillation is

View SolutionAcceleration displacement (a-x) graph of a particle executing S.H.M. is shown in the figure. The frequency of oscillation is (tanθ=8)
View SolutionThe acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

View SolutionThe acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

View Solution
