An α− particle and a proton are accelerated from rest by a potential difference of 100 V. After this, their de Broglie wavelengths are and λa and λp respectively. The ratio λpλa to the nearest integer, is ............... Solution in Kannada
1
2
3
4
The correct Answer is:C
λ=h√2mE ∴λ∝1√mE
∴λpλa=√maEampEp=√4×2EE=2√2≈3
∴Ep=eVandE∝=2eV
Topper's Solved these Questions
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-II)
63 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)
9 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
Similar Questions
Maximum frequency of the photon produced by the union of a proton and a antiproton is (given : mp=1.67×10−27kg):
View SolutionEnergy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
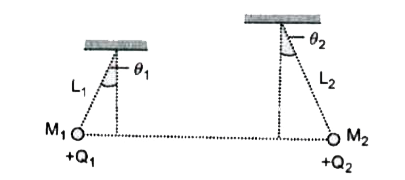
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionTwo particles of de-broglie wavelength lamda_(x) and lamda_(y) are moving in opposite direction. Find debroglie wavelength after perfectly inelastic collision:
View SolutionIf alpha particle, proton and electron move with the same momentum, them their respective de Broglie wavelengths lamda_(alpha),lamda_(p),lamda_(e) are related as
View SolutionThe energy of a photon is equal to the kinetic energy of a proton. If λ1 is the de-Broglie wavelength of a proton, λ2 the wavelength associated with the proton and if the energy of the photon is E, then (λ1/λ2) is proportional to
View SolutionAn electron of mass m_(e) and a proton of mass m_(p) are moving with the same speed. The ratio of their de-Broglie wavelength lamda_(e)//lamda_(p) is
View SolutionEnergy of a photon = E , kinetic energy of a proton is also the same as energy of the photon. Corresponding wavelength of the photon and de Broglie wavelength of the proton are λ1andλ2, respectively. What is the relation between E and the ratio of λ1andλ2?
View SolutionA particle A of mass m and initial velocity v collides with a particle of B of mass m/2 which is at rest. The collision is head-on and elastic. The ratio of the de Broglie wavelengths lamda_(A) "to" lamda_(B) after collision is
View SolutionIf lamda_(0) is the De-Broglie wavelength for a proton accelerated through a potential difference of 100 V, De- Broglie wavelength for prop- particle accelerated through the same potential difference is
View SolutionElectron are accelerated through a potential difference V and protons are accelerated through a potential difference of 4 V. The de-Broglie wavelength are lamda_e and lamda_p for electrons and protons, respectively The ratio of lamda_e/lamda_p is given by ( given , m_e is mass of electron and m_p is mass of proton )
View SolutionIf λ1 and λ2 denote the de-Broglie wavelength of two particles with same masses but charges in the ratio of 1:2 after they are accelerated from rest through the same potential difference, then
View SolutionThe energy of a photon is equal to the K.E. of a proton. The energy of a photon is E. If lamda_(1) and lamda_(2) are the de broglie wavelengths of proton and photon, then (lamda_(1))/(lamda_(2)) is proportional to :
View SolutionTwo particles of de-broglie wavelength lamda_(x) and lamda_(y) are moving in opposite direction. Find debroglie wavelength after perfectly inelastic collision:
View Solution
