In the following (not a scale) , if BC=20cm and ∠BAD=∠CAD , then BD = ___________. 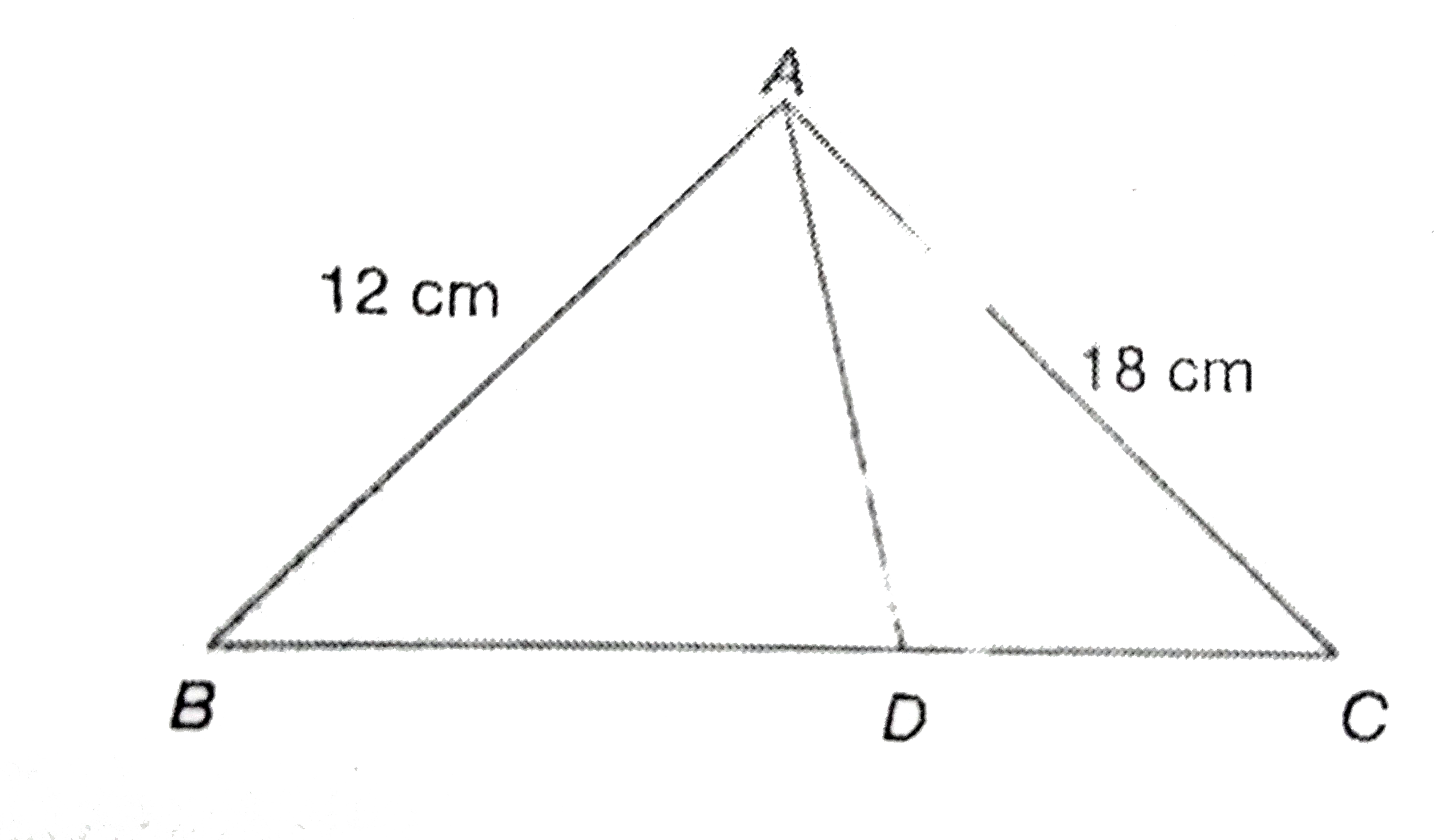
The correct Answer is:8cm
Step by step video & image solution for In the following (not a scale) , if BC=20cm and angleBAD =angle CAD , then BD = ___________. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - Short answer type questions13videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - Essay type questions3videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - Concept Application Level -315videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - BANKING EXERCISE - LEVEL 310videos
BANKING
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIMITSEXERCISE - LEVEL-315videos
LIMITS
Similar Questions
In the following figure (not to scale), ∠DAB+∠CBA=90∘, BC = AD, AB = 20 cm, CD = 10 cm, then the area of the quadrilateral ABCD is:
निम्नलिखित आकृति (जो पैमाने के अनुसार नहीं है) में, ∠DAB+∠CBA=90∘, BC = AD, AB = 20 सेमी, CD = 10 सेमी है, तो चतुर्भुज ABCD का का क्षेत्रफल कितना होगा? View Solution
View SolutionABC is a triangle and D is a point on the side BC. If BC = 12 cm, BD = 9 cm and ∠ADC=∠BAC, then the length of AC is equal to
View Solution
Knowledge Check
- Question 1 - Select One
In the following figure ( not to scale), ∠ADC=60∘,∠BAD=80∘ and ∠EBC=2∠PDE. Find ∠APE.
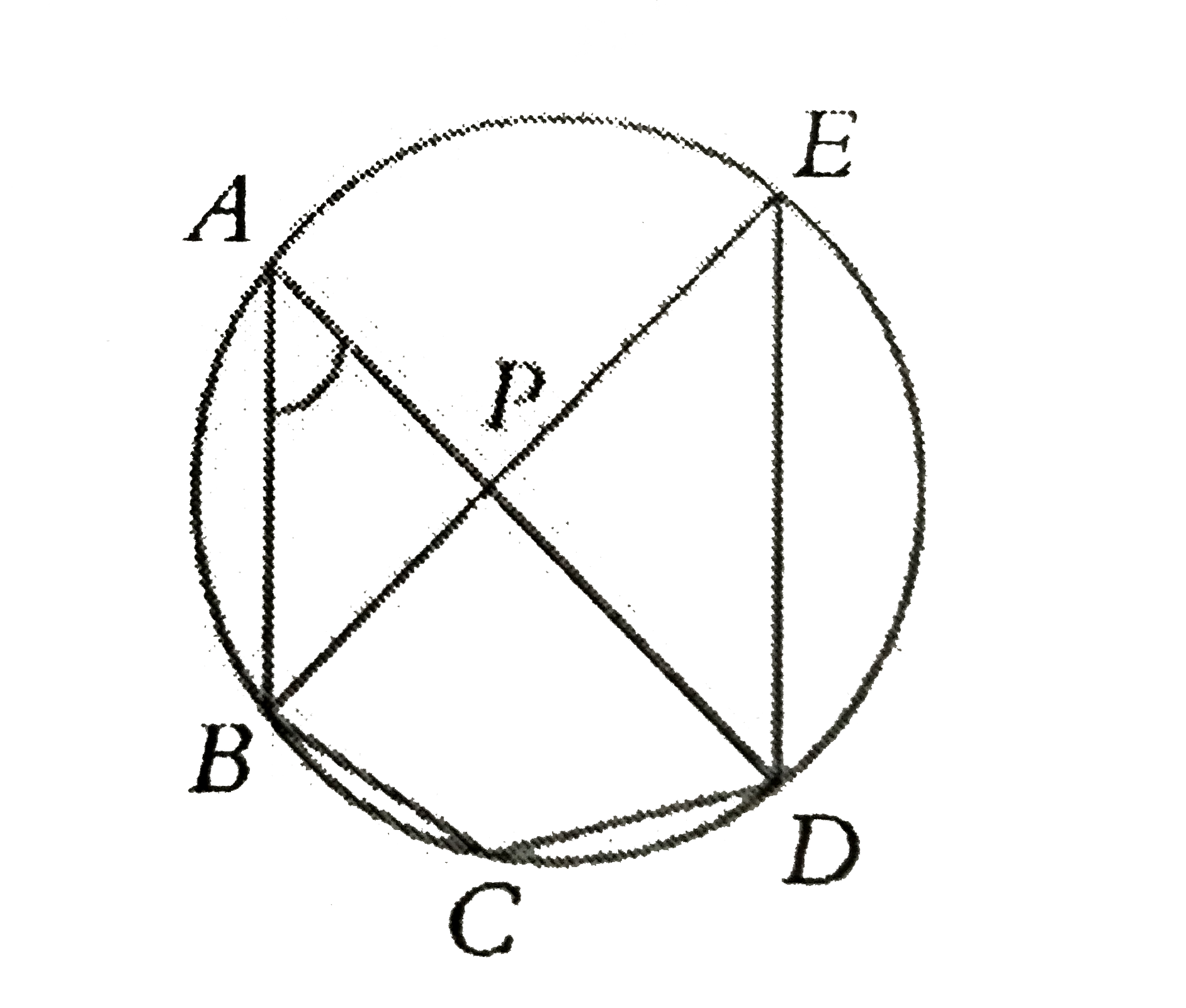 A60∘B80∘C120∘D140∘
A60∘B80∘C120∘D140∘ - Question 2 - Select One

In the figure above (not to scale)
DAE∣∣BC,∠BAD=(2x−70)∘
∠ACB(x−20)∘and∠FAG=(2x+20)∘. Find ∠EACA40∘B25∘C30∘D35∘ - Question 3 - Select One
In the figure, if DE || BC, AD = 3 cm, BD = 4 cm, and BC = 14 cm, then DE equals :
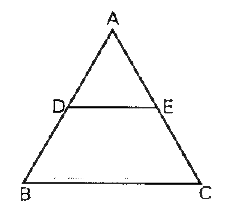 A7 cmB6 cmC4 cmD3 cm
A7 cmB6 cmC4 cmD3 cm
In this ΔABC,BD=8cm,BC=20cm,CD=16cmand∠CBD=∠CAB. Find the perimeter of ΔBDA.
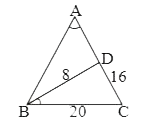 View Solution
View SolutionIn the triangle ABC, D is on BC ∠ADB=90∘,∠ABC=45∘, AD= 10cm, AC = 20 cm. then BC is equal to:
View SolutionABC is a Δ BD = 8 BC = 20 CD = 16 ∠CBD=∠CAB find perimeter of ABC
View SolutionIn the following figure, ∠ACB=90∘ and CD⊥AB, If AD=4cm and BD=9cm then the ratio of BC:AC is
View SolutionAB is diameter ∠ABC=65∘∠CAD=45∘∠DCA=
 View Solution
View Solution

