Diagonal $A C$ of a rectangle $A B C D$ is produced to the point $E$ such that $A C : C E = 2 : 1, A B = 8 c m$ and $B C = 6 c m$ . Find the length of $D E$ .

A

$2 \sqrt{19}$ $c m$

B

$15$ $c m$

C

$3 \sqrt{17}$ $c m$

D

$13$ $c m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Given $A B = 8 c m and B C = 6 c m$
$∴ A C = \sqrt{8^{2} + 6^{2}} = 10 c m$
And also given $A C : C E = 2 : 1$
Produce BC to meet DE at the point P
Aa CP is parallel to AD,
$Δ E C P ~ Δ E A D$ (1)
$∴ \frac{C P}{A D} = \frac{C E}{A E} = \frac{C P}{6} = \frac{1}{3}$
$\Rightarrow C P = 2 c m$ .
$Δ C P D$ is a right triangle.
$∴ D P = \sqrt{C D^{2} + C P^{2}} = \sqrt{68} = 2 \sqrt{17} c m$
But $D P : P E = 2 : 1 (o m (1))$
$P E = \sqrt{170 c m}$
$∴ D E = D P + P E$
$= 2 \sqrt{17} + \sqrt{17} = 3 \sqrt{17} c m$ .

|

Updated on:21/07/2023

Class 10 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In the given figure AB = 12, AC = 15 cm and AD = 6 cm. $B C ∣ ∣ D E$ , find the length of AE

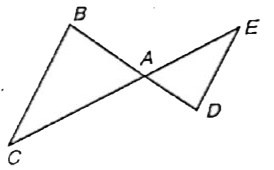A6 cm
B7.5 cm
C9 cm
D10 cm
Question 2 - Select One
$□ A B C D$ is a parallelogram, $A B = 14 c m, B C = 18 c m and A C = 16 c m$ . Find the length of the other diagonal:
A24 cm
B28 cm
C36 cm
D32 cm
Question 3 - Select One
For a triangle ABC,D and E are two points on AB and AC such that $A D = \frac{1}{4} A B, A E = \frac{1}{4} A C$ . If $B C = 12 c m$ , then $D E$ is
Aa) 5 cm
Bb) 4 cm
Cc) 3 cm
Dd) 6 cm

PEARSON IIT JEE FOUNDATION-GEOMETRY-Concept Application Level -3

Home
Ask Doubt
Profile