In a ∠PQR, M lies on PR and between P and R such that QR=QM=PM. If ∠MQR=40∘, then find ∠P.
35∘
25∘
45∘
55∘
The correct Answer is:A
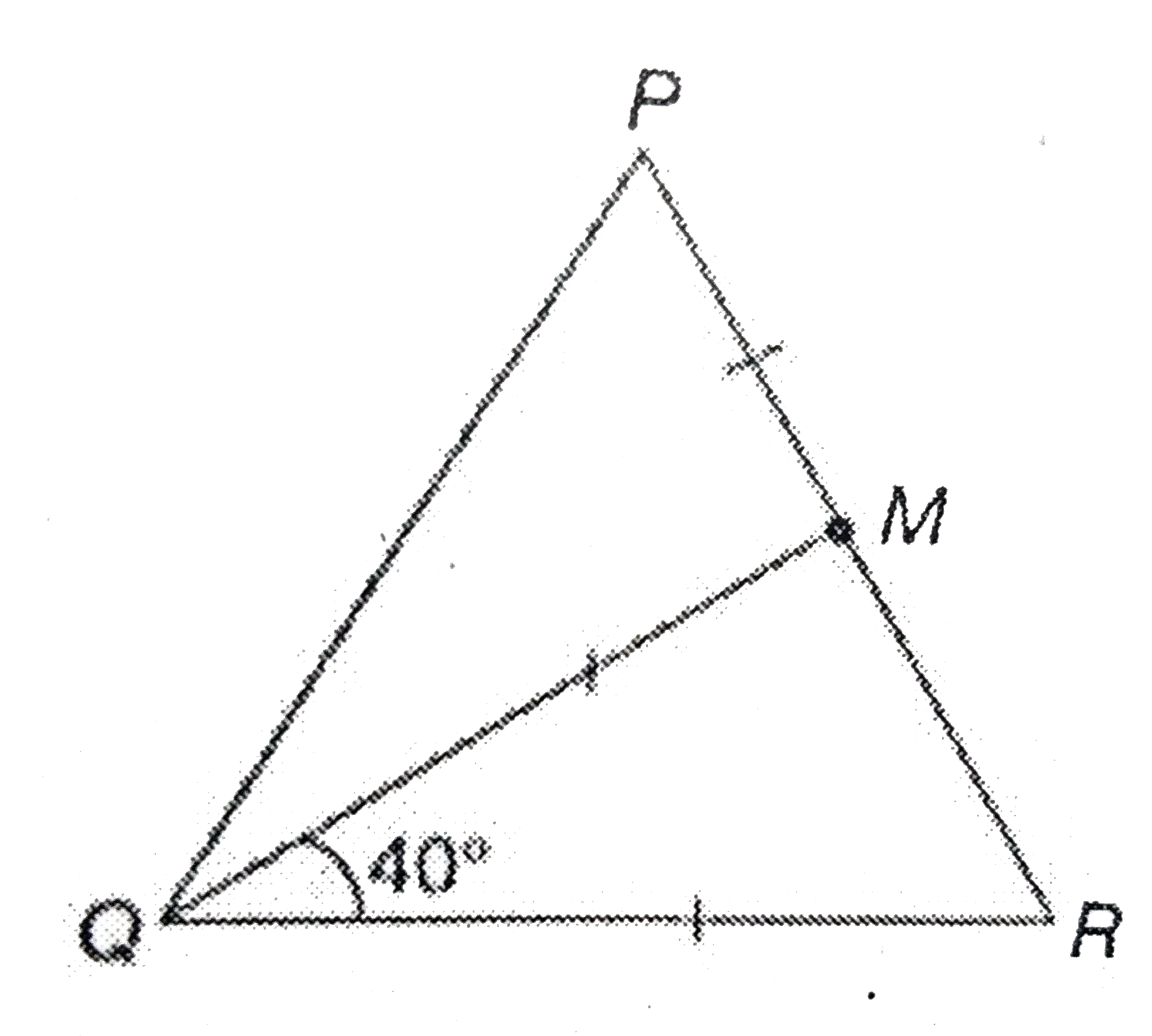
Given QR=QM=PMand∠MQR=40∘
In ΔQMR,QM=QR
∴∠QRM=∠QMR
Now, ∠QRM=∠QMR
=12(180∘−40∘)=70∘
In ΔMPQ,PM=MQ
∠PQM=∠MPQ(1)
But ∠+∠MPQ=∠QMR=70∘(2)
form Eqs. (1) and (2)
∠MPQ=12(70∘)=35∘
Topper's Solved these Questions
Similar Questions
In △PQR, the side QR is extended to S such that RS = PR. If ∠QPS=110∘ and ∠PRQ=70∘, then the value of ∠PQR is:
PQR में, भुजा QR को S तक बढ़ाया जाता है ऐसा कि RS = PR. यदि ∠QPS=110∘ और ∠PRQ=70∘, तो ∠PQR का मान है:
View SolutionIn △PQR, QT is perpendicular to PR and s is a point on QR such that, ∠PSQ=p∘. If ∠TQR=46∘ and ∠SPR=32∘, then the value of p is :
त्रिभुज PQR में, QT, PR पर लंब है और QR पर बिंदु s इस प्रकार पर है कि ∠PSQ=p∘ है| यदि ∠TQR=46∘ और ∠SPR=32∘ है, तो p का मान ज्ञात करें|
View Solution
Knowledge Check
In a triangle PQR, S and T lie on PQ and PR, respectively, such that ∠QSR=∠PRQ and ∠QTR=∠PQR. And, PQ = 12, QR = 9, PR = 15. Find the value of PS×PT
AA)50.4BB)52.64CC)49.75DD)none of theseΔPQR is right angled at Q. If ∠R=30∘, then find the value of (cos P -1/3).
A1/6B(2√2−1)√2C−1/√3D(√3−4)/2√3I is the incentre of a triangle PQR and ∠=107∘ then find the value ∠P.
A56°B34°C26°D42°
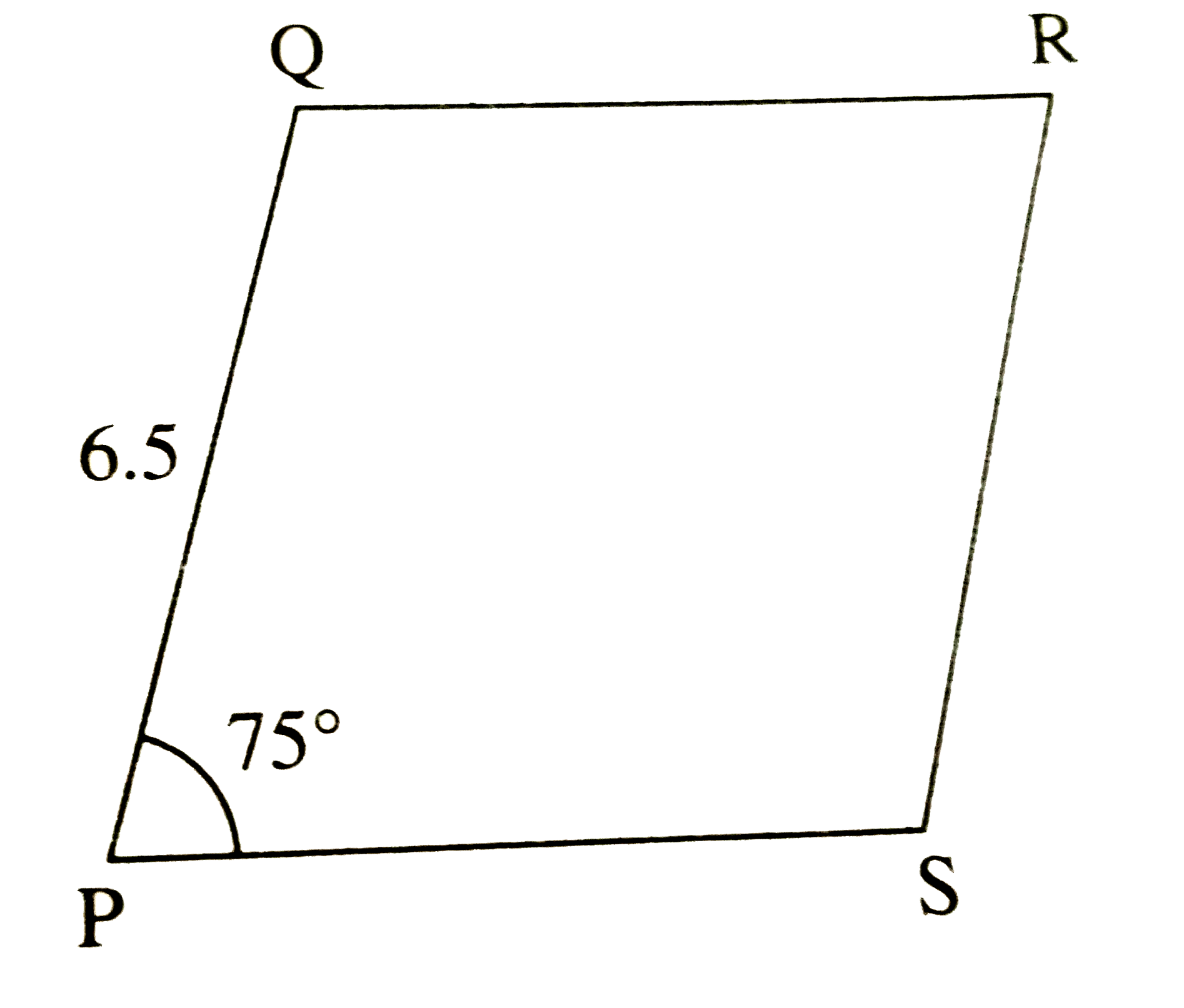
In rhombus PQRS,
PQ =6.5
and ∠QPS=75∘ , then find QR and ∠ PQR .
View SolutionIn a quadrilateral PQRS, the diagonals PR and QS bisect each other. If ∠Q=56∘, determine ∠R.
View SolutionIn △PQR,∠Q≻∠R, PS is the bisector of ∠P and PT⊥QR. If ∠SPT=28∘ and ∠R=23∘ , then the measure of ∠Q is:
त्रिभुज PQR में, ∠Q≻∠R, है तथा PS कोण P का समद्विभाजक है और PT⊥QR है । यदि ∠SPT=28∘ और ∠R=23∘ है, तो कोण Q का मान क्या है?
View SolutionIn a parallelogram PQRS, the bisectors of ∠P and ∠Q meet at O. Find ∠POQ.
View Solution
In the given figure, PQRS is a quadrilateral ,PR and QS intersect at O. If ∠PQR=70∘,∠SPQ=80∘,and∠PRQ=60∘, then find ∠SPR.
View Solution