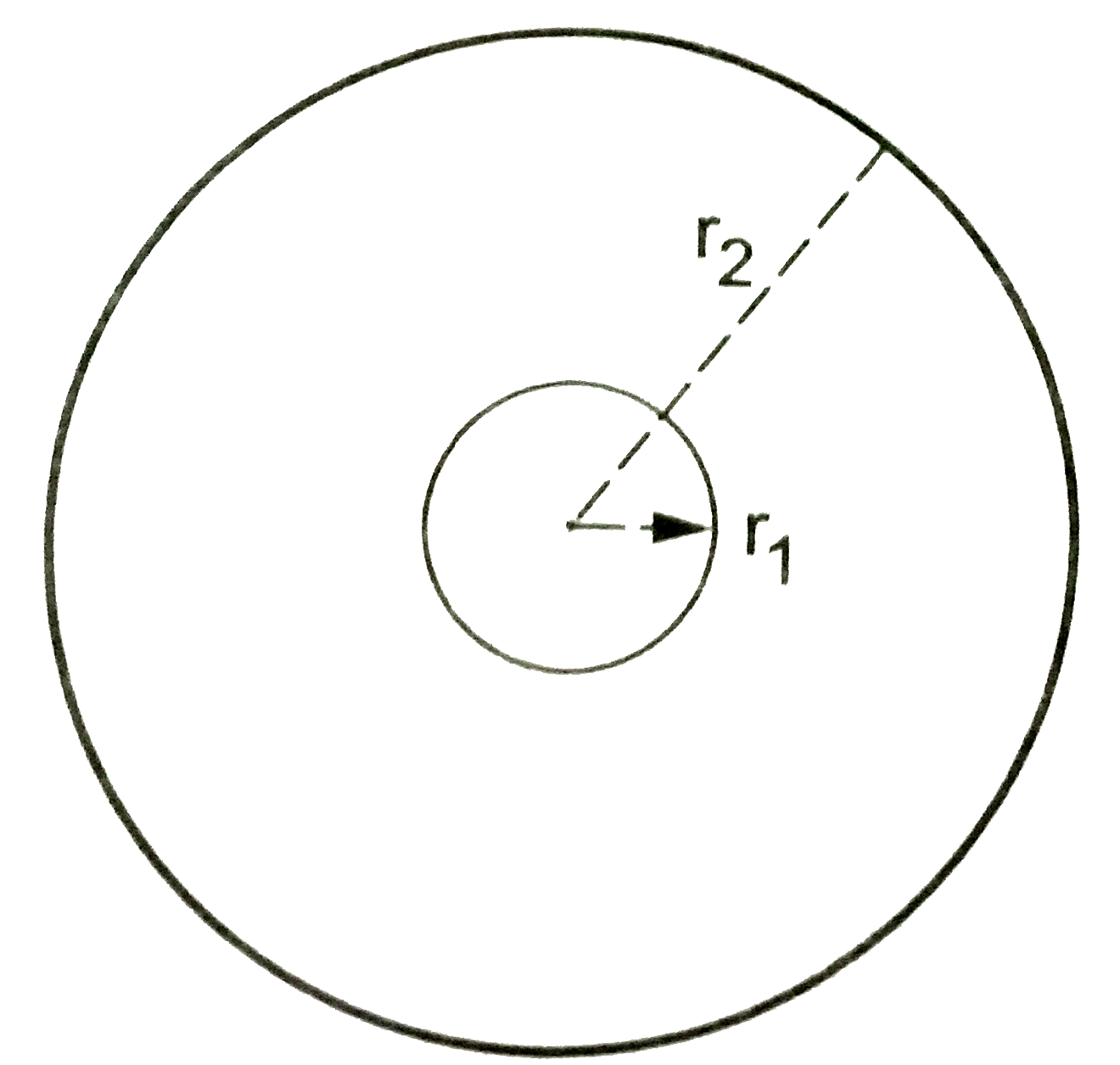
Two concentric circular coils, one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement. Solution in Tamil
Let a current I2 flow through the outer circular coil. The field at the centre of the coil is B2=μ0I22r2. Since r1<<r2,B2 may be considered constant over cross-sectional area of the other coil. Hence, flux linking with it is
ϕ1=πr21B2=μ0πr212r2I2=M12I2
Thus, M12=μ0πr212r2 from equation (3) and (6)
M12=M21=μ0πr212r2
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - SOLUTIONS TO EXERCISES FROM NCERT TEXT
14 Videos
- ELECTROMAGNETIC INDUCTION BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - PRACTICE PROBLEMS FOR SELF ASSESSMENT
3 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Competitive Exam Corner
20 Videos
- ELECTROMAGNETIC WAVES BOOK - NEW JOYTHI PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - COMPETITIVE EXAM CORNER
16 Videos
Similar Questions
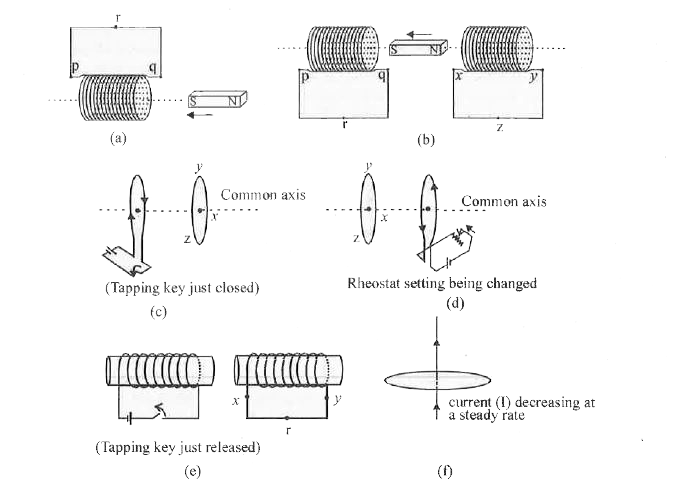
Predict the direction of induced current in the situations described by the following figures (a) to (f).
View SolutionA long solenoid with 15 turns per cm has a small loop area 2.0cm2 placed inside the solenoid normal to its axis. If the current carried by the solenoid changed steadiy from 2.0A to 4.0A in 0.1 s, what is the induced emf in the loop while the current is changing?
View SolutionTwo charged spherical conductors of radii R1andR2 are connected by a wire. Then the ratio of surface charge densitites of the spheres σ1/σ2 is
View SolutionIf the total electromagnetic energy falling on a surface is U, then the total momentum delivered (for complete absorption) is
View SolutionIn co-axial concentric coils of radius r_(1) and r_(2) such that r_(1) lt lt r_(2) Fig. find direction of induced current when K is (i) pressed (ii) released.
View SolutionTwo circular coils, one of smaller radius r1 and the other of very large radius r2 are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo coils are placed closed to each other. The mutual inductance between them assuming R_(2)lt ltR_(1)
View SolutionTwo concentric circular coil,s one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View Solution(a) Explain the meaning of the term mutual inductance. Consider two concentric circular coils, one radius r_(1) and the other of radius r_(2)(r_(1) lt r_(2)) placed coaxially with centres coinciding with each other. Obtain the expression for the mutual inductance of the arrangement. (b) A rectangular coil of area A , having number of turns N is rotated at 'f' revoluation per second in a uniform magnetic field B , the field being perpendicular to the coil. Prove that maximum emf induced in the coil is 2pifNBA .
View Solutionदो संकेद्रीय (concentric ) वृत्ताकार कुंडलियाँ समाक्षीय रुप से (coaxially) व्यवस्थित हैं । इनकी त्रिज्याएँ r1 तथा (r2 हैं तथा r1≤≤r2 हैं । इस अवस्था का अन्योन्य प्रेरकत्व (mutual inductance) ज्ञात करें ।
View Solutionदो वृत्ताकार कुण्डलियों जिनकी त्रिज्याएँ R1 एवं R2(R2>>R1) है, एक समतल पर रखी हुई है। छोटी कुण्डली बड़ी कुण्डली के केन्द्र पर स्थित है। यदि बड़ी कुण्डली में धारा प्रवाहित की जाए तो छोटी कुण्डली का बड़ी कुण्डली के सापेक्ष अन्योन्य प्रेरण गुणांक निर्भर करेगा :
View Solutionदो संकेंद्रित वृताकार कुंडलिया एक कम त्रिजिया r1 की तथा दूसरी अधिक त्रिजिया r2 की ऐसे की r1>>r2 समाक्षी रखी है था दोनों के केंद्र संपत्ति है इस व्यवस्था के लिए अन्यों परिकत्व ज्ञात कीजिये
View SolutionTwo circular coils, one of smaller radius r_(1) and the other of very large radius r_(2) are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo circular coils, one of smaller radius r1 and the other of very large radius r2 are placed co-axially with centres coinciding. Obtain the mutal inductance of the arrangement.
View SolutionThe conducting circular loops of radii R_(1) and R_(2) are placed in the same plane with their centres coinciding. If R_(1) gt gt R_(2) , the mutual inductance M between them will be directly proportional to
View SolutionThe conducting circular loops of radii R_(1) and R_(2) are placed in the same plane with their centres coinciding. If R_(1) gt gt R_(2) , the mutual inductance M between them will be directly proportional to
View Solution
