An ideal gas passes from one equllibrium state (P1,V1,T1,N) to anoter equilibrium state (2P1,3V1,T2,N) Then Solution in Tamil
T1=T2
T1=T26
T1=6T2
T1=3T2
The correct Answer is:B
From ideal gas equation, PV=NkT
One equilibrium state (P1,V1,T1,N)
Another equilibrium state (P2,V2,T2,N)
P2=2P1andV2=3V1
P1V1P2V2=T1T2
P1V1P2V2=T1T2
P1V1(2P1)(3V1)=T1T2⇒16=T1T2
∴ T1=T26
Topper's Solved these Questions
- HEAT AND THERMODYNAMICSBOOK - FULL MARKSCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - TEXTUAL EVALUATION SOLVED (II. Short Answer Questions)
48 Videos
- HEAT AND THERMODYNAMICSBOOK - FULL MARKSCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - TEXTUAL EVALUATION SOLVED (III. Long Answer Questions)
24 Videos
- HEAT AND THERMODYNAMICSBOOK - FULL MARKSCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (Numerical Problems)
16 Videos
- GRAVITATIONBOOK - FULL MARKSCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL QUESTIONS SOLVED (Numerical Problems)
18 Videos
- KINEMATICSBOOK - FULL MARKSCHAPTER - KINEMATICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (LONG ANSWER QUESTIONS)
10 Videos
Similar Questions
An object contains more heat -Is it a right statement ? If not why ?
View SolutionExplain the meaning of heat and work with suitable examples.
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is:
View SolutionA geo-stationary satellite is orbiting the Earth of a height of 6R above the surface of Earth R being the radius of the Earth calculate the time period of another satellite at a height of 2.5R from the surface of Earth.
View SolutionExplain the types of motion with example
View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
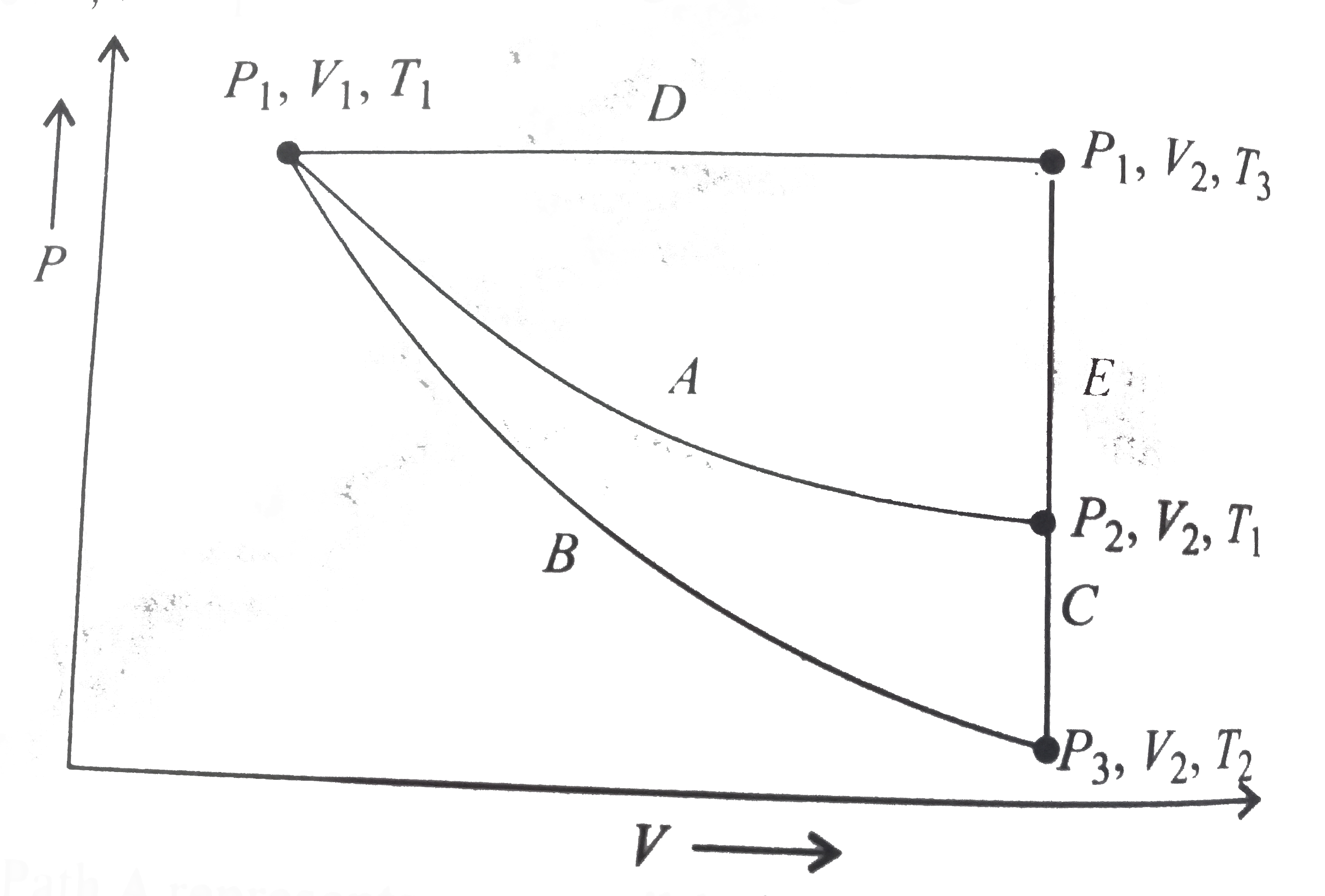
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path A?
View SolutionIn an adiabatic process, the state of a gas is changed from P_(1),V_(1),T_(1), "to" P_(2),V_(2),T_(2). Which of the following relation is correct
View SolutionAn ideal gasa undergoes through folowing cyclic procees. 1-2 : Reversible adialbatic comparession from P_(1)V_(1)T_(1)"to"P_(2)V_(2)T_(2) 2-3 : Reversible isochoric heating from P_(2)V_(2)T_(2)" to " P_(3)V_(3)T_(3) 3-4 Reversible adialbatic expansion from P_(3)V_(3)T_(3)" to " P_(4)V_(4)T_(4) 4-1 Reversible isochoric cooling from P_(4)V_(4)T_(4)" to " P_(1)V_(1)T_(1) Eefficiencyof the cycle is :
View SolutionOne mole of hydrogen, assumed to be ideal, is adiabatically expanded from its initial state (P1,V1,T1) to the final state (P2,V2,T2). The decrease in the internal energy of the gas during this process will be given by
View SolutionAn ideal gas passes from one equllibrium state (P1,V1,T1,N) to anoter equilibrium state (2P1,3V1,T2,N) Then
View SolutionIn an adiabatic process the state of a gas is changed from P_(1) , V_(1) ,T_(1) , to P_(2) ,V_(2) ,T_(2). Which of the following relation is correct ?
View Solutionयदि रुद्धोष्म परिवर्तन में गैस की अवस्था P1,V1,T1 सेP2,V2,T2 तक परिवर्तित हो जाती है , तो निम्न में से कौन - सा संबंधसत्य है -
View SolutionThe initial pressure, temperature & volume of 1 mol of a gas are P1,T1andV1 respectively. The state of the gas is changed by the following two ways. Will the internal energy change be the same in both cases?
(1) P1V1T2Reversible−−−−−→ProcessP2V2T2
(2) P1V1T1Irreversible−−−−−−→processP2V2T2.
View SolutionAn ideal gas expands from state (P_(1), V_(1)) to state (P_(2), V_(2)) , where P_(2) = 2P_(1) and V_(2) = 2V_(1) . The path of the gas is expressed by the following relation, P = P_(1) [1 + ((V - V_(1))/(V_(1)))^(2)]
View SolutionEstablish gas equation, (P_(1)V_(1))/(T_(1))=(P_(2)V_(2))/(T_(2)) .
View Solution
