One mole of hydrogen, assumed to be ideal, is adiabatically expanded from its initial state (P1,V1,T1) to the final state (P2,V2,T2). The decrease in the internal energy of the gas during this process will be given by
CV(T1−T2)
CP(T1−T2)
CP+CV2(T1−T2)
(CP−CV)(T1−T2)
The correct Answer is:A
Step by step video, text & image solution for One mole of hydrogen, assumed to be ideal, is adiabatically expanded from its initial state (P_(1), V_(1), T_(1)) to the final state (P_(2), V_(2), T_(2)). The decrease in the internal energy of the gas during this process will be given by by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Dscillations) (Kinematics of SHM)20videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Energy & spring pendulum)16videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Thermal Physics) (Kinetic theory of gasess)13videos
RACE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
Similar Questions
A gas with cpcV=γ goes from an intial state (p1,V1,T1) to a final state (p2,V2,T2) through an adiabatic process. The work done by the gas is
View SolutionA certain mass of an ideal gas at pressure P1 is adiabatically expanded from an initial volume V1 to a final volume V2. The resulting pressure P2 of the gas is given by:
View Solution
Knowledge Check
- Question 1 - Select One
One mole of helium is adiabatically expanded from its initial state (Pi,Vi,Ti) to its final state (Pf,Vf,Tf). The decrease in the internal energy associated with this expansion is equal to
ACV(Ti−Tf)BCP(TiTf)C12(CP+CV)(Ti−Tf)D(Cp+CV)(Ti−Tf) - Question 2 - Select One or More
An ideal gas is expand from (p1,V1,T1) to (p2,V2,T2) under different conditions. The correct statement(s) among the following is (are):
AThe work done by the gas is less when it is expanded reversibly from V1 to V2 under adiabatic conditions as compared to that when expanded reversibly from V1 to V2 under isothermal conditionsBThe change in internal energy of the gas is (i) zero, if it is expanded reversibly with T1=T2, and (ii) positive, if it is expanded reversibly under adiabatic conditions with T1≠T2CIf the expansion is carried out freely, it is simultaneously both isothermal as well as adiabaticDThe work done on the gas is maximum when it is compressed irreversibly from (P2,V2) to (P1,V1) against constant pressure P1 - Question 3 - Select One
An ideal gas is transformed from state A(P1,V1) to the state B(P2,V2) through path AB. In this process the work done by the gas is
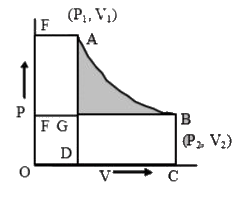 AW = area ABCDABW = area ABEFACW = area ABGADW = area ABCOFA
AW = area ABCDABW = area ABEFACW = area ABGADW = area ABCOFA
The change in the entropy of a 1 mole of an ideal gas which went through an isothermal process form an initial state (P1,V1,T) to the final state (P2,V2<T) is equal to
View SolutionOne mole of a gas in the state A(P1,V1,T1) is subjected to adiabatic expansion to attain state B(P2,V2,T2). The work done by the gas is:
View SolutionAn ideal gas (1 mol, monatomic) is in the intial state P (see Fig.) on an isothermal A at temperature T0. It is brought under a constant volume (2V0) process to Q which lies on an adiabatic B intersecting the isothermal A at (P0,V0,T0). The change in the internal energy of the gas during the process is (in terms of T0)(22/3=1.587)
 View Solution
View SolutionAn ideal gas follows a process described by PV2=C from (P1,V1,T1) to (P2,V2,T2) (C is a constant). Then
View SolutionFor an ideal gas, an illustration of the different paths, A (B+C) and (D+E) from an intial state P1,V1,T1 to a final state P2,V2,T1 as shown in the given figure.
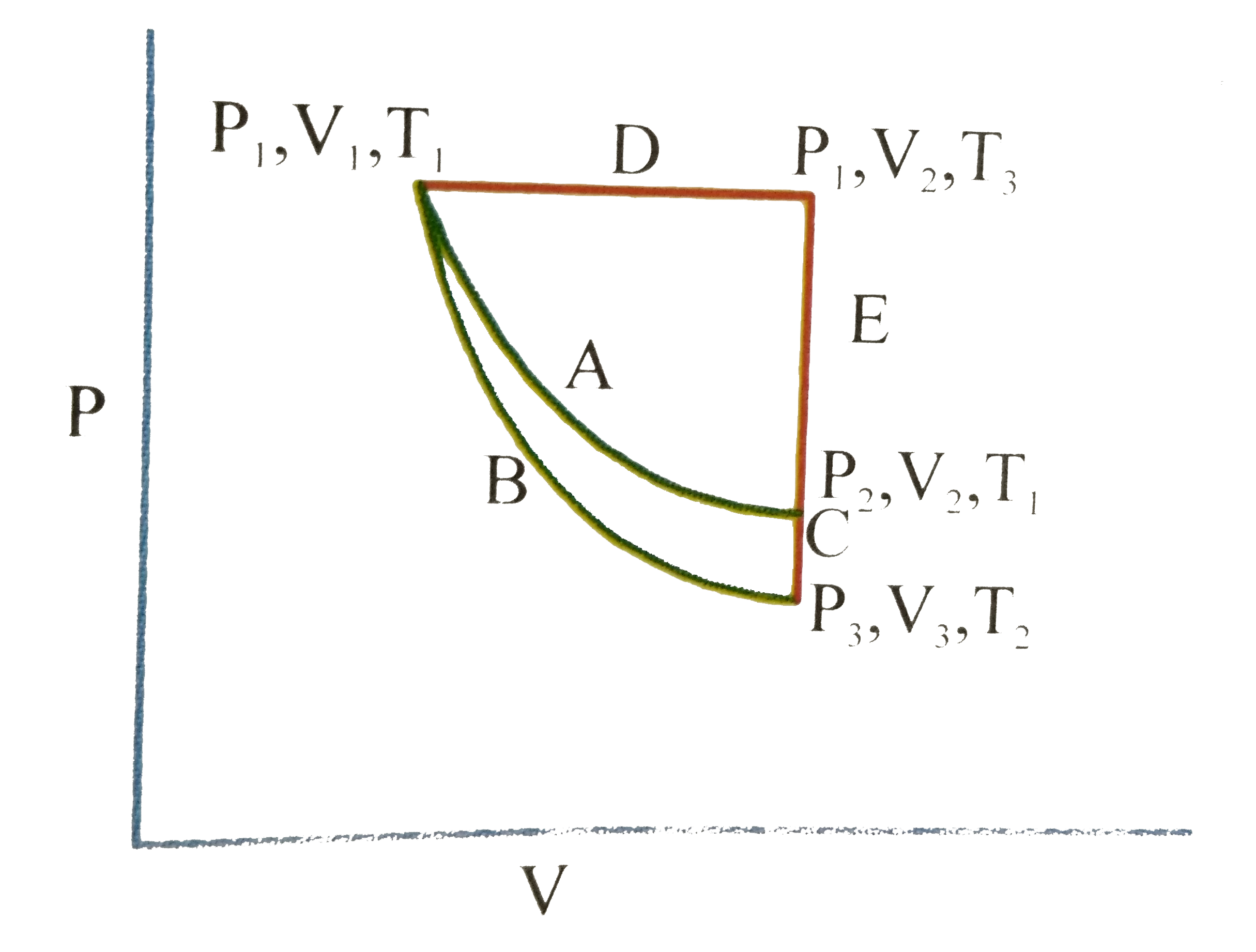
Path (A) represents a reversible isothernal expanison from P1V1 to P2V2. Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating the gas at constant volume (C) from P3,V2,T2 to P2,V2T1 to P1,V2,T3 followed by reversible cooling at a constant volume V2 (E) from P1,V2,T3 to P2,V2,T1
What is ΔS for path (D+E) ?View Solution

