Two air core solenoids have the same lengdth of 80 cm and same cross-sectional area 5cm2. Find the mutual inductance between them if the number of turns in the first coil is 1200 turns and that in the second coil is 400 turns.Solution in Tamil
Step by step video & image solution for Two air core solenoids have the same lengdth of 80 cm and same cross-sectional area 5cm^(2). Find the mutual inductance between them if the number of turns in the first coil is 1200 turns and that in the second coil is 400 turns. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER -14 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -14 (UNSOLVED)EXERCISE - PART-IV
9 Videos
- SAMPLE PAPER -14 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -14 (UNSOLVED)EXERCISE - PART-II
9 Videos
- SAMPLE PAPER -13BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -13EXERCISE - PART - IV
10 Videos
- SAMPLE PAPER -4 (SOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -4 (SOLVED)EXERCISE - PART-IV
10 Videos
Similar Questions
Explain the equivalent resistance of a series and parallel resistor network.
View SolutionCalculate the number of electrons in one coulomb of negative charge.
View SolutionObtain the expression for electric field due to an charged infinite plane sheet .
View SolutionCalculate the electric field due to a dipole on its axial line and equatorial plane.
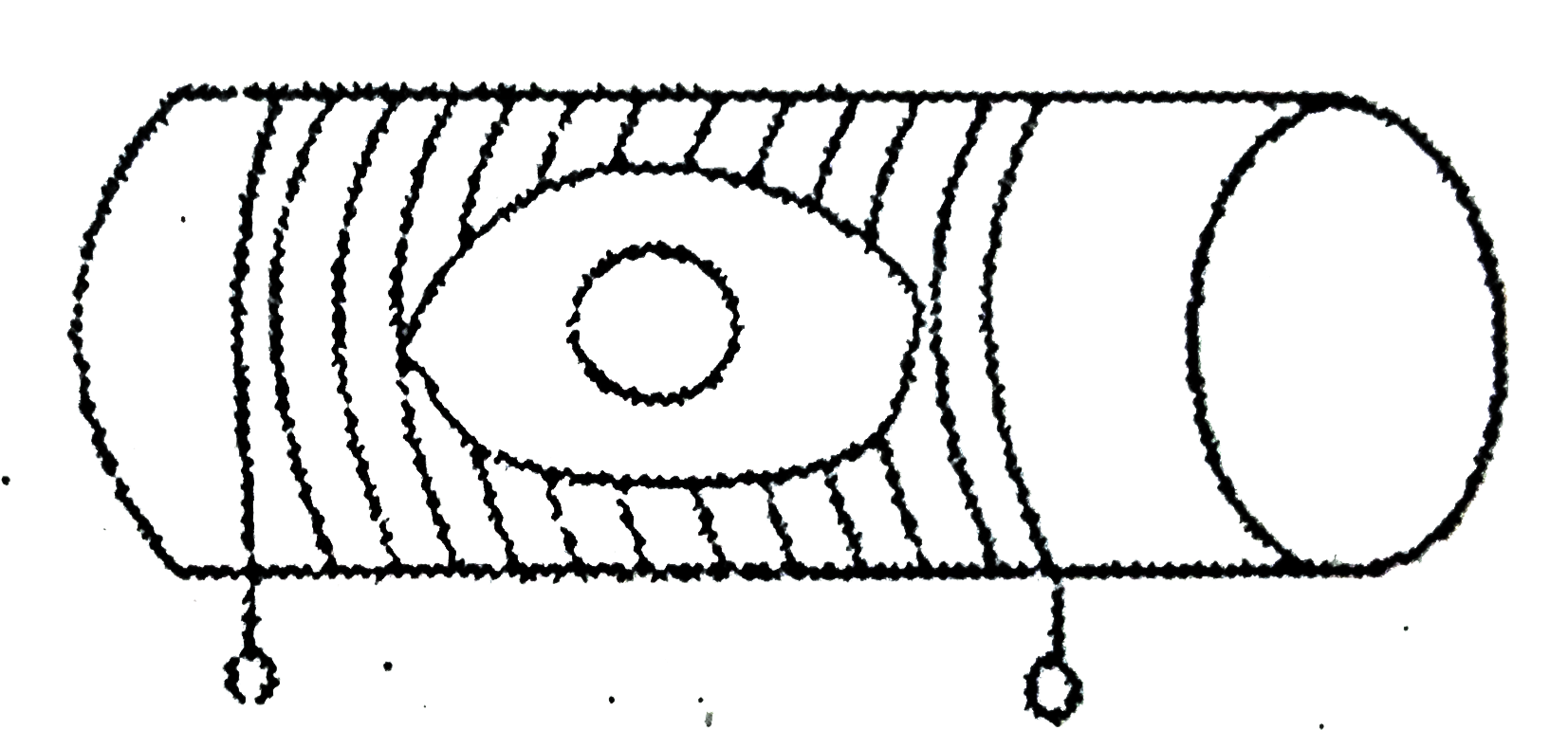
View SolutionA circular coil with a cross-sectional area of 4cm^(2) has 10 turns. It is placed at the center of a long solenoid that has 15 turns/cm and a cross sectional area of 10cm^(2) , shown in the figure. The axis of the coil conicides with the axis of the solenoid. What is their mutual inductance?
View SolutionA solenoidal coil has 50 turns per centimeter along its length and a cross sectional area of 4×10−4m2. 200 turns of another wire is wound round the first solenoid co-axially. The two coils are electrically insulated from each other. Calculate the mutual inductance between the two coils.
View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns/cm. It is placed at the centre of a long solenoid that has 15 turns/cm and a cross-sectional area of 10cm2. The axis of the coil coincides with the axis of the solenoid. What is their mutual inductance ?
View SolutionTwo air core solenoids have the same lengdth of 80 cm and same cross-sectional area 5cm2. Find the mutual inductance between them if the number of turns in the first coil is 1200 turns and that in the second coil is 400 turns.
View SolutionA 200 turn coil of radius 2 cm is placed coil axially within a long solenoid of 3 cm radius. If the turns density of the solenoid is 90 turns per cm, then calculate mutual inductance of the coil.
View SolutionCalculate the self-inductance of an air-cored solenoid 50 cm long and 2 cm radius if the number of turns in the coil of solenoid is 400.
View SolutionThe self-inductance of an air core solenoid of 100 turns iz 1 mH. The self-inductance of another solenoid of 50 turns (with the same length and cross sectional area) with a core having relative permeability 500 is
View SolutionThe self-inductance of an air core solenoid of 100 turns is 1 mH. The self-inductance of another solenoid of 50 turns (with the same length and cross-sectional area) with a core having relative permeability 500 is
View SolutionA 200 turn coil of radius 2 cm is placed coil axially within a long solenoid of 3 cm radius. If the turns density of the solenoid is 90 turns per cm, then calculate mutual inductance of the coil.
View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns/cm. It is placed at the centre of a long solenoid that has 15 turns/cm and a cross-sectional area of 10cm2. The axis of the coil coincides with the axis of the solenoid. What is their mutual inductance ?
View SolutionA solenoidal coil has 50 turns per centimetre along its length and a cross-sectional area of 4xx10^(-4)m^(2) . 200 turns of another wire is wound round the first solenoid co-axially. The two coils are electrically insulated from each other. Calculate the mutual inductance between the two coils.
View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns. It is placed at the center of a long solenoid that has 15 turns/cm and a cross sectional area of 10cm2, shown in the figure. The axis of the coil conicides with the axis of the solenoid. What is their mutual inductance?
View Solution
