Two identical sources of light are separated through a distance d=λ8, where λ is the wavelength of the waves emitted by either source. The phase difference of the sources is π4.Intensity distribution in the radiation field as a function of θ is :
4I0cos2(π)4
4I0cos2(π)8
4I0cos2[π8sinθ]
4I0cos2[π8(sinθ+1)]
The correct Answer is:D
Hered=λ8
The phase difference between the sources
Δϕ=π4
Also x=dsinθ
∴ϕ=2πλ.x=2πλ.λ8sinθ=π4sinθ
∴ Total phase difference,
ϕ=ϕ.+Δϕ.=π4sinθ+π4
or ϕ=π4(sinθ+1)
∴ Required intensity is
I=4I0cos2(ϕ)2
or I=4I0cos2[π8(sinθ+1)]
Topper's Solved these Questions
- WAVE OPTICS BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICS EXERCISE - MCQ LEVEL- II (ASSERTIONS)
2 Videos
- WAVE OPTICS BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICS EXERCISE - MCQ LEVEL- II (PARAGRAPH QUESTIONS)
28 Videos
- WAVE OPTICS BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICS EXERCISE - MCQ LEVEL -1 (PARAGRAPH QUESTIONS )
34 Videos
- WAVE MOTIONBOOK - MODERN PUBLICATIONCHAPTER - WAVE MOTIONEXERCISE - RECENT COMPETITIVE QUESTIONS
18 Videos
- WORK , POWER , ENERGY AND COLLISIONSBOOK - MODERN PUBLICATIONCHAPTER - WORK , POWER , ENERGY AND COLLISIONSEXERCISE - Revision Test
40 Videos
Similar Questions
Two identical sources each of intensity I0 have a separation d=λ/8, where λ is the wavelength of the waves emitted by either source. The phase difference of the sources is π/4 The intensity distribution I(θ) in the radiation field as a function of θ Which specifies the direction from the sources to the distant observation point P is given by
View SolutionTwo identical radiators have a separation of d=λ/4 where λ is the wavelength of the waves emitted by either source. The initial phase difference between the sources is π/4. Then the intensity on the screen at a distant point situated at an angle θ=30∘ from the radiators is (here I0 is intensity at that point due to one radiator alone)
View Solution
Knowledge Check
Two identical sources each of intensity I0 have a separation d=λ/8, where λ is the wavelength of the waves emitted by either source. The phase difference of the sources is π/4 The intensity distribution I(θ) in the radiation field as a function of θ Which specifies the direction from the sources to the distant observation point P is given by
AI(θ)=I0cos2θBI(θ)=I04cos2(πθ8)CI(θ)=4I0cos2[π8(sinθ+1)]DI(θ)=I0sin2θTwo identical radiators have a separation of d=λ/4 where λ is the wavelength of the waves emitted by either source. The initial phase difference between the sources is λ/4. Then the intensity on the screen at a distant point situated at an angle θ=30∘ from the radiators is (here I0 is intensity at that point due to one radiator alone)
A(a) I0B(b) 2I0C(c) 3I0D(d) 4I0S1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
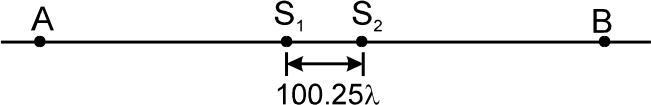 A∞B19C0D9
A∞B19C0D9
The intensities of two light sources are I and 91 .If the phase difference between the waves is pi then resultant intensity will be :-
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is

View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is

View SolutionTwo coherent light sources A and B are at a distance 3λ from each other (λ=wavelength)The distances from A on the X-axis at which the interference is constructive are
View SolutionTwo coherent light sources A and B are at a distance 3λ from each other (λ=wavelength)The distances from A on the X-axis at which the interference is constructive are

View Solution
