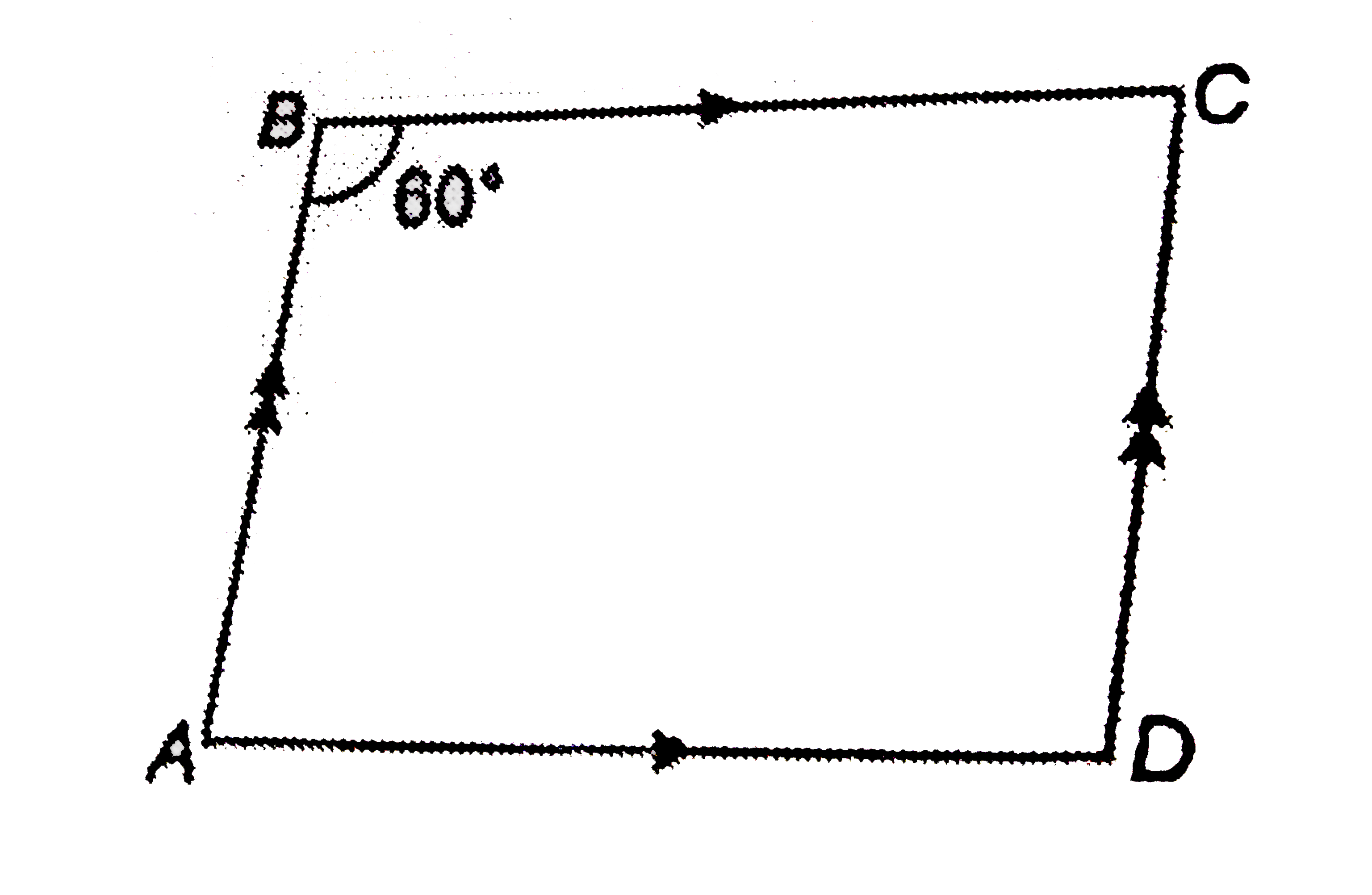In a figure AE = BC and AE||BC and length of AB, CD and ED are same then if ∠A=102∘,∠BCD = ?
A
138∘
B
162∘
C
88∘
D
none of these
Video Solution
Text Solution
The correct Answer is:B
|
Answer
Step by step video, text & image solution for In a figure AE = BC and AE||BC and length of AB, CD and ED are same then if angleA=102^(@), angleBCD = ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.410videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.560videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2100videos
GEOMETRY
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 240videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions18videos
HCF AND LCM
Similar Questions
Knowledge Check
- Question 1 - Select One
In a figure AE = BC and AE||BC and length of AB, CD and ED are same then if ∠A=102∘,∠BCD = ?
 A138∘B162∘C88∘Dnone of these
A138∘B162∘C88∘Dnone of these - Question 2 - Select One
In the figure , l||m ||n and AB||CD , then ∠BCD is -
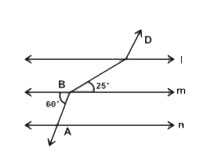 A120∘B145∘C85∘D60∘
A120∘B145∘C85∘D60∘ - Question 3 - Select One
In the ajoining figure AE∣∣CD and BC∣∣ED, then find y:
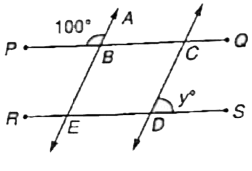 A60∘B80∘C90∘D75∘
A60∘B80∘C90∘D75∘
Two triangles ABC and DBC are on the same base BC and on the same side of BC in which ∠A=∠D=90∘. If CAandBD meet each other at E, show that AE.EC=BE.ED.
View SolutionIn quadrilateral ABCD, AB ||CD and AD= BC, prove that ∠A=∠B.
View SolutionIn the following figure, AD=DB and DE∣∣BC. Then AE=EC (True/ False)
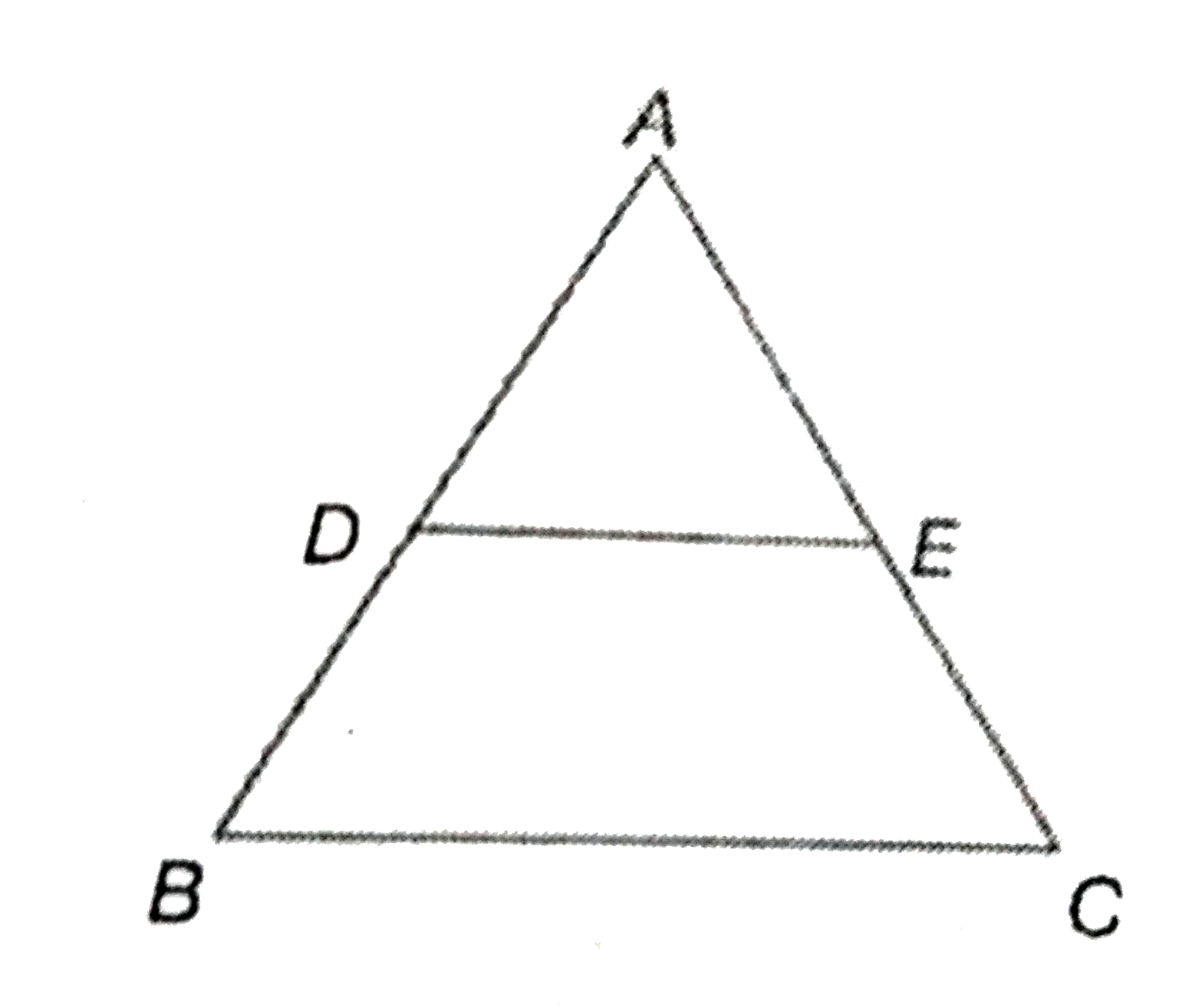 View Solution
View SolutionIn the adjoining figure AE||CD and BC||ED, then find Y.
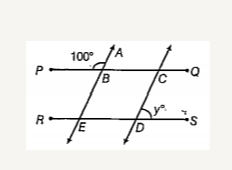 View Solution
View SolutionIn Figure, if AB=AC and BE=CD, prove that AD=AE.
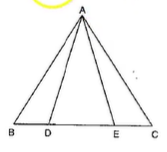 View Solution
View Solution