when two displacements represented by y1=asin(ωt) and y2=bcos(ωt) are superimposed the motion is
Not a simple harmonic
Simple harmonic with amplitude ab
Simple harmonic with amplitude √a2+b2
Simple harmonic with amplitude (a)+(b)2
The correct Answer is:C
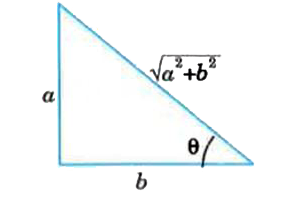
y=y1+y2
=asinωt+bcosωt
=√a2+b2(a√(a2+b2)sinωt+b√a(2)+b2cosωt)
=√a2+b2[cosθsinωt+sinθcosωt]
=√a2+b2sin(ωt+θ)
y=Asin(ωt+θ)
It executes S.H.M. with amplitude √a2+b2
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition file (Multiple choice question) JEE (Main) & Other State Boards for Engineering Entrance25videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (JEE (Advanced) For IIT Entrance)7videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Multiple choice questions)20videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST16videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
Similar Questions
Knowledge Check
- Question 1 - Select One
When two displacement represented by y1=asin(ωt) and y2=bcos(ωt) are superimposed, the motion is
Anot a simple harmonicBsimple harmonic with amplitude abCsimple harmonic with amplitude √a2+b2Dsimple harmonic with amplitude (a2+b2)2 - Question 2 - Select One
Two wave are represented by equation y1=asinωt and y2=acosωt the first wave :-
Aleads the second by πBlags the second by πCleads the second by π2Dlags the second by π2 - Question 3 - Select One
Two waves represented by y1=asinωtandy2=asin(ωt+ϕ)with ϕ=π2
are superposed at any point at a particular instant. The resultant amplitude isAaB4aC√2aDzero
If two waves represented by y1=4sinωt and y2=3sin(ωt+π3)interfere at a point, the amplitude of the resulting wave will be about
View SolutionTwo waves are represented by y1=asin(ωt+π6)andy2=acosωt. What will be their resultant amplitude
View SolutionThe displacements of two intering lightwaves are y1=4sinωt and y2=3cos(ωt). The amplitude of the resultant wave is (y1 and y2 are in CGS system)
View SolutionTwo light waves are represented by y1=asinωtandy2=asin(ωt+δ). The phase of the resultant wave is
View SolutionTwo simple harmonic motions are represented by y1=10sinωt and y2=15cosωt. The phase difference between them is
View Solution

