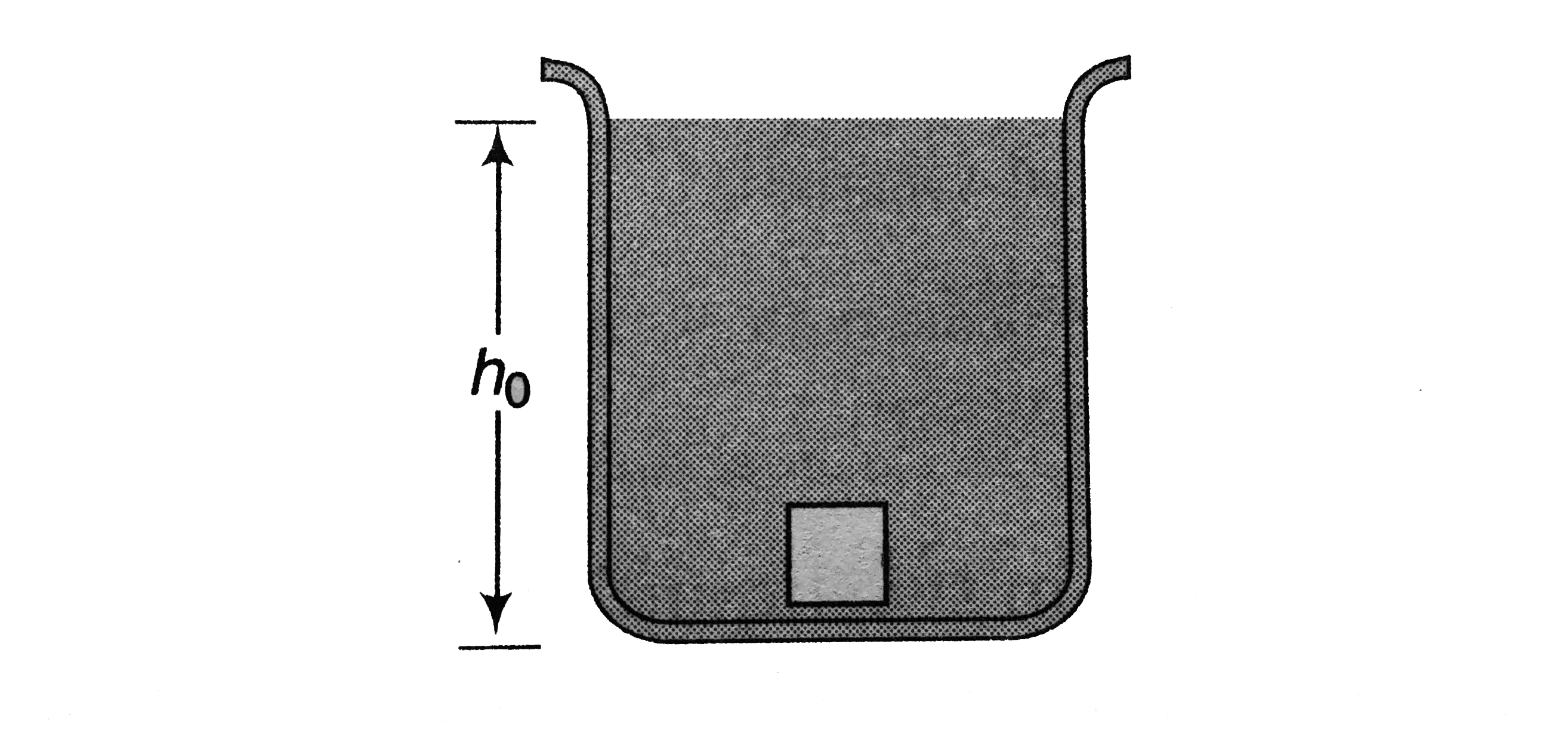
The shown container contains a liquid of variable density which varies as d=d0(4−3yh0)kg/m3 , where h0andd0 are positive constants and y is
measured from bottom of the container. A solid block whose density is 5d0/2 and mass m is released in the container as shown in the figure. show that the block will execute SHM and find its time period.

a=−6g5h0xT=2π√5h06g
Topper's Solved these Questions
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - ASSIGNMENT SECTION-II MCQ (SINGLE CORRECT)
47 Videos
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - MCQ (MULTIPLE CORRECT)
18 Videos
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - NUMERICAL BASED QUESTIONS DECIMAL TYPE
11 Videos
- OPTICSBOOK - FIITJEECHAPTER - OPTICSEXERCISE - NUMERICAL BASED QUESTIONS
2 Videos
- PHYSICS PART2BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type
6 Videos
Similar Questions
Figure shown a container having liquid of variable density. The density of liquid veriesas ρ=ρ0(4−3hh0). Here, h0 and ρ0 are constants and h is measured from bottom of the container. A solid block of small dimensions whose density is 52ρ0 and mass m is released from bottom of the tank. Prove that the block will execute simple harmonic motion. Find the frequency of oscillation.
 .
.
View SolutionFigure shown a container having liquid of variable density. The density of liquid veriesas ρ=ρ0(4−3hh0). Here, h0 and ρ0 are constants and h is measured from bottom of the container. A solid block of small dimensions whose density is 52ρ0 and mass m is released from bottom of the tank. Prove that the block will execute simple harmonic motion. Find the frequency of oscillation.
 .
.
View Solution
Knowledge Check
The equation of SHM of a particle is d2ydt2+ky=0, where k is a positive constant. The time period of motion is
A2π√3B2πkCk2D√k2πA body having volume V and density ρ is attached to the bottom of a container as shown. Density of the liquid is d(>ρ). Container has a constant upward acceleration a. Tension in the string is
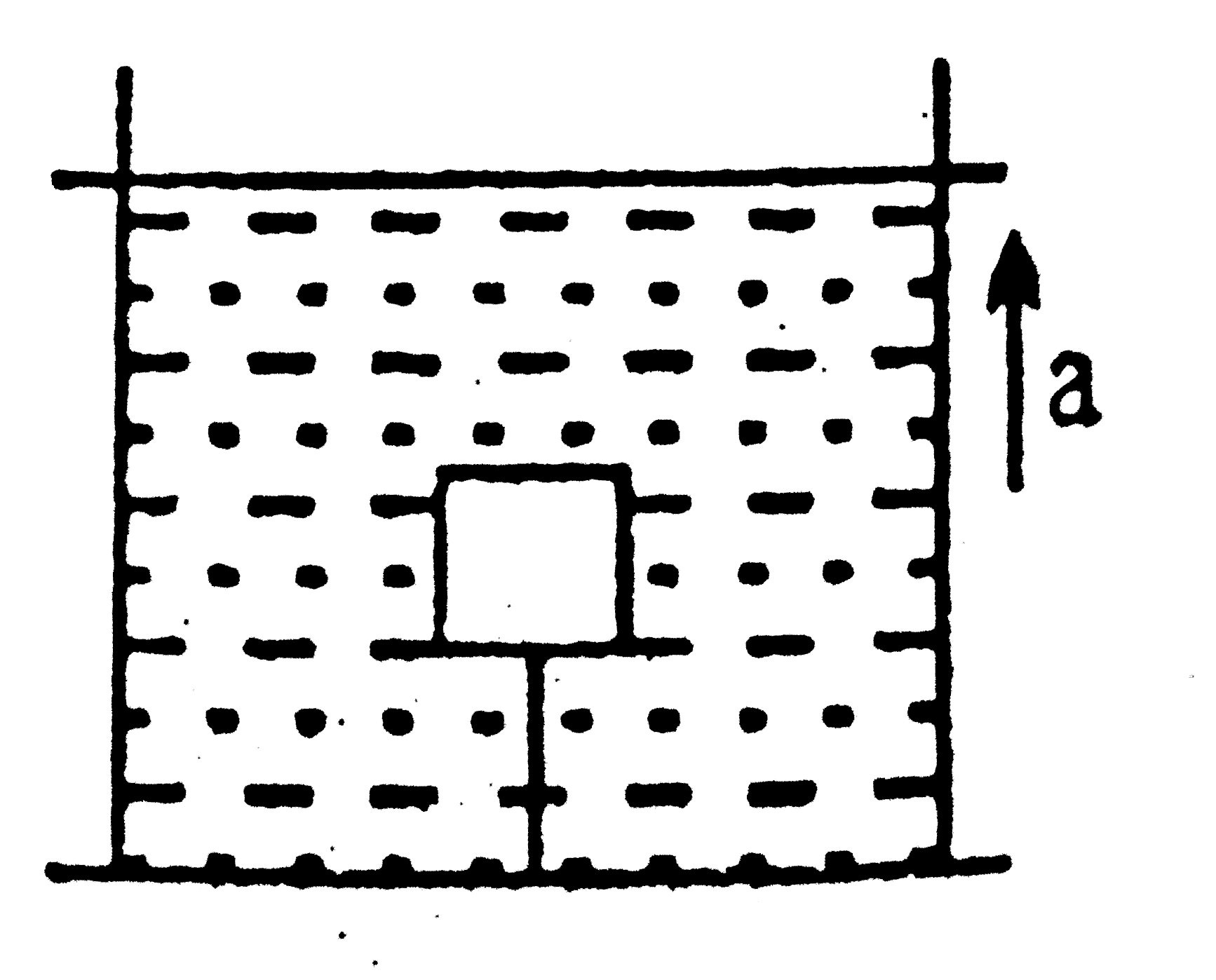 AV[Dg−ρ(g+a)]BV(g+a)(d−ρ)CV(d−ρ)gDnone
AV[Dg−ρ(g+a)]BV(g+a)(d−ρ)CV(d−ρ)gDnone
A vertical U tube contains a liquid of density ρ, length of the liquid in the tube is 2H. The liquid is displaced slightly and released. Show that the liquid will execute SHM. Also find its time period. Take liquid to be ideal.
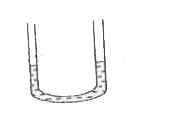
View SolutionA vertical U tube contains a liquid of density ρ, length of the liquid in the tube is 2H. The liquid is displaced slightly and released. Show that the liquid will execute SHM. Also find its time period. Take liquid to be ideal.

View SolutionA container A is having some fluid of density θ0. If is connected with a U tbe manometer shown in figure. Find the pressure of the container in both the cases given in figure.
View SolutionA container A is having some fluid of density θ0. If is connected with a U tube manometer shown in figure. Find the pressure of the container in both the cases given in figure.
View SolutionConsider a situation shown in the figure. Show that if the blocks are displaced slightly in opposite directions and released, they will execute S.H.M. calculate the time period.

View SolutionThe equation of SHM of a particle is d2ydt2+ky=0, where k is a positive constant. The time period of motion is
View Solution
