A block A of mass m is lying on a rough horizontal surface. Another block B of mass 2m having velocity 10 m/s just before collision, makes head on collision with stationary block A. The coefficient of restitution between blocks is 0.5, and the coefficient of friction between blocks and ground is 0.3. Find the distance between the blocks when they stop.
The correct Answer is:12.5 m
To solve the problem step by step, we will follow the principles of conservation of momentum and the coefficient of restitution, and then calculate the stopping distance due to friction.
Step 1: Understand the given information
- Mass of block A (m₁) = m
- Mass of block B (m₂) = 2m
- Initial velocity of block A (u₁) = 0 m/s (stationary)
- Initial velocity of block B (u₂) = 10 m/s
- Coefficient of restitution (e) = 0.5
- Coefficient of friction (μ) = 0.3
- Acceleration due to gravity (g) = 10 m/s²
Step 2: Apply conservation of momentum
The total momentum before the collision must equal the total momentum after the collision.
m₁u₁+m₂u₂=m₁v₁+m₂v₂
Substituting the values we have:
m(0)+2m(10)=mv₁+2mv₂
This simplifies to:
20m=mv₁+2mv₂(1)
Step 3: Apply the coefficient of restitution
The coefficient of restitution relates the relative velocities of separation and approach:
e=v₂−v₁u₁−u₂
Substituting the known values:
0.5=v₂−v₁0−10
This simplifies to:
0.5=v₂−v₁−10
Rearranging gives:
v₂−v₁=−5(2)
Step 4: Solve the equations (1) and (2)
From equation (2), we can express v₂ in terms of v₁:
v₂=v₁−5
Substituting this into equation (1):
20m=mv₁+2m(v₁−5)
Expanding this gives:
20m=mv₁+2mv₁−10m
Combining like terms:
20m=3mv₁−10m
Adding 10m to both sides:
30m=3mv₁
Dividing both sides by 3m:
v₁=10 m/s
Now substituting v₁ back into equation (2):
v₂=10−5=5 m/s
Step 5: Calculate the acceleration due to friction
The frictional force acting on each block is given by:
Ff=μm₁g=0.3⋅m⋅10=3m
The acceleration a due to friction for each block is:
a=Ffm=3mm=3 m/s²
Step 6: Calculate the distance each block travels before stopping
Using the equation of motion:
v2=u2+2as
For block A (initial velocity u₁=10 m/s):
0=(10)2−2(3)s₁
This simplifies to:
100=6s₁⟹s₁=1006≈16.67 m
For block B (initial velocity u₂=5 m/s):
0=(5)2−2(3)s₂
This simplifies to:
25=6s₂⟹s₂=256≈4.17 m
Step 7: Calculate the distance between the blocks when they stop
The distance between the two blocks when they stop is:
d=s₁−s₂=16.67−4.17≈12.5 m
Final Answer
The distance between the blocks when they stop is approximately 12.5 meters.
---
- Mass of block A (m₁) = m
- Mass of block B (m₂) = 2m
- Initial velocity of block A (u₁) = 0 m/s (stationary)
- Initial velocity of block B (u₂) = 10 m/s
- Coefficient of restitution (e) = 0.5
- Coefficient of friction (μ) = 0.3
- Acceleration due to gravity (g) = 10 m/s²
The total momentum before the collision must equal the total momentum after the collision.
The coefficient of restitution relates the relative velocities of separation and approach:
From equation (2), we can express v₂ in terms of v₁:
The frictional force acting on each block is given by:
Using the equation of motion:
The distance between the two blocks when they stop is:
The distance between the blocks when they stop is approximately 12.5 meters.
Topper's Solved these Questions
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I
45 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I (ASSERTION REASONING TYPE)
2 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) Level - I (Numerical)
8 Videos
- AC CIRCUITSBOOK - FIITJEECHAPTER - AC CIRCUITSEXERCISE - ASSERTION REASONING TYPE
1 Video
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Comprehension -4
3 Videos
Similar Questions
Find the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
View SolutionThe block of mass M and are arrenged as the situation in fig is shown.The coefficient of friction between two block is μand that between the bigger block and the ground is μ find the acceleration of the block
View Solution
Knowledge Check
A block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
A12B√3C1√3DNoneA block A of mass 2 kg rests on a horizontal surface. Another block B of mass 1 kg moving at a speed of m/s when at a distance of 16 cm from A. collides elastically with A. The coefficeint of friction between the horizontal surface and earth of the blocks is 0.2. Then (g=10m/s2)
Aafter collision block B reboundsBafter collision block B comes to restCfinal separation between the blocks is 30 cmDfinal separation between the blocks is 50 cmA long block A of mass M is at rest on a smooth horizontal surface.A small block B of mass M/2 is placed on A at one end and projected along A with some veklocity v.The coefficient of friction between the block is μ then , the accelerations of blocks A and B before reaching a common velocity will be respectively
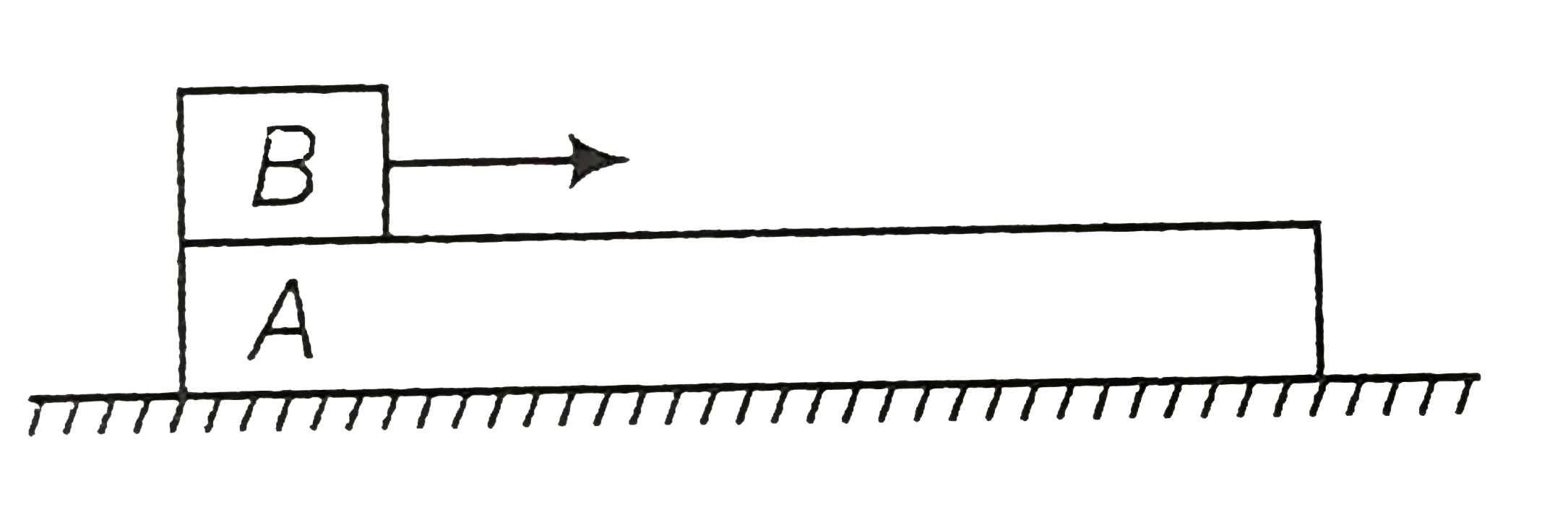 Aμg2(towards right),μg2(towards left)Bμg2(towards right),(μg)(towrards left)Cμg2(towards right),(μg)(towrards left)D(μg)(towards right),μg2(towrards left)
Aμg2(towards right),μg2(towards left)Bμg2(towards right),(μg)(towrards left)Cμg2(towards right),(μg)(towrards left)D(μg)(towards right),μg2(towrards left)
A block of mas m moving at a velocity υ collides head on with another block of mass 2m at rest. If the coefficient of restitution is 1/2, find the velocities of te blocks after the collision.
View SolutionBlock A has a mass 3kg and is sliding on a rough horizontal surface with a velocity uA=2m/s when it makes a direct collision with block B, which has a mass of 2kg and is originally at rest. The collision is perfectly elastic. Determine the velocity of each block just after collision and the distance between the blocks when they stop sliding. The coefficient of kinetic friction between the blocks and the plane is μk=0.3 ( Take g=10m/s2)
View SolutionA block of mass m lying on a rough horizontal plance is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The block will remain in equilibrium, if the coefficient of friction between it and the surface is
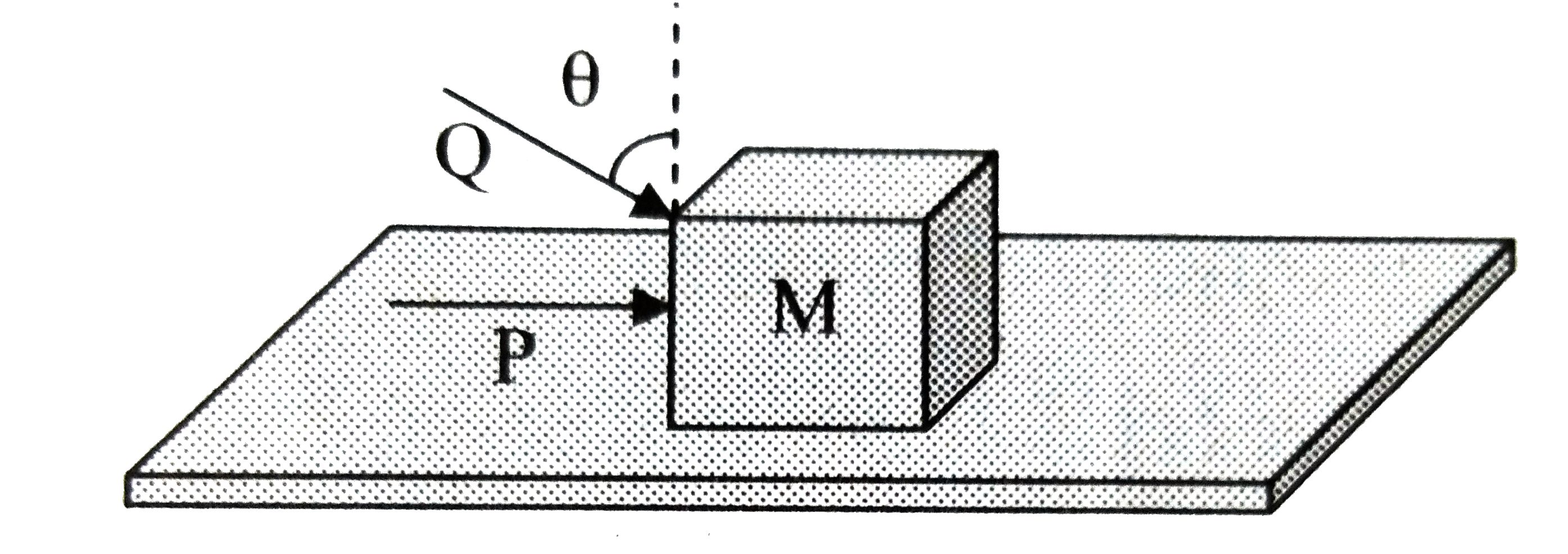
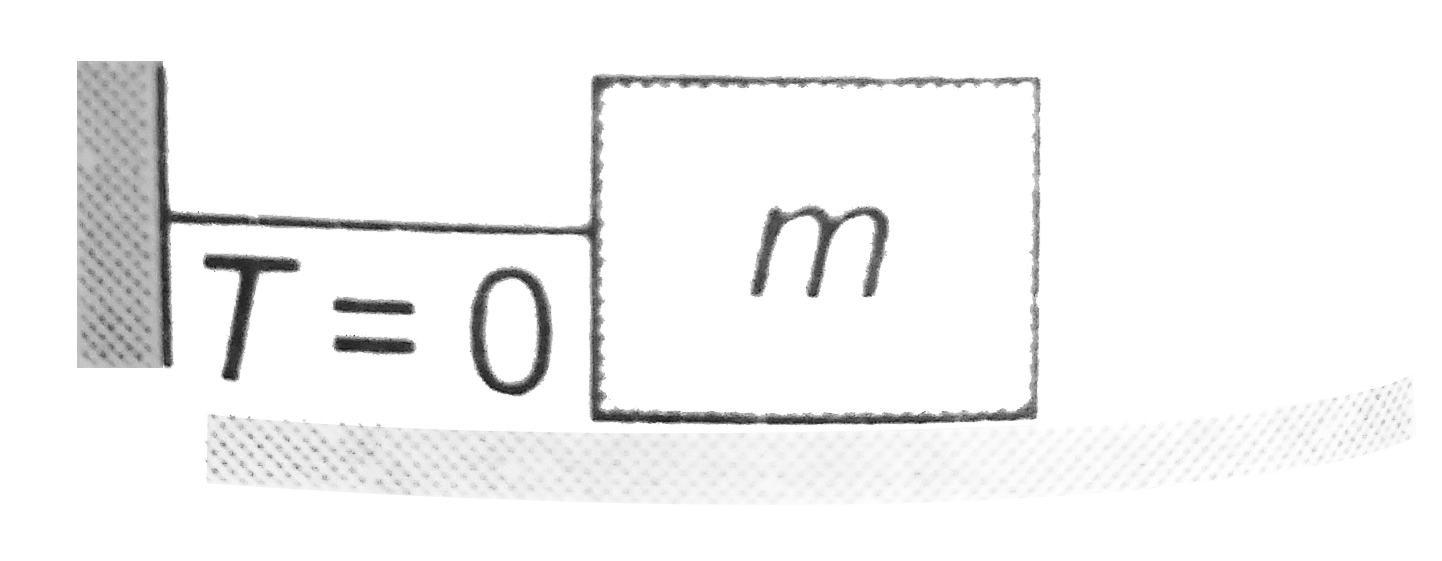
View SolutionA block of mass m is stationary on a horizontal surface. It is connected with a string which has no tension. The coefficient of friction between the block and surface is m . Then , the frictional force between the block and surface is:
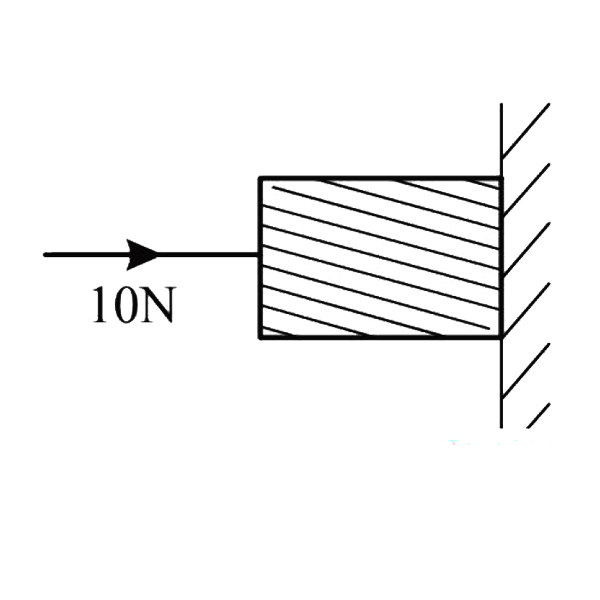
View SolutionA horizontal force of 10N is necessary to just hold a block stationary against as well. The coefficient of friction between the block and the wall is 0.2. The weight of the block is
View Solution