A wedge of mass M and angle α rests on a smooth horizontal plane. A smooth sphere of mass m strickes it in a direction perpendicular to its inclined face and rebounds. If the coefficient of restitution is e, find the ratio of the speed of the sphere just before and just after the impact.
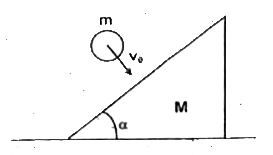
The correct Answer is:M+msin2αeM−msin2α
Step by step video, text & image solution for A wedge of mass M and angle alpha rests on a smooth horizontal plane. A smooth sphere of mass m strickes it in a direction perpendicular to its inclined face and rebounds. If the coefficient of restitution is e, find the ratio of the speed of the sphere just before and just after the impact. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I
45 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) Level - I (ASSERTION REASONING TYPE)
2 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) Level - I (Numerical)
8 Videos
- AC CIRCUITSBOOK - FIITJEECHAPTER - AC CIRCUITSEXERCISE - ASSERTION REASONING TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Comprehension -4
3 Videos
Similar Questions
A sphere of mass m moving with a uniform velocity v hits another identical sphere at rest. If the coefficient of restitution is e, the ratio of the velocities of the two sphere after the collision is e−1e+1.
View SolutionA particle of mass m moving with speed u hits a wedge of mass M as shown in figure. If coefficient of restitution is e and friciton can be neglected, then find the speed of the wedge with respect to ground just after the collision.
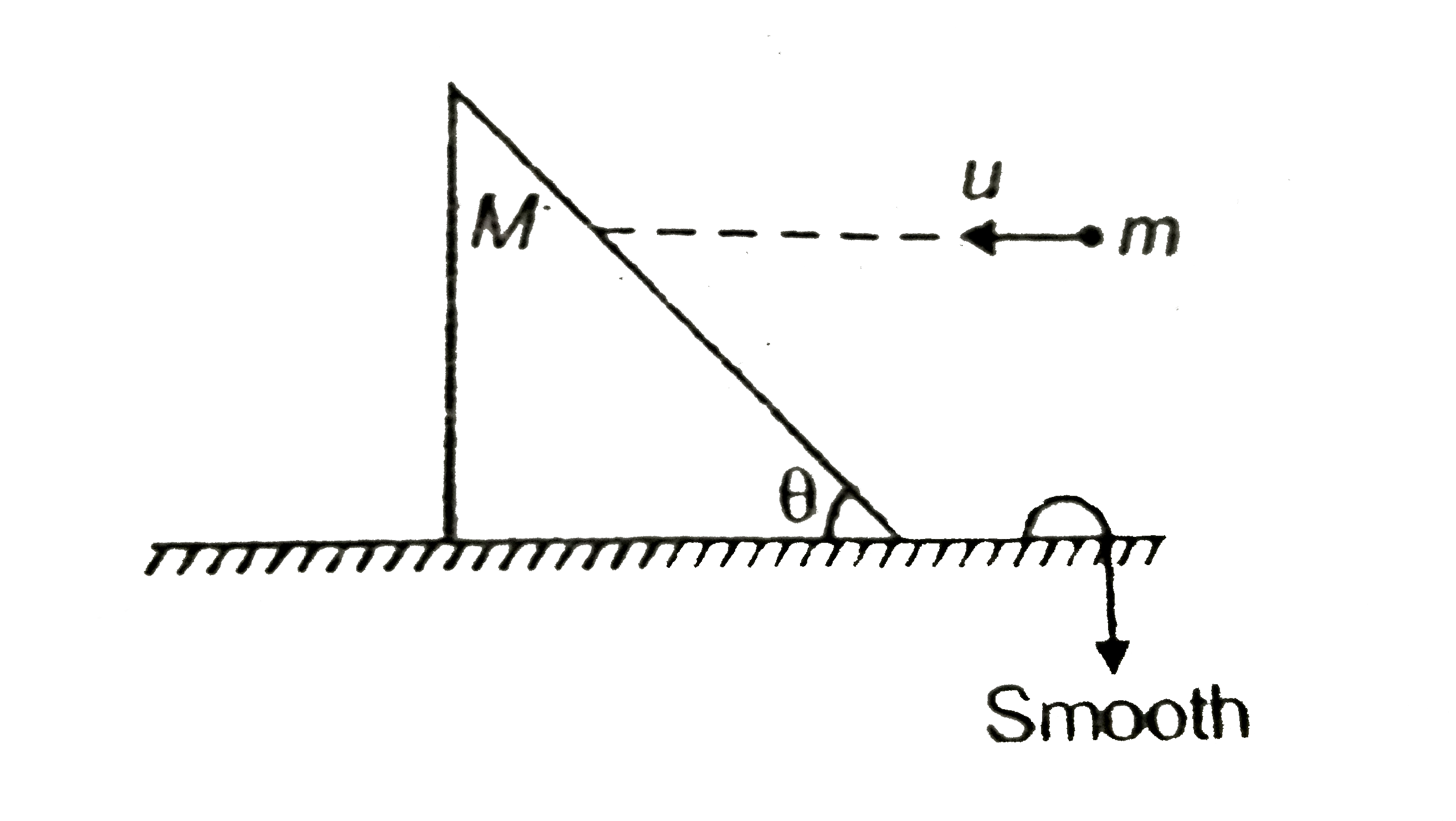
View Solution
Knowledge Check
A sphere of mass m moving with a velocity u hits another stationary sphere of same mass. If e is the coefficient of restitution, what is the ratio of the velocities of two spheres after the collision?
A1BeC1+e1−eD2−e1+eOne sphere collides with another sphere of same mass at rest inelastically. If the value of coefficient of restitution is 1/2, the ratio of their speeds after collision shall be-
A1:2B2:1C1:3D3:1A sphere of mass m moving with a constant velocity hits another stationary sphere of the same mass, if e is the coefficient of restitution, then ratio of speed of the first sphere to the speed of the second sphere after collision will be
A(1−e1+e)B(1+e1−e)C(e+1e−1)D(e−1e+1)
A uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
View SolutionA particle of mass m strikes on ground with angle of incidence 45∘. If coefficient of restitution, e=1√2, find the velociy after impact and angle of reflection.
View SolutionA ball is projected in a direction inclined to the vertical and bounces on a smooth horizontal plane. The range of one rebound is R. If the coefficient of restitution is e, then range of the next rebound is
View SolutionA sphere of mass m moving with a constant velocity u hits another stationary sphere of the same mass and of coefficient of restitution (e). The ratio of velocities of the two spheres, after collision, will be
View SolutionA ball of mass M strikes another smooth ball of mass m at rest. If they separate in mutually perpendicular directions, then the coefficient of impact (e) is :
View Solution
