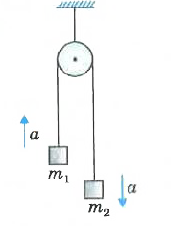
A light string passing over a smooth light pulley connects two blocks of masses m_(1) and m_(2) (vertically).If the acceleration of the system is g/8 then the ratio of masses "m_(1)" to "m_(2)" is "(" take "m_(1)>m_(2)]
8:1
9:7
4:3
5:3
The correct Answer is:B
Step by step video, text & image solution for A light string passing over a smooth light pulley connects two blocks of masses m_(1) and m_(2) (vertically).If the acceleration of the system is g/8 then the ratio of masses "m_(1)" to "m_(2)" is "(" take "m_(1)>m_(2)] by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE)(Level-1) (ASSERTION REASONING TYPE)2videos
LAWS OF MOTION
- BOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) (Level-II)23videos
LAWS OF MOTION
- BOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) (Level-II & III)18videos
LAWS OF MOTION
- BOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - NUMERICAL BASED QUESTIONS DECIMAL TYPE5videos
KINEMATICS
- BOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Numerical Based Type2videos
MAGNETIC
Similar Questions
Knowledge Check
- Question 1 - Select One
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 (vertically). If the acceleration of the system is g/8, then the ratio of masses is
A8:1B4:3C5:3D9:7 - Question 2 - Select One
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 (vertically). If the acceleration of the system is g/8, then the ratio of the masses is
A(a) 8:1B(b) 9:7C(c) 4:3D(d) 5:3 - Question 3 - Select One
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 (vertically). If the acceleration of the system is g/8, then the ratio of the masses is
A8:1B9:7C4:3D5:3
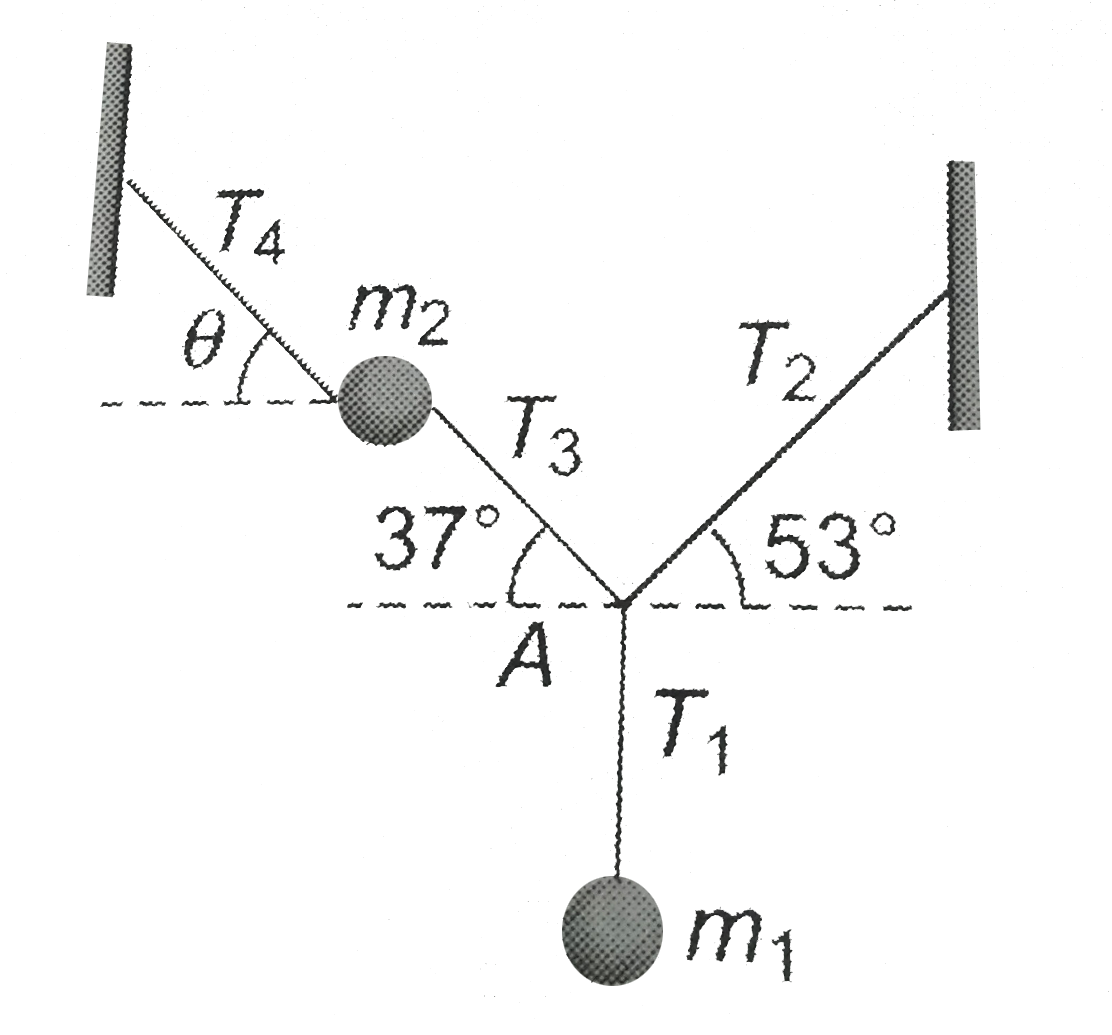
Two masses m1 and m2 are attached with light strings as shown. If the system is in equilibrium, find the value of θ
 View Solution
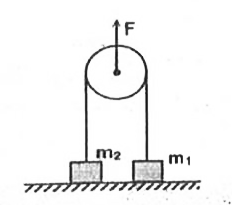
View SolutionTwo masses m1andm2 are connected by means of a light string, that passes over a light pulley as shown in the figure. If m1=2kgandm2=5kg and a vertical force F is applied on the pulley, then find the acceleration of the masses and that of the pulley when
(a) f = 35N, (b) F = 70N, (c) F = 140N.
 View Solution
View SolutionTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:
 View Solution
View SolutionThree blocks of masses m1,m2 and M are arranged as shown in figure. All the surfaces are fricationless and string is inextensible. Pulleys are light. A constant force F is applied on block of mass m1. Pulleys and string are light. Part of the string connecting both pulleys is vertical and part of the strings connecting pulleys with masses m1 and m2 are horizontal.
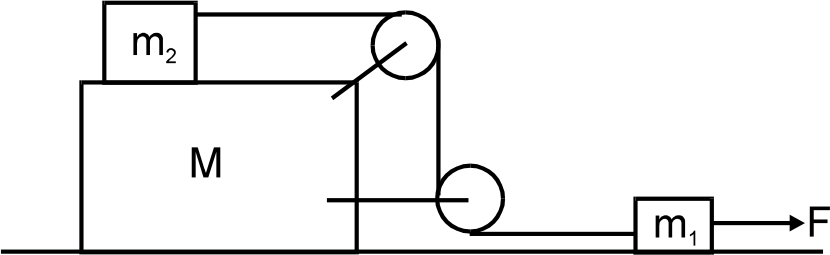
(P)Accleration of mass m1(1)Fm1(Q)Acceleration of massm2(2)Fm1+m2(R)Acceleration of mass M(3)zero(S)Tension in the string(4)m2Fm1+m2View SolutionConsider the case of two bodies of masses m1andm2 which are connected by light inextensible string passing over a light smooth pulley as shown in the figure. The expression for acceleration of the system and tension of the string are expressed below under different situations:
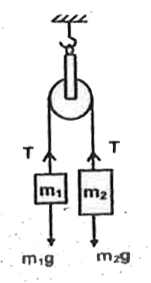
(i) when m1>m2. In this case
a=(m1−m2m1+m2)gandT=(2m1m2m1+m2)g
(ii) when m2>m1. In this case
a=(m2−m1m1+m2)gandT=(2m1m2m1+m2)g
(iii) When m1=m2=m. In this case a=0,T=mg
If the pulley is pulled upward with acceleration equal to the acceleration due to gravity, what will be the tension in the string?View Solution