The basic principle underlying the hall effect is the Lorentz force. Whenk an electron moves along a direction perpendicular to an applied magnetic field. It exeriences a force acting normal to both the directions and moves in response to this force and the force exerted by the internal electric field. For an - n-type bar shaped semiconductor, the carries are predominatly electrons of bulk density n. We assume that the constant current I flows along the x-axis from left to right in the presence of a magnetic field toward the negative z-axis resulting in an excess surface electrical charge on the sides of the sample. this charge results in the hall voltage a potential drop across the two sides of the sample
Thre transverse voltage is the hall voltage VH and its magnitude is equal to IB/qnD, where I is the current B is the magnetic field, d is the sample thickness and q is the elementary charge. A silver ribbon lies as shown in the adjacent figure (z1=11.8mm and = 0.23 mm) carrying a current of 120 A in the x -direction in unifrom magnetic field B = 0.95 T. If electron density is 5.85×1028/m3 then
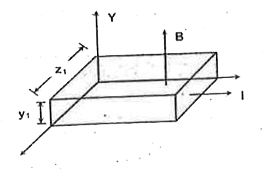
What is the value of hall emf?
53 mv
53μV
5.3 mV
5.3μV
The correct Answer is:B
Topper's Solved these Questions
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Comprehension -4
2 Videos
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Numerical Based Type
2 Videos
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Comprehension -2
3 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
- MAGNETISMBOOK - FIITJEECHAPTER - MAGNETISMEXERCISE - Example
12 Videos
Similar Questions
A 100 turn closely wound circular coil of radius 10 cm carries a current of 3.2A. A uniform horizontal magnetic field of strength 2T is turned on. The axis of the coil is initially in the direction of the field . The coil rotates through an angle of 90∘ under the influence of the magnetic field. The moment of inertia of the coil is 0.1 kg m2
What is the field at the centre of the coil?
View SolutionTwo circular coils X and Y, having equal number of turns and carrying currents in the same sense, subtend same solid angle at point O. If the smaller coil X is midway between O and Y and if we represent the magnetic induction due to bigger coil Y at O as By and the due to smaller coil X at O as Bx,then find the ratio Bx/By.
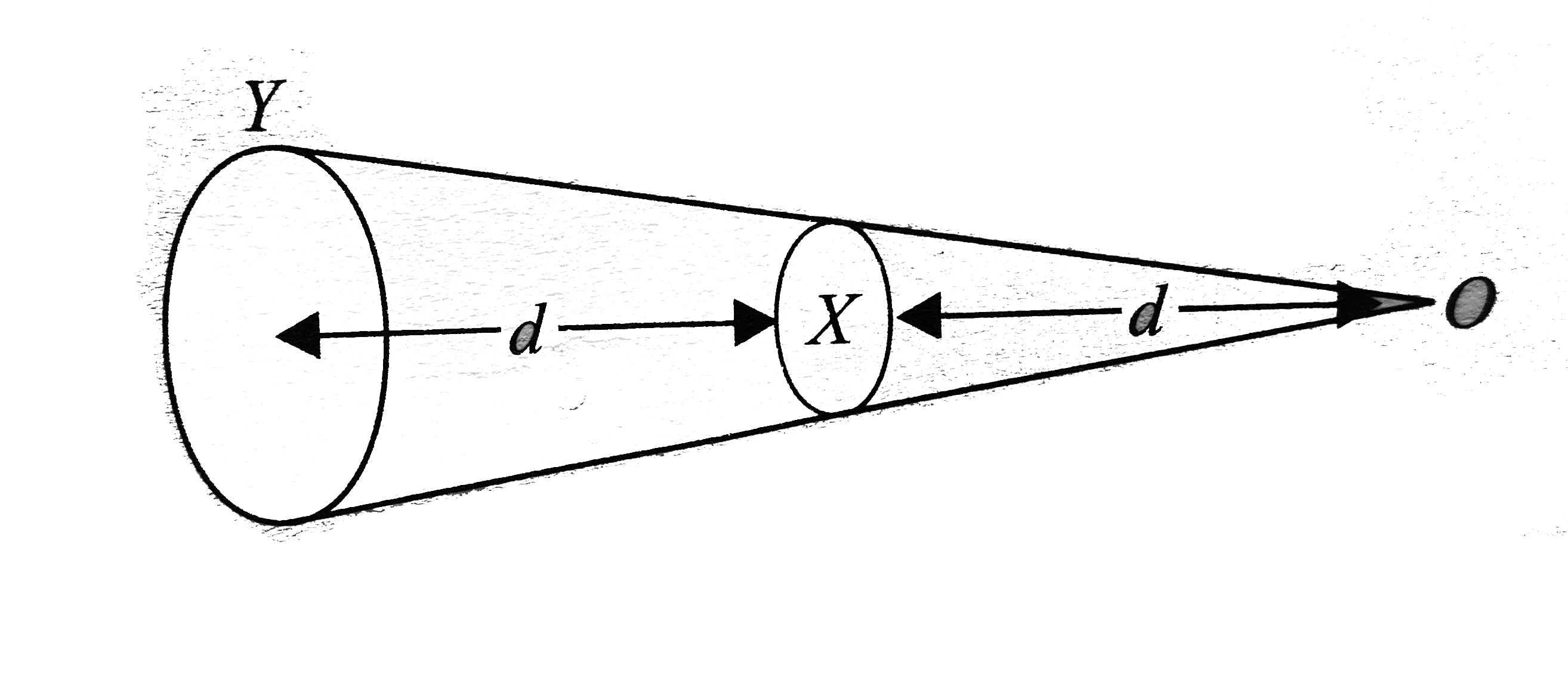
View SolutionTo know the surface of matter on smallest scale we allow an energetic charged particle to slam into a solid target or allow two energetic charged particle to collide head on. We use cyclotrons to give very high kinetic energy to the charged particles, we arrange for the proton to circulate in a magnetic field and give it a modest electrical kick once per revolution. Two hollow D shaped objects, called does are part of an electrical oscillator that alternates the electric potential difference across the gap between the does back ad forth. the does are immersed in a magnetic field whose direction is out of the plane of page and that is set up by a large electromagnet, suppose a proton injected by a source S at the centre of the cyclotron, initially moves towards a negatively charged de. It will accelerate toward this doe and enter it. Once inside, it is shielded from electric fields by the copper walls of the dee: that is the electric field does not enter the dee. The magnetic field however is not screened by the copper dee. So, the proton moves in a circular path whose radius, which depends on speed is given by r=mvqB
The key to the operation of cyclotron is that the frequency f at which the proton circulates in the field and thaat does not depend on its speed must be equal to the fixed frequency Foce of the electrical oscillator. The resonance condition is given by
qB=2πmFoce
If a proton circulates 200 times in a magnetic field of 1.5 T and receives an energy boost of 100 keV every time it completes an orbit it will end up with a kinetic energy of
View SolutionGenerally, we consider that during slowing of a moving automobile, its retardation is constant, but in practice this is seldom the case. Under many circumstances, especially at high speed, we usually apply the brakes slowly at first and then more strongly as the car slows. The braking force therefore depends on the time during the interval over which the car is slowing and acceleration changes with time.
ax(t)=vA−vxdt=Fx(t)m
∫vxv0xdVx=∫10Fx(t)mdt
∴Vx=V0x+1m∫10Fx(t)dt
x(t)=x0+∫10Vx(t)dt
An example for the same is discussed here.
A car of mass m = 1000 kg is moving with 25 m/s. The driver begins to apply the brakes so that the magnitude of the braking force increases linearly with time at a rate of 2000 N/s.
Read the above passage carefully and answer the following questions.
Find the velocity of the car at t = 10 sec.
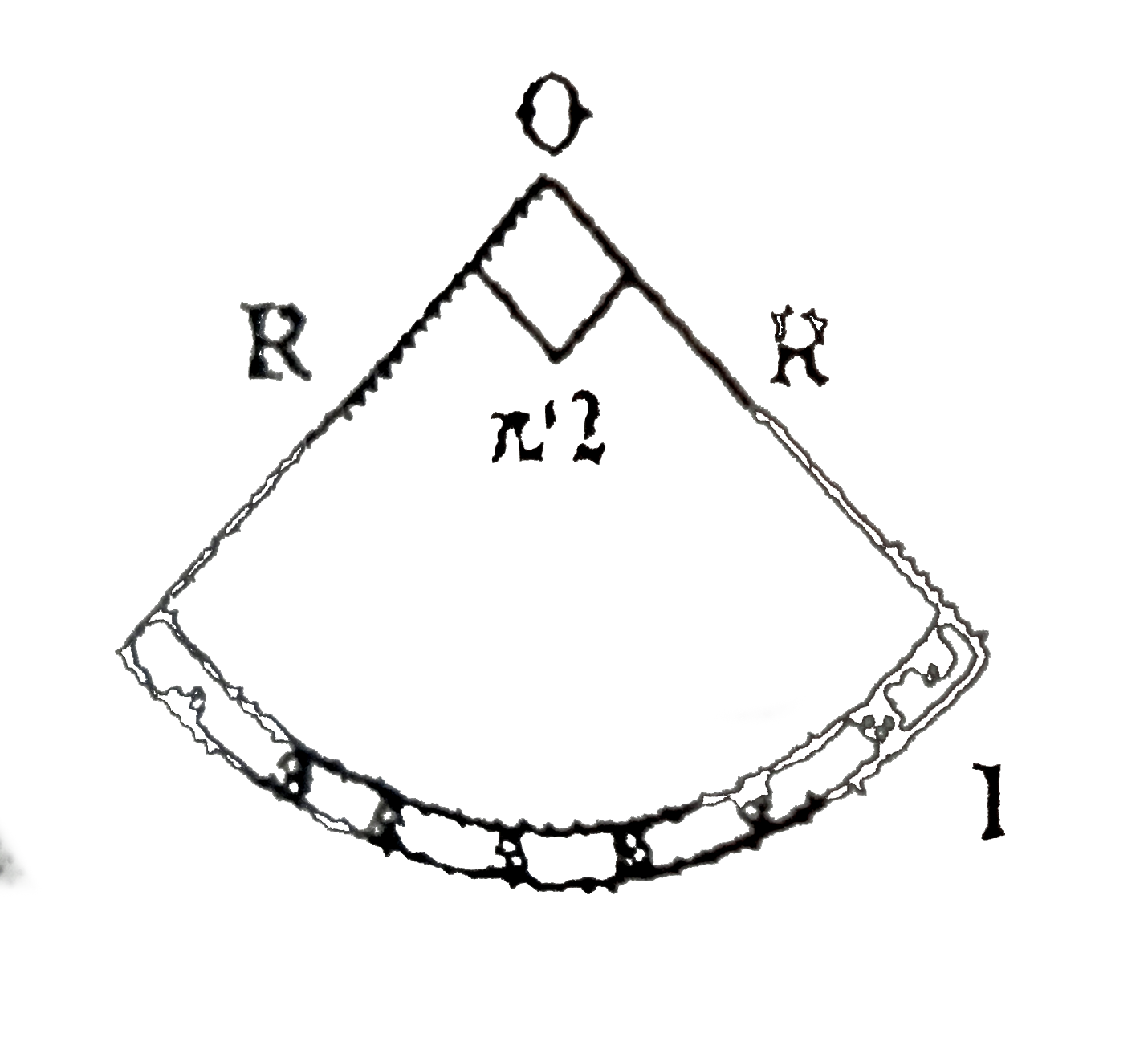
View SolutionA current I flows in a long straight wire (into the plane of the paper) with cross-section having the from of a circular are of radius R a shown in the figure. Find the magnetic induction at the point O.
View SolutionWhat is the magnitude and direction of force on an electron moving along the direction of the magnetic field.
View SolutionThe charge is moving along the direction of magnetic field. Then force acting on it is………
View SolutionAn electron having charge e moves with velocity →n in +x direction. An electric field act on it along+y direction. The force on the electron acts along .
View Solutionजब एक इलेक्ट्रॉन चुंबकीय क्षेत्र के .........गति करता है तो उस पर कोई बल कार्य नहीं करता।
View SolutionThe basic principle underlying the hall effect is the Lorentz force. Whenk an electron moves along a direction perpendicular to an applied magnetic field. It exeriences a force acting normal to both the directions and moves in response to this force and the force exerted by the internal electric field. For an - n-type bar shaped semiconductor, the carries are predominatly electrons of bulk density n. We assume that the constant current I flows along the x-axis from left to right in the presence of a magnetic field toward the negative z-axis resulting in an excess surface electrical charge on the sides of the sample. this charge results in the hall voltage a potential drop across the two sides of the sample Thre transverse voltage is the hall voltage V_(H) and its magnitude is equal to IB/qnD, where I is the current B is the magnetic field, d is the sample thickness and q is the elementary charge. A silver ribbon lies as shown in the adjacent figure (z_(1) = 11.8 mm and = 0.23 mm) carrying a current of 120 A in the x -direction in unifrom magnetic field B = 0.95 T. If electron density is 5.85 xx 10^(28)//m^(3) then magnitude of the drift velocity of electron is
View SolutionThe basic principle underlying the hall effect is the Lorentz force. Whenk an electron moves along a direction perpendicular to an applied magnetic field. It exeriences a force acting normal to both the directions and moves in response to this force and the force exerted by the internal electric field. For an - n-type bar shaped semiconductor, the carries are predominatly electrons of bulk density n. We assume that the constant current I flows along the x-axis from left to right in the presence of a magnetic field toward the negative z-axis resulting in an excess surface electrical charge on the sides of the sample. this charge results in the hall voltage a potential drop across the two sides of the sample Thre transverse voltage is the hall voltage V_(H) and its magnitude is equal to IB/qnD, where I is the current B is the magnetic field, d is the sample thickness and q is the elementary charge. A silver ribbon lies as shown in the adjacent figure (z_(1) = 11.8 mm and = 0.23 mm) carrying a current of 120 A in the x -direction in unifrom magnetic field B = 0.95 T. If electron density is 5.85 xx 10^(28)//m^(3) then What is the magnitude and direction of electric field ?
View SolutionAn electron is moving along X-axis in a magnetic field acting along Y-axis. What is the direction of magnetic force acting on it?
View Solutionचुम्बकीय क्षेत्र में उसकी दिशा के समान्तर एक इलेक्ट्रॉन गति कर रहा है। इलेक्ट्रॉन पर बल
View SolutionAn electron having charge e moves with velocity vecv in +x direction. An electric field acts on it along +y direction. The force on the electron acts along-
View SolutionAn electron is moving along X axis and a magnetic field is directed along Y axis. What will he the direction of magnetic force acting on the electron?
View Solution
