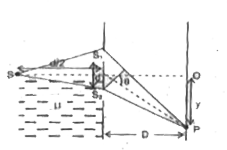
A primary monochromatic coherent source is used in YDSE and half of the space between the primary source and the slits is filled with a transparent liquid of refractive index p with respect to air, such that path of the wave SS2 is through this liquid. Everywhere else there is air and slits are sealed with equal glass plates. Find the location of central maximum.

Complete the optical path length covered by the two waves created from the primary source S if P is a point of observation for which path lengths for both the waves are equal, then P will be the central maximum.
Hence, for central maximum,
SS2−SS1=S1P−S2P
⇒μ√(d2)2+(d2)2−√(d2)2+(d2)2=dsinθ=dyD
⇒y=(μ−1)D/√2=(μ−1)1000√2d
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - OPTICSEXERCISE - SOLVED PROBLEMS (SUBJECTIVE)18videos
OPTICS
- BOOK - FIITJEECHAPTER - OPTICSEXERCISE - Solved problem (objective)15videos
OPTICS
- BOOK - FIITJEECHAPTER - OPTICSEXERCISE - NUMERICAL BASED QUESTIONS2videos
OPTICS
- BOOK - FIITJEECHAPTER - MODERN PHYSICSEXERCISE - Numerical based questions5videos
MODERN PHYSICS
- BOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - NUMERICAL BASED QUESTIONS DECIMAL TYPE11videos
PHYSICS PART-III
Similar Questions
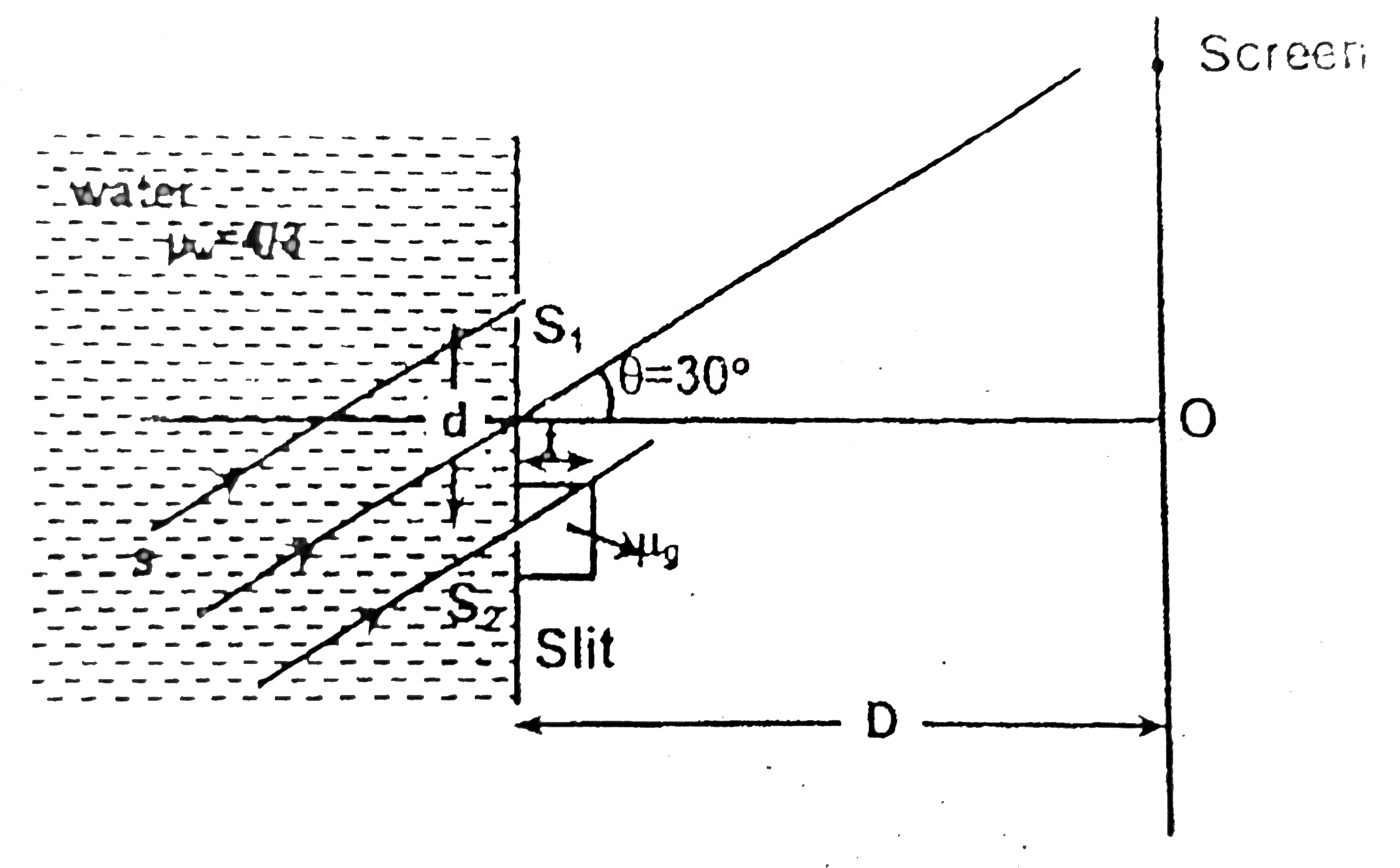
In a YDSE experiment the two slits are covered with a transparent membrane of negligible thickness which allows light to pass through it but does not allow water. A glass slab of thickness t=0.41mm and refractive index μg=1.5 is placed infront of one of the slits as shown in the figure. The separation between the slits is d=0.30mm. The entire space to the left of the slits is filled with water of refractive index μw=4/3.
A coherent light of intensity I and absolute wavelength λ=5000Å is being incident on the slits making an angle 30∘ with horizontal. If screen is placed at a distance D=1m from the slits, find
(a) the position of central maxima.
(b) the intensity at point O.
 View Solution
View SolutionIn a YDSE experiment the two slits are covered with a transparent membrane of negligible thickness which allows light to pass through it but does not allow water. A glass slab of thickness t=0.41mm and refractive index μg=1.5 is placed infront of one of the slits as shown in the figure. The separation between the slits is d=0.30mm. The entire space to the left of the slits is filled with water of refractive index μw=4/3.
A coherent light of intensity I and absolute wavelength λ=5000Å is being incident on the slits making an angle 30∘ with horizontal. If screen is placed at a distance D=1m from the slits, find
(a) the position of central maxima.
(b) the intensity at point O.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.
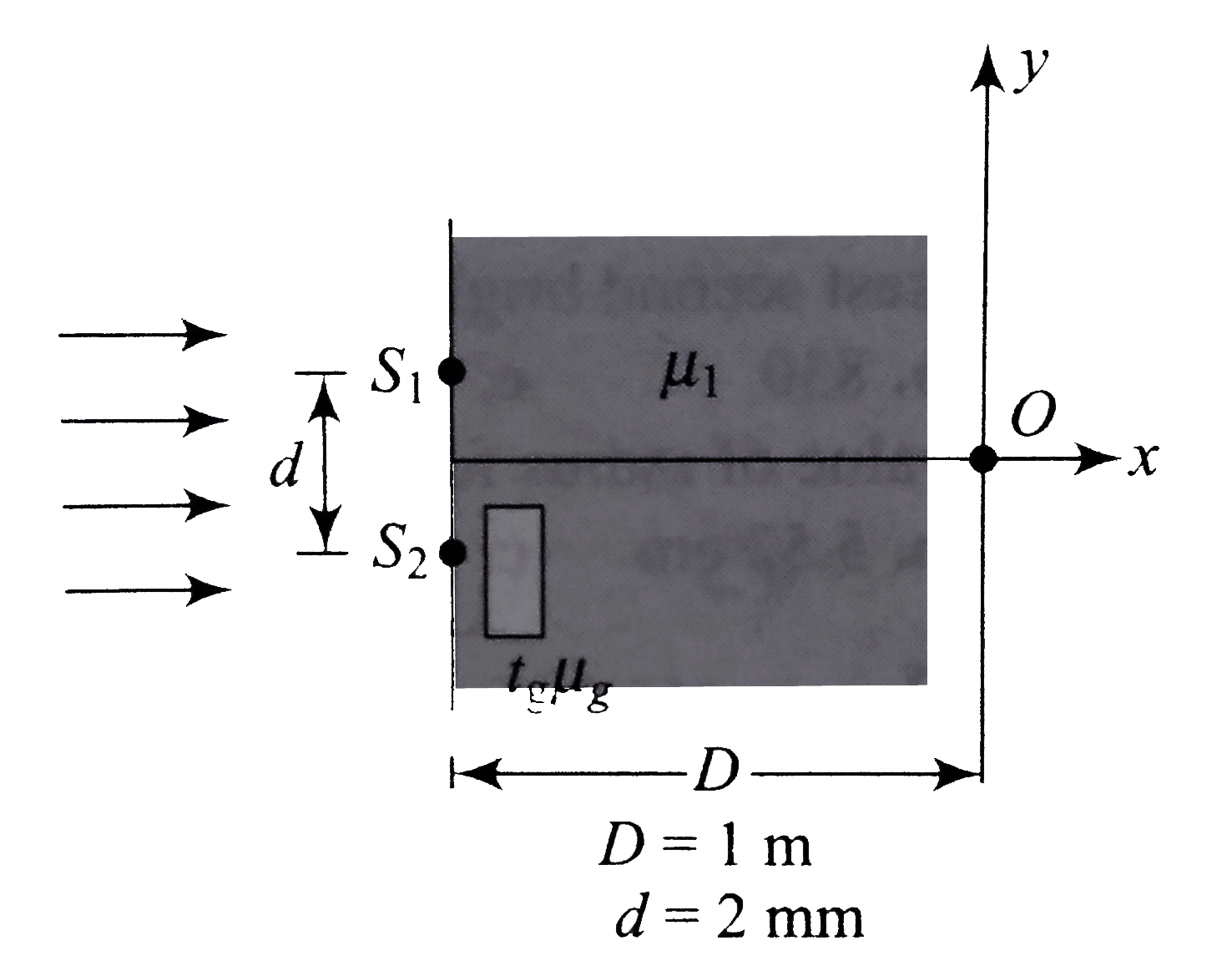
Find the time when central maxima is at point O. located symmetrically on the x-asix.A2 sB4 sC6 sD8 s - Question 2 - Select One
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

What is the speed of the central maxima when it is atA3×10−3ms−1B4×10−3ms−1C6×10−3ms−1D1×10−3ms−1 - Question 3 - Select One
Velocity of light in glass whose refractive index with respect to air is 1.5 is 2×108m/s and in certain liquid the velocity of light found to be 2.5×108m/s. The refractive index of the liquid with respect to air is
A0.64B0.80C1.20D1.44
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

Find the time when central maxima is at point O. located symmetrically on the x-asix.View SolutionIn a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

What is the speed of the central maxima when it is atView SolutionMonochromatic light of lamba=5000Å is incident on two slits separately by a distance of 5×10−4m. The interference patteren is seen on a screen placed at a distance of 1m form the slits. A thing glass plate of thicknes 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the centre of the screen if the density there is I0 in the absence of the plate. Also find the lateral shift of the central maximum. '
View SolutionVelocity of light in glass whose refractive index with respect to air is 1.5 is 2×108m/s and in certain liquid the velocity of light found to be 2.5×108 m/s . The refractive index of the liquid with respect to air is
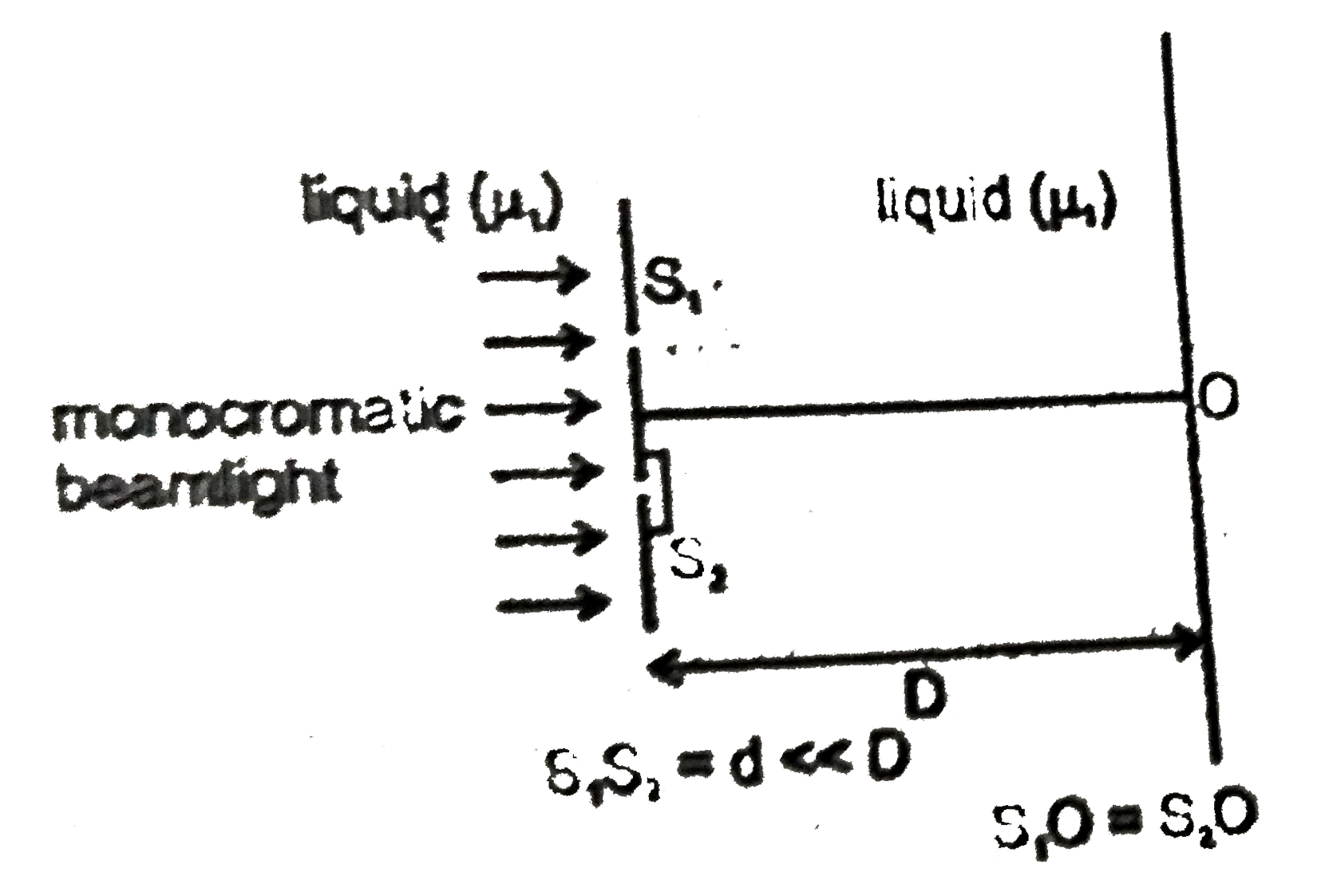
View SolutionYoung's double slit experiment is conducted in a liquid of refractive index μ1 as shown in figure. A thin transparent slab of refractive index μ2 is placed in front of the slit s2. The magnitude of optical path difference at 'O' is
 View Solution
View Solution

