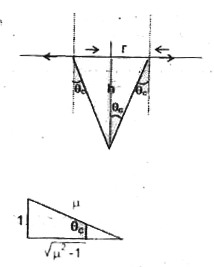
A small coin is placed at the bottom of a cylindrical vessel of radius R and height h. If a transparent liquid of refractive index μ completely filled into the cylinder, find the minimum fraction of the area that should be covered in order not to see the coin.
When the rays coming from the coin incident at an angle θ≥θc, they will be totally reflected.
⇒ Minimum area that should be covered = A=πr2 where r = radius of the circular aperture r can be found as
r=htanθc
where θc can be determined by the formula
sinθc=μaμ=1μ
⇒tanθc=1√μ2−1
∴A=πr2=πh2μ2−1
Topper's Solved these Questions
- OPTICSBOOK - FIITJEECHAPTER - OPTICSEXERCISE - SOLVED PROBLEMS (SUBJECTIVE)
18 Videos
- OPTICSBOOK - FIITJEECHAPTER - OPTICSEXERCISE - Solved problem (objective)
15 Videos
- OPTICSBOOK - FIITJEECHAPTER - OPTICSEXERCISE - NUMERICAL BASED QUESTIONS
2 Videos
- MODERN PHYSICSBOOK - FIITJEECHAPTER - MODERN PHYSICSEXERCISE - Numerical based questions
5 Videos
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - NUMERICAL BASED QUESTIONS DECIMAL TYPE
11 Videos
Similar Questions
A small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index -of water = 1-33.
View SolutionA small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index -of water = 1-33.
View Solution
Knowledge Check
A cylindrical vessel of radius R and height H and open at the top is completely filled with y . A small circular hole of radius r is made near the bottom of vessel . The time taken for 25 % of water to flow out is
A√2Hg(√3−1)BR2r2√2Hg(1+2√3)CR2r2√2Hg(1−2√3)DR2r2√2Hg(√3−1)A plane mirror is placed at the bottom of a tank containing a liquid of refractive index μ. P is a small object at a height h above the mirror. An observes P and its image in the mirror. The apparent distance between two will be
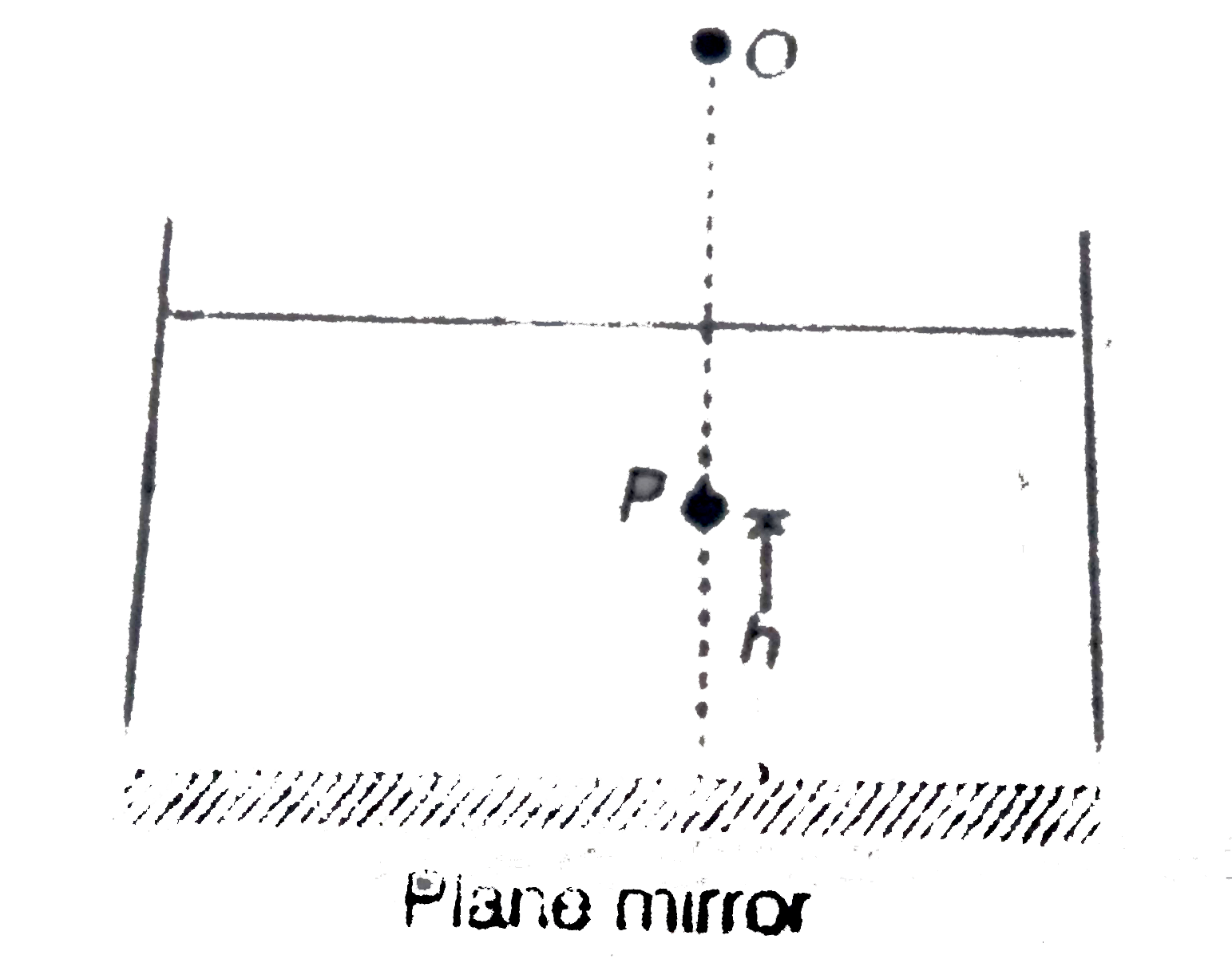 A2μhB2hμC2hμ−1Dh(1+1μ)
A2μhB2hμC2hμ−1Dh(1+1μ)
A person looking through a telescope focuses the lens at a point on the edge of the bottom of an empty cylindrical vessel Next he fills the entire vessel with a liquid of refractive index μ, without disturbing the telescope. Now, he observes the midpoint of the bottom of the vessel Determine the radius to depth ratio of the vessel
View SolutionA person looking through a telescope focuses lens at a point on the edge of the bottom of an empty cylindrical vessel. Next he fills the entire vessel with a liquid oif refractive index μ, without disturbing the telescope. Now, he observes the mid point of the vessel. Determine the radius to depth ratio of the vessel
View SolutionFigure shows a concave mirror of focal length F with its principal axis vertical. In mirror a transparent liquid of refractive index μ is filled upto height d. Find where on axis of mirror a pin should be placed so that its image will be formed on itself.
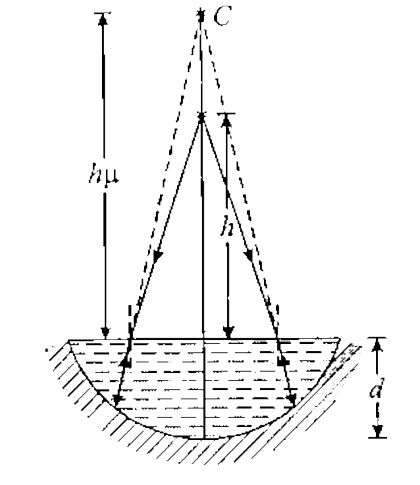
View SolutionA small illuminated bulb is at the bottom of a tank, containing a liquid of refractive index μ upto a height H. Find the expression for the diameter of an opaque disc, floating symmetrically on the liquid surface in order to cut- off the light from the bulb.
View SolutionA small illuminated bulb is at the bottom of a tank, containing a liquid of refractive index μ upto a height H. Find the expression for the diameter of an opaque disc, floating symmetrically on the liquid surface in order to cut- off the light from the bulb.
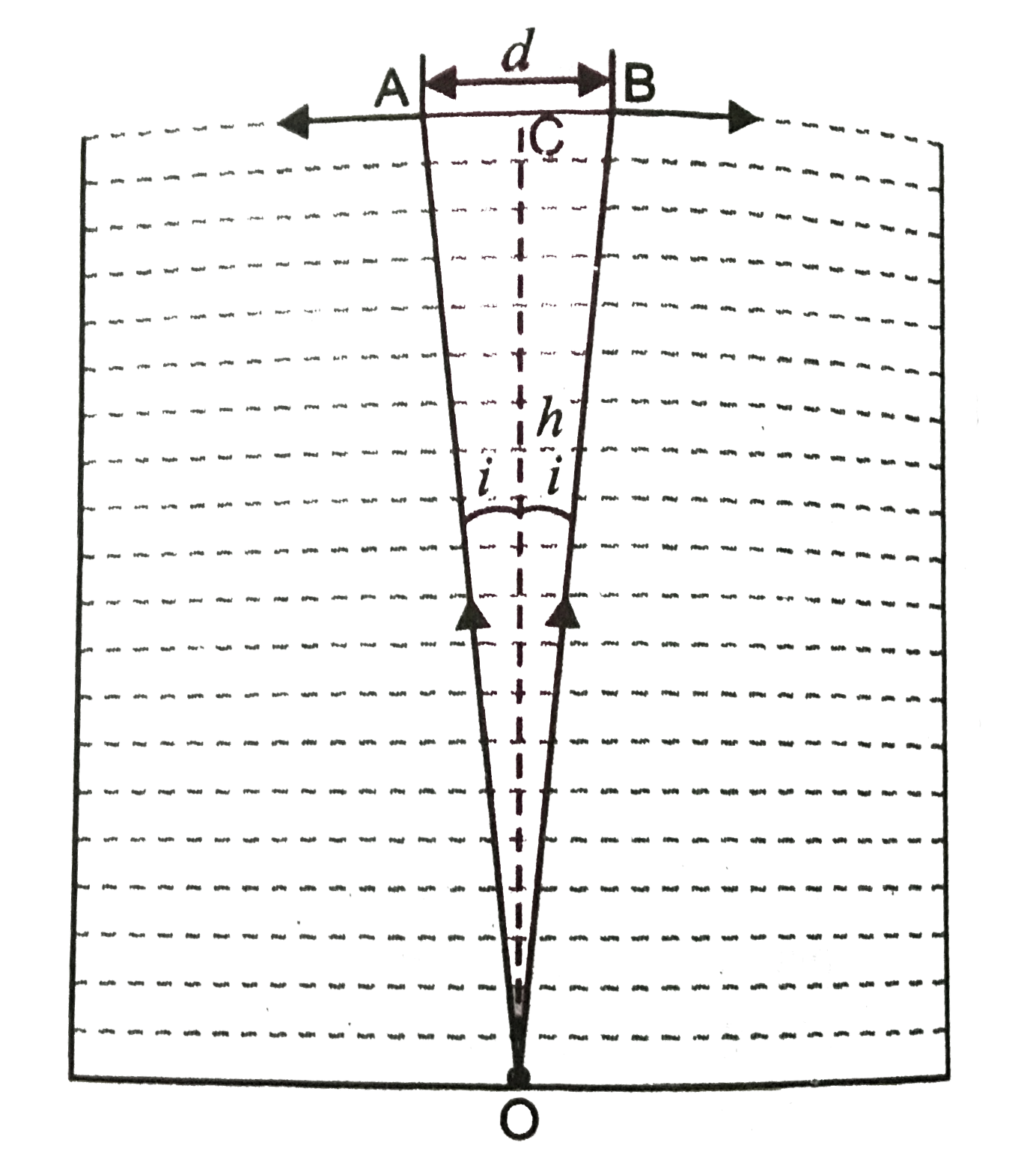
View SolutionA jar of height h is filled wih a transparent liquid of refractive index μ, Fig. At the centre of the jar on the botom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.
 .
.
View Solution
