Two particles A and B of mass m each and moving with velocity v, hit the ends of a rigid bar of the same mass m and length l simultaneously and stick to the bar as shown in the figure. The bar is kept on a smooth horizontal plane. The linear and angular speed of the system (bar + particle) after the collision are

vcm=0,ω=127vl
vcm=0,ω=4vl
vcm=0,ω=5vl
vcm=0,ω=v5l
The correct Answer is:A
By conservation of linear momentum, we have
mv−mv=(m+m+m)vcmorvcm=0
Now using conservation of angular momentum, we get
mvl=Iω=[ml212+2m(l/2)2]ω ∴ω=12v7l
Topper's Solved these Questions
- SYSTEM OF PARTICLES & ROTATIONAL MOTION BOOK - DISHA PUBLICATIONCHAPTER - SYSTEM OF PARTICLES & ROTATIONAL MOTION EXERCISE - EXERCISE-1 : CONCEPT BUILDER
58 Videos
- SEMICONDUCTOR ELECTRONICS : METERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - DISHA PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS : METERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - EXERCISE -2: CONCEPT APPLICATOR
25 Videos
- THERMAL PROPERTIES OF MATTER BOOK - DISHA PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTER EXERCISE - Exercise-2 (Concept Applicator)
28 Videos
Similar Questions
Two particles A and B of mass m each hit the ends of a rigid bar of mass M and length l in-elastically from opposite sides the speeds v and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar+particle).

View SolutionTwo particles A and B of mass m each hit the ends of a rigid bar of mass M and length l inelastically from opposite sides the speeds v and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar+particle).

View Solution
Knowledge Check
Two particles A and B of mass m each hit the ends of a rigid bar of mass M and length l inelastically from opposite sides the speeds v and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar+particle).
 Avcm=0,ω=217vlBvcm=0,ω=4vlCvcm=0,ω=5vlDvcm=0,ω=v5l
Avcm=0,ω=217vlBvcm=0,ω=4vlCvcm=0,ω=5vlDvcm=0,ω=v5lA particle of mass m moving with velocity v strikes a stationary particle of mass 2m and sticks to it. The speed of the system will be.
Av//2B2 vCv//3D3 vA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
A0.8vB0.2vC0.6vD0.4v
Find the acceleration of the prism of mass M and that of the bar of mass m shown in figure.
View SolutionA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
View SolutionA rigid uniform rod of mass M and length L is placed along the Y-axis on a smooth horizontal surface with its centre at the origin. Two particles , each of mas m(=M/12) hits simultaneously , at the two ends with equal speed v as shown in the figure. The collision is completely inelastic.
(i) Find the angular velocity of the system after the collision.
(ii) Two particles of mass 2m and m are placed respectively at ( L/2,0) and (−L/2,0). Calculate the time when the rod hits these particles.
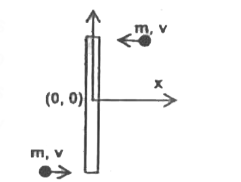
View SolutionA rigid uniform rod of mass M and length L is placed along the Y-axis on a smooth horizontal surface with its centre at the origin. Two particles , each of mas m(=M/12) hits simultaneously , at the two ends with equal speed v as shown in the figure. The collision is completely inelastic.
(i) Find the angular velocity of the system after the collision.
(ii) Two particles of mass 2m and m are placed respectively at ( L/2,0) and (−L/2,0). Calculate the time when the rod hits these particles.

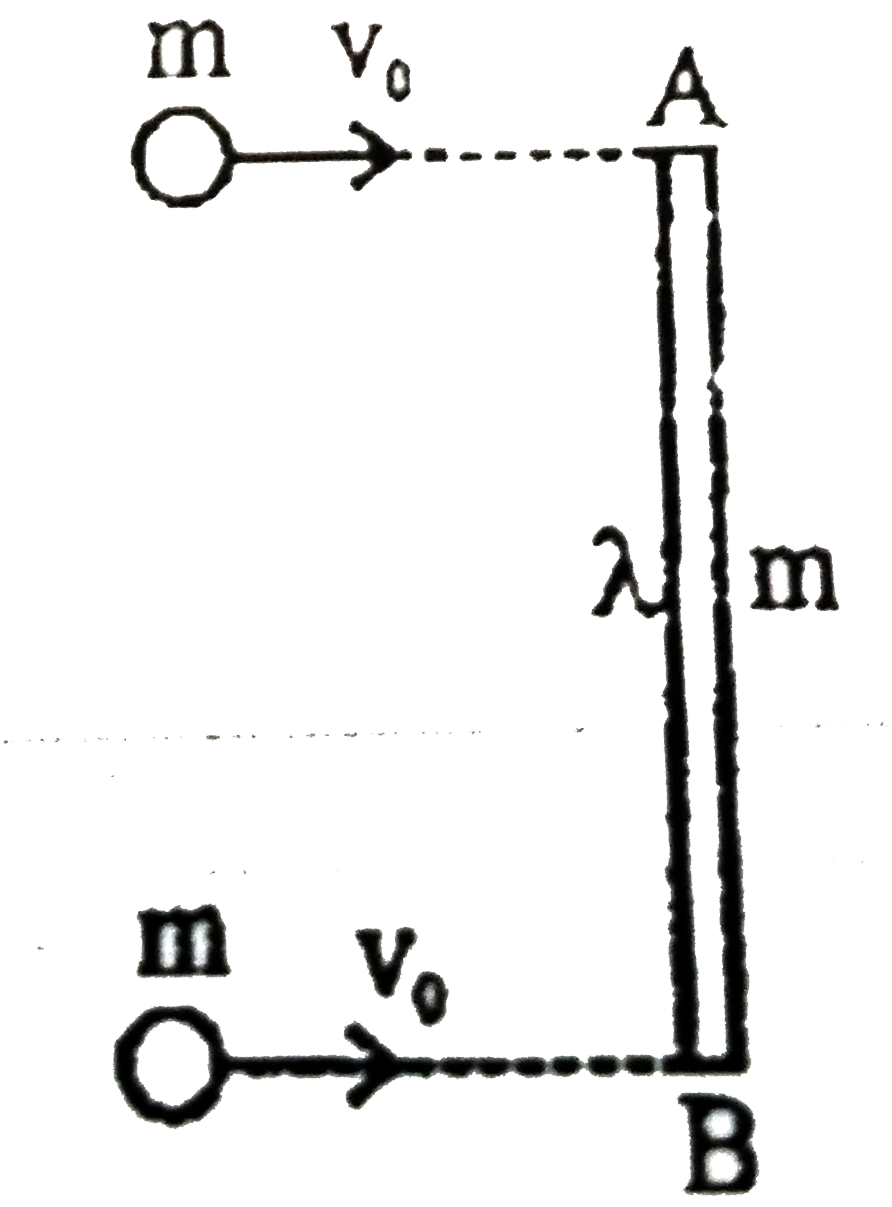
View SolutionRod AB is placed on smooth horizontal plane and two particle each having same mass m as rod, strikes the rod simultaneously and stick as it as shown. Find the loss in kinetic energy due to collision.
View Solution
