The vertical motion of a ship at sea is described by the equation dxdt2=−4x , where x is the vertical height of the ship (in meter) above its mean position. If it oscillates through a height of 1 m
The correct Answer is:[2 m/s,−4 m/s2]
Step by step video, text & image solution for The vertical motion of a ship at sea is described by the equation (d^(x))/(dt^(2))=-4x , where x is the vertical height of the ship (in meter) above its mean position. If it oscillates through a height of 1 m by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Energy & spring pendulum)16videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Simple pendulum and types of SHM)17videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Thermal Physics) (Thermodynamic process)21videos
RACE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
The roots of the equation x4−2x2+4=0 are the vertices of a :
Asquare inscribed in a circle of radius 2Brectangle inscribed in a circle of radius 2Csquare inscribed in a circle of radius √2Drectangle inscribed in a circle of radius √2 - Question 2 - Select One
The equation of SHM of a particle is given as 2d2xdt2+32x=0 where x is the displacement from the mean position. The period of its oscillation ( in seconds) is -
A4Bπ2Cπ2√2D2π - Question 3 - Select One
A body is projected horizontally from a point above the ground and motion of the body is described by the equation x=2t, y=5t2 where x, and y are horizontal and vertical coordinates in metre after time t. The initial velocity of the body will be
A√29 m/s horizontalB5m/s horizontalC2m/s verticalD2 m/s horizontal
What is the velocity of the bob of a simple pendulum at its mean position, if it is able to rise to vertical height of 10 cm (take g=9.8m/s2)
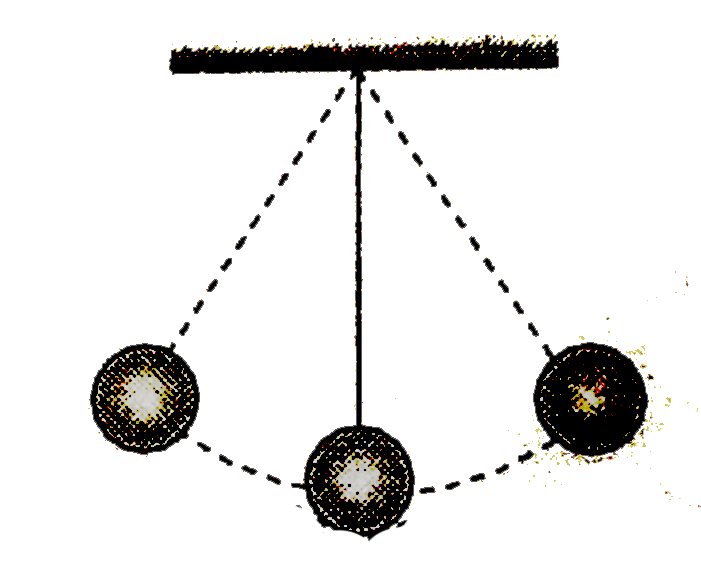 View Solution
View SolutionA body is projected horizontally from a point above the ground. The motion of the body
is described by the equations x = 2 t and y=5t2 where x and y are the horizontal and vertical displacements (in m) respectively at time t. What is the magnitude of the velocity of the body 0.2 second after it is projected?View SolutionThe solution of the differential equation
d2xdt2+x=0,x(0)=1,x'(0)=0View SolutionThe equation of motion of a particle of mass 1g is d2xdt2+π2x=0, where x is displacement (in m) from mean position. The frequency of oscillation is (in Hz)
View SolutionThe equation of a particle executing SHM is d2xdt2=−ω2x. Where ω=2πtime period. The velocity of particle is maximum when it passes through mean position and its accleration is maximum at extremeposition. The displacement of particle is given by x=Asin(ωt+θ) where θ-initial phase of motion. A-Amplitude of motion and T-Time period
The time period of pendulum is given by the equation T=2π√lg. Hered2xdt2 is :View Solution

