The acceleration of a particle moving along x-axis is a=−100x+50. It is released from x=2. Here a and x are in S.I units. The motion of particle will be:
periodic, oscillatory but not SHM
periodic but not oscillatory
oscillatory but not periodic
simple harmonic
The correct Answer is:D
Step by step video, text & image solution for The acceleration of a particle moving along x-axis is a=-100x+50. It is released from x=2. Here a and x are in S.I units. The motion of particle will be: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Energy & spring pendulum)
16 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Simple pendulum and types of SHM)
17 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Thermal Physics) (Thermodynamic process)
21 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
The acceleration of a particle moving along x -axis is a= −100x.It is released from rest at x=2.Here a and x are in S.I.units.The minimum time taken by particle to go from x=2 to x=0 is πα seconds.Find the value of α4.
View Solutionx-coordinate of a particle moving along this axis is x=(2+t2+2t3). Here, x is in meres and t in seconds. Find (a) position of particle from where it started its journey, (b) initial velocity of particle and (c) acceleration of particle at t=2s.
View Solution
Knowledge Check
The acceleration of a particle moving along x-axis is a = –200 x + 50. It is released from x = 2. Here 'a' and 'x' are in S.I. units. The motion of particle will be :-
APeriodic, oscillatory but not SHMBPeriodic but not oscillatoryCOscillatory but not periodicDSimple harmonicThe acceleration of a particle is a=−100x+50. It is released from x=2. Here, a and x are in SI units
Athe particle will perform SHM of amplitude 2mBthe particle will perform SHM of amplitude 1.5mCthe particle will perform SHM of time period 0.63sDthe particle will have a maximum velocity of 15m/sFor a particle moving along the x-axis, mark the correct statement(s).
Aif x is positive and is increasing with the time, then average velocity of the particle is positive.Bif x is negative and becoming positive after some time, then the velocity of the particle is always positive.CIf x is negative and becoming less negative as time passes, then the average velocity of the particle is positive.DIf x is positive and is increasing with time, then the velocity of the particle is always positive.
The position x of particle moving along x-axis varies with time t as x=Asin(ωt) where A and ω are positive constants. The acceleration a of particle varies with its position (x) as
View SolutionThe displacement of a particle moving along x-axis is given by :
x=a+bt+ct2
The acceleration of the particle is.
View SolutionThe velocity (υ) of a particle moving along X-axis varies with its position x as shown in figure. The acceleration (a) of particle varies with position (x) as
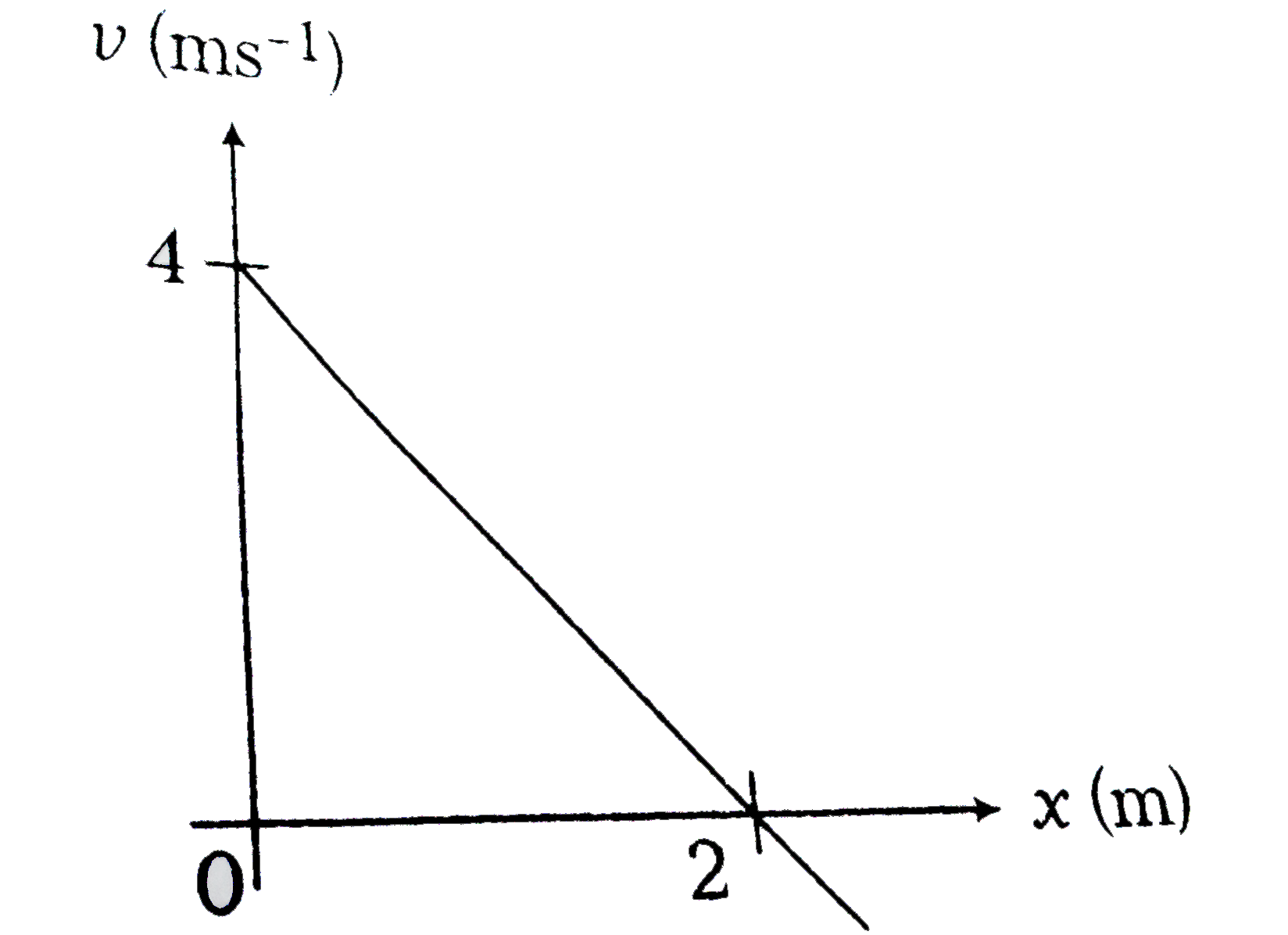
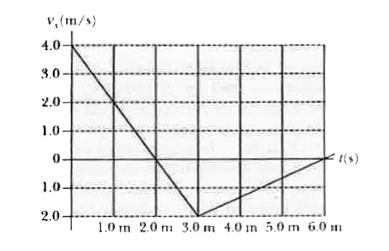
View Solutionvx is the velocity of a particle moving along the x-axis as shown in the figure. If x=2.0m at t=1.0s, what is the position of the particle at t=6.0s ?
View SolutionA particle constrained to move along x-axis given a velocity u along the positive x-axis. The acceleration ' a ' of the particle varies as a = - bx, where b is a positive constant and x is the x co-ordinate of the position of the particle . Then select the correct alternative(s): .
View Solution
