A projectile (mass = 0.20 kg) is fired at and embeds itself in a target (mass = 2.50 kg). The target (with the projectile in it) flies off after being struck. What percentage of the projectile's incident kinetic energy does the target (with the projectile in it) carry off after being struck?
2.1%
5.0%
3.8%
7.4%
The correct Answer is:D
To solve the problem step by step, we will use the principles of conservation of momentum and kinetic energy.
Step 1: Understand the System
We have a projectile with mass mp=0.20kg that embeds itself into a target with mass mt=2.50kg. After the collision, they move together with a common velocity V′.
Step 2: Conservation of Momentum
Since no external forces are acting on the system, momentum is conserved. The initial momentum of the system is equal to the final momentum.
mp⋅V=(mp+mt)⋅V′
Where V is the initial velocity of the projectile.
Step 3: Solve for Final Velocity V′
Rearranging the momentum conservation equation gives us:
V′=mp⋅Vmp+mt
Step 4: Calculate Initial Kinetic Energy of the Projectile
The initial kinetic energy KEi of the projectile is given by:
KEi=12mpV2
Step 5: Calculate Final Kinetic Energy of the Combined Mass
The final kinetic energy KEf of the target and projectile together is:
KEf=12(mp+mt)V′2
Substituting V′ from Step 3 into this equation:
KEf=12(mp+mt)(mp⋅Vmp+mt)2
Step 6: Simplify the Final Kinetic Energy Expression
KEf=12(mp+mt)⋅m2p⋅V2(mp+mt)2
KEf=12⋅m2p⋅V2mp+mt
Step 7: Calculate the Percentage of Kinetic Energy Carried Off
To find the percentage of the initial kinetic energy that the target carries off after being struck, we calculate:
Percentage=(KEfKEi)×100
Substituting the expressions for KEf and KEi:
Percentage=⎛⎜
⎜⎝12⋅m2p⋅V2mp+mt12mpV2⎞⎟
⎟⎠×100
Step 8: Simplify the Percentage Expression
Percentage=(m2pmp(mp+mt))×100
Percentage=(mpmp+mt)×100
Step 9: Substitute the Values
Substituting mp=0.20kg and mt=2.50kg:
Percentage=(0.200.20+2.50)×100
Percentage=(0.202.70)×100≈7.41%
Final Answer
The target (with the projectile in it) carries off approximately 7.41% of the projectile's incident kinetic energy after being struck.
---
We have a projectile with mass mp=0.20kg that embeds itself into a target with mass mt=2.50kg. After the collision, they move together with a common velocity V′.
Since no external forces are acting on the system, momentum is conserved. The initial momentum of the system is equal to the final momentum.
Rearranging the momentum conservation equation gives us:
The initial kinetic energy KEi of the projectile is given by:
The final kinetic energy KEf of the target and projectile together is:
KEf=12(mp+mt)⋅m2p⋅V2(mp+mt)2
To find the percentage of the initial kinetic energy that the target carries off after being struck, we calculate:
⎜⎝12⋅m2p⋅V2mp+mt12mpV2⎞⎟
⎟⎠×100
Percentage=(m2pmp(mp+mt))×100
Substituting mp=0.20kg and mt=2.50kg:
The target (with the projectile in it) carries off approximately 7.41% of the projectile's incident kinetic energy after being struck.
Topper's Solved these Questions
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (More than One Correct Choice)
5 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Linked Comprehension)
6 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Problems
46 Videos
- CAPACITANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PRACTICE QUESTION (INTEGER TYPE)
3 Videos
- CIRCUITSBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)
3 Videos
Similar Questions
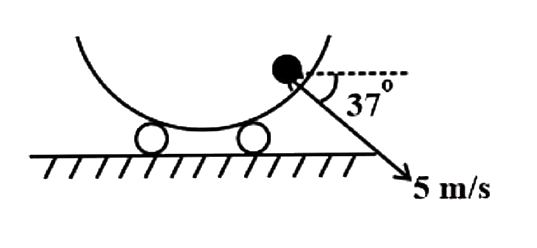
A projectile of mass 1.2 kg undergoes a perfectly inelastic collision with a trolley of mass 3.6 kg as shown in the figure. At the time of the collision, the second of the projectile is 5ms−1 at an angle of 37∘ with the horizontal. The trolley is free to move, only along a horizontal rail which coincides with the direction of the projectile's horizontal motion. Assuming that the trolley doesn't topple, calculate the amount of heat energy (in J) released in the collision.
[Take sin37∘=3/5andcos37∘=4/5]
View SolutionA cannon is mounted on a railway wagon which stands on a straight section of the track. The mass of the wagon with the cannon,projectiles and man is 2000 kg and mass of each projectile is 25 kg. The cannon is fired in a horizontal direction along the track, the projectiles having an initial velocity of 1000m/s with respect to the cannon (i) what should be the speed of the cannon after the first shot ? (ii) what should be the speed after the third shot?
View Solution
Knowledge Check
A projectile of mass 20 kg is fired with a velocity of 400m/sat an angle of 45∘ with the horizontal .At the highest point of the trajectocy the projectile explodes into two fragments of equal mass,one of which falls vertically downward with zero initial speed, The distance of the point where the other fragment falls from the point offiring is:
A24000 mB16000 mC32000 mD8000 mA projectile of mass 2kg has velocities 3m/s and 4ms at two points during its flight in the uniform gravitational field of the earth. If these two velocities are perpendicular to each other, then the minimum kinetic enerty of the particle during its flight is
A6.32JB8.40JC16.32JD5.76JA 2.0-kg projectile is fired with initial velocity components vox=30m/s and voy=40m/s from a point on the earth's surface. Neglect any effects due to air resistance.
What is the kinetic energy of the projectile when it reaches the highest point in its trajectory ?AzerojoulesB1600JC900JD2500J
A string of mass 2.50kg is under a tension os 200N. The length of the stretched string is 20.0m. If the transverse jerk is struck at one end of the string, how long does the disturbance take to reach the other end?
View SolutionA uniform square plate of mass 2.0 kg and edge 10 cm rotates about one of its diagonals under the action of a constant torque of 0.10 Nm. Calculate the angular momentum and the kinetic energy of the plate at the end of the fifth second after the start.
View SolutionA 24−kg projectile is fired at an angle of 53∘ above the horizontal with an initial speed of 50m/s. At the highest point in its trajectory, the projectile explodes into two fragments of equal mass, the first of which falls vertically with zero initial speed.
(a) How far from the point of firing does the second fragment strike the ground? (Assume the ground is level.)
(b) How much energy was released during the explosion?
View SolutionAn object of mass 5 kg is projected with a velocity of 20 m/s at angle of 60° to the horizontal. At the highest point of its path, the projectile explodes and breaks up into two fragments of masses 1 kg and 4 kg. The fragments separate horizontally after the explosion. The explosion releases internal energy such that the kinetic energy of the system at the highest point is doubled. If the separation between the two fragments when they reach the ground is x, then find the value of x5√3.
View SolutionA cannon shell is fired to hit a target at a horizontal distance d. However , it breaks into two parts of mass ratio 1:2 at its height point. The smaller part returns to cannon. The other part
(i) will fall at a distance d/2 beyond the target
(ii) have eight times the kinetic energy of smaller part
(iii) the increase in kinetic energy of system after explosion is 200%
(iv) after explosion , heavier part will take more time as compared to smaller part , to strike the ground
View Solution
