The head of a hammer (m = 1.5 kg) moving at 4.5 m/s strikes a nail and bounces back with the same speed after an elastic collision lasting 0.075 s. What is the magnitude of the average force the hammer exerts on the nail?
6.8 N
90 N
60 N
180 N
The correct Answer is:D
To solve the problem of finding the magnitude of the average force the hammer exerts on the nail during an elastic collision, we can follow these steps:
Step 1: Understand the problem
The hammer has a mass m=1.5kg and is moving at a speed of u=4.5m/s before striking the nail. After the collision, it bounces back with the same speed but in the opposite direction.
Step 2: Determine the initial and final velocities
- Initial velocity of the hammer before the collision:
u=−4.5m/s(downward direction)
- Final velocity of the hammer after the collision:
v=4.5m/s(upward direction)
Step 3: Calculate the change in momentum
The change in momentum (Δp) of the hammer can be calculated using the formula:
Δp=m(v−u)
Substituting the values:
Δp=1.5kg×(4.5m/s−(−4.5m/s))
Δp=1.5kg×(4.5+4.5)m/s
Δp=1.5kg×9m/s=13.5kg m/s
Step 4: Calculate the average force
The average force (Favg) exerted by the hammer on the nail can be calculated using the formula:
Favg=ΔpΔt
Where Δt=0.075s. Substituting the values:
Favg=13.5kg m/s0.075s
Favg=180N
Final Answer
The magnitude of the average force the hammer exerts on the nail is 180N.
---
The hammer has a mass m=1.5kg and is moving at a speed of u=4.5m/s before striking the nail. After the collision, it bounces back with the same speed but in the opposite direction.
- Initial velocity of the hammer before the collision:
u=−4.5m/s(downward direction)
- Final velocity of the hammer after the collision:
v=4.5m/s(upward direction)
The change in momentum (Δp) of the hammer can be calculated using the formula:
Δp=m(v−u)
Substituting the values:
Δp=1.5kg×(4.5m/s−(−4.5m/s))
Δp=1.5kg×(4.5+4.5)m/s
Δp=1.5kg×9m/s=13.5kg m/s
The average force (Favg) exerted by the hammer on the nail can be calculated using the formula:
Favg=ΔpΔt
Where Δt=0.075s. Substituting the values:
Favg=13.5kg m/s0.075s
Favg=180N
The magnitude of the average force the hammer exerts on the nail is 180N.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (More than One Correct Choice)5videos
CENTER OF MASS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Linked Comprehension)6videos
CENTER OF MASS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Problems46videos
CENTER OF MASS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PRACTICE QUESTION (INTEGER TYPE)3videos
CAPACITANCE
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
Similar Questions
A hammer of mass 500g, moving at 50m/s, strikes a nail. The nail stops the hammer in a very short time of 0.01s. What is the force of the nail on the hammer?
View SolutionA hammer of mass 500g, moving at 50m/s, strikes a nail. The nail stops the hammer in a very short time of 0.01s. What is the force of the nail on the hammer?
View Solution
Knowledge Check
- Question 1 - Select One
A ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
A2000NB1000NC5000ND125N - Question 2 - Select One
A ball of mass 0.5 kg moving with a velocity of 2ms−1 strikes a wall normally and bounces back with the same speed. If the time of contact between the ball and the wall is one millisecond, the average force exerted by the wall on the ball is
A2000 NB1000 NC5000 ND125 N - Question 3 - Select One
A 0.065 kg tennis ball moving to the right with a speed of 15 m/s is struck by a tennis racket, causing it to move to the left with a speed of 15 m/s. If the ball remains in contact with the racquet for 0.020 s, what is the magnitude of the average force experienced by the ball?
A0NB98 NC160 ND240 N
A 0.70 kg ball moving horizontally at 6.0 m/s strikes a vertical wall and rebounds with speed 3.5 m/s. What is the magnitude of the change in its linear momentum?
View SolutionA hammer of mass 500g, moving at 50m/s, strikes a nail. The nail stops the hammer in a very short time of 0.01s. What is the force of the nail on the hammer?
View SolutionA 3.00kg steel hall strikes the wall with a speed of 10.0m/s at an angle of 60∘ with the surface. It bounces off with the same speed and angle. If the ball is in contact with the wall for 0.200s, what is the average force exerted by the wall on the ball?
View SolutionA 3-kg steel ball strikes a wall with a speed of 10.0ms−1 at an angle of 60.0∘ with the surfaces of the wall. The ball bounces off with the same speed and same angle. If the ball was in contact with the wall for 0.2s, find the average force exerted by the wall on the ball.
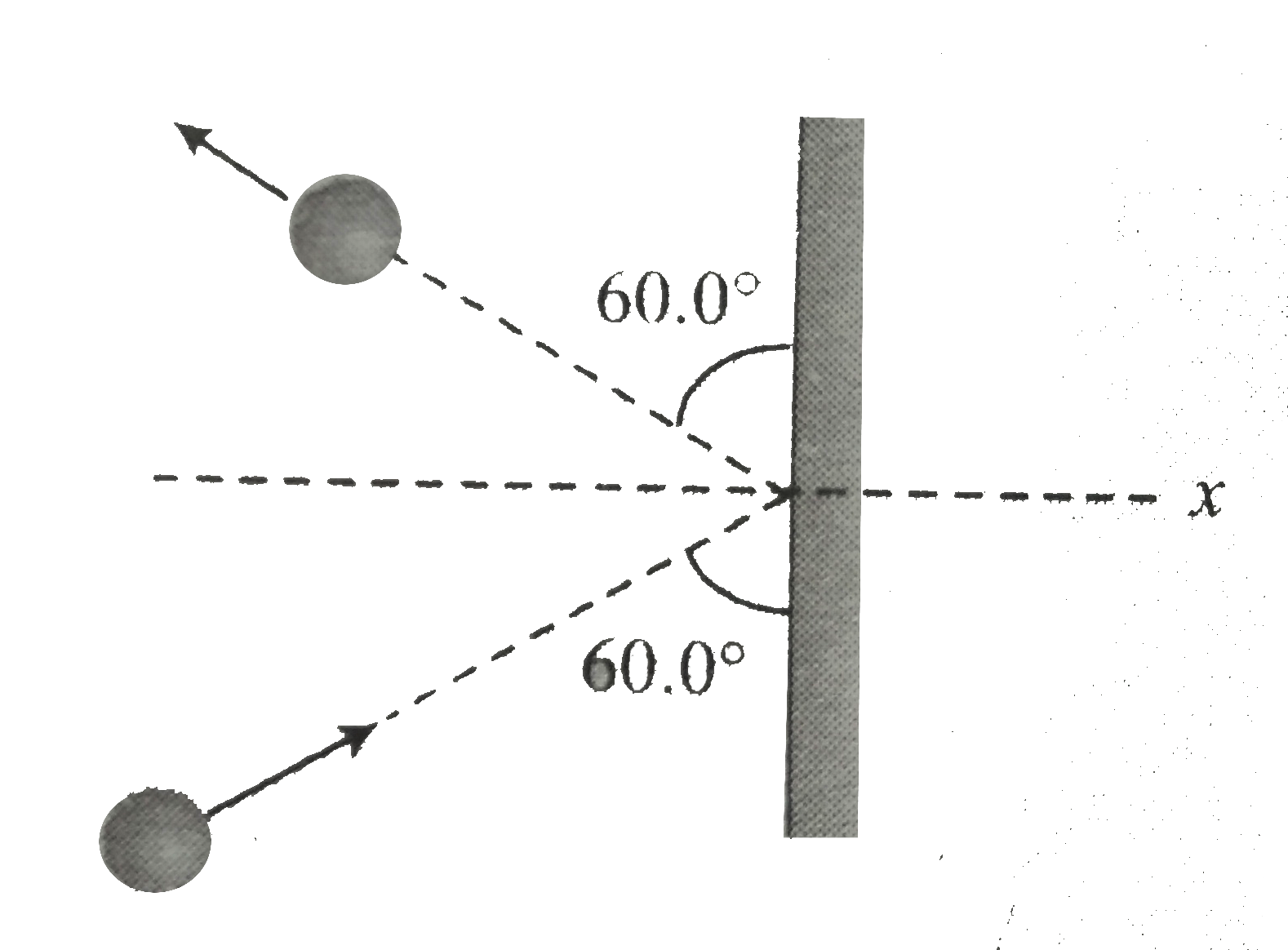 View Solution
View SolutionA ball of mass 250 g moving with 20 m/s strikes a vertical wall and rebounds along the same line with a velocity of 15 m/s. If the time of contact is 0.1 s, the force exerted by the wall on the ball is
View Solution

