A graph of the x component of the electric field as a function of x in a region of space is shown in Fig. 24-11. The scale of the vertical axis is set by Exs=20.0 N//C. The yandz components of the electric field are zero in this region. If the electric potential at the origin is 10 V, then:
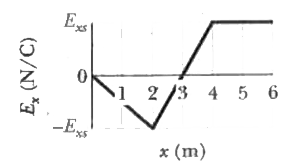
(a) What is the electric potential at x = 2.0 m?
(b) What is the greatest positive value of the electric potential for points on the x axis for which 0≤x≤6.0 m?
(c) For what value of x is the electric potential zero?
By Eq. 24-20, the change in potential is the negative of the "area" under the curve. Thus, using the area-of-a triangle formula, we have
V−10=∫x=20→E.d→s=12(2)(20)
which yields V = 30 V.
For any region within 0<x<3m−∫→E.d→s is positive, but for any region for which
x>3 m it is negative. Therefore, V=Vmax occurs at x=3 m.
V−10=−∫x=30→E.d→s=12320
which yields Vmax=40V.
In view of our result in part (b), we see that now (to find V = 0) we are looking for some X > 3 m such that the "area" from x = 3 m to x =Xis 40 V. Using the formula for a triangle (3 < x < 4) and a rectangle ( 4 < x < X), we require
12(1)(20)+(X−4)(20)=40
Therefore, X = 5.5 m.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.06 Finding the potential
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.07 Net potential of several charged particles
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.04 Finding the potential change from the electric field
1 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)
14 Videos
Similar Questions
A graph of the x-component of the electric field as a function of x in a region of space is shown in the figure.
They y and z-components of the electric field are zero in this region. The electric potential at the origin is 10 V.
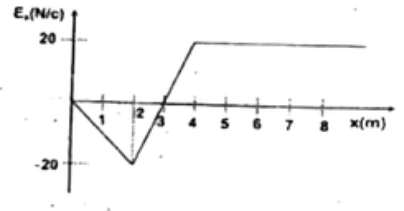
The greatest positive value of electric potential for points of the x-axis for which 0≤x≤6m is
View SolutionA graph of the x-component of the electric field as a function of x in a region of space is shown in figure. The y−andz−compo≠nts of the electric field are zero in this region. If the electric potential is 10 V at the origin, then the potential at x = 2.0 m is

View Solution
Knowledge Check
A graph of the x-component of the electric field as a function of x in a region of space is shown in figure. The y−andz−compo≠nts of the electric field are zero in this region. If the electric potential is 10 V at the origin, then the potential at x = 2.0 m is
 A10VB40VC−10VD30V
A10VB40VC−10VD30VIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
AConstantBZeroCPositiveDNegativeIn a certain region of space the electric potential V is known to be constant. Is the electric field in this region (a) positive (b) zero ,or (c ) negative ?
AAlso constantBPositiveCNegativeDZero
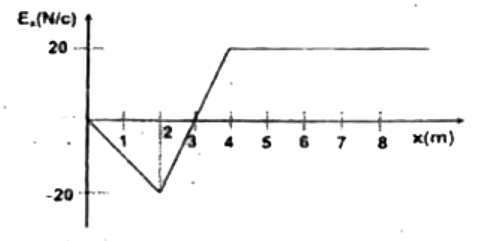
A graph of the x-component of the electric field as a function of x in a region of space is shown in the figure. They y and z-components of the electric field are zero in this region. The electric potential at the origin is 10 V. The value of x for which potential is zero is
View SolutionIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
View SolutionAn electric field →E=(25ˆi+30ˆj)NC−1 exists in a region of space.If the potential at the origin is taken to be zero then the potential at x=2 m y=2 m is :?
View SolutionThe electric potential as a function of distance 'x' is shown in the figure . Draw a graph of the electric field E as a function of x.

View SolutionThe electric field in a region is directed outward and is proportional to the distance r from the origin, Taking the electric potential at origin to be zero,
View Solution
