(a) In Fig. 24-17 a, 12 electrons ( of charge −e ) are equally spaced and fixed around a circle of radius R. Relative to V=0 at infinity, what are the electric potential and electric field at the center C of the circle due to these electrons?
(b) The electrons are moved along the circle until they are nonuniformly spaced over a 120° arc (Fig. 24-17b). At C, find the electric potential and describe the electric field.
(1) The electric potential V at C is the algebraic sum of the electric potentials contributed by all the electrons. Because electric potential is a scalar, the orientations of the electrons do not matter. (2) The electric field at C is a vector quantity and thus the orientation of the electrons is important.
Calculations: Because the electrons all have the same negative charge −e and are all the same distance R from C, Eq. 24-29 gives us
V=−1214πε0eR. (Answer) (24-30)
Because of the symmetry of the arrangement in Fig. 24-17 a, the electric field vector at C due to any given electron is canceled by the field vector due to the electron that is diametrically opposite it. Thus, at C,
→E=0. (Answer)
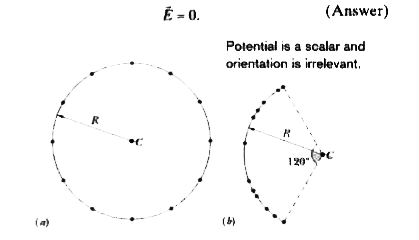
(b) Reasoning: The potential is still given by Eq. 24-30, because the distance between C and each electron is unchanged and orientation is irrelevant. The electric field is no longer zero, however, because the arrangement is no longer symmetric. A net field is now directed toward the charge distribution.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.09 Net potential due to two charged particles
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.10 Potential due to line of charge
1 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - SAMPLE PROBLEM 24.07 Net potential of several charged particles
1 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)
14 Videos
Similar Questions
A thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is

View SolutionFind the electric field due to an uniformly charged arc, at the center 'c'.
View Solution
Knowledge Check
Ten electrons are equally spaced and fixed around a circle of radius R. Relative to V=0 at infinity, the electrostatic potential V and the electric field E at the centre C are
AV≠0and→E≠0BV≠0and→E=0CV=0and→E=0DV=0and→E≠0In total, 10 charges (5 of them are +q each and other 5 are −q each) are placed randomly on the circumference of a circle. The radius of the circle is R. The electric potential at the center of this circle due to these charges will be
A0B10Kq/RC5Kq/RDCannot be calculated unless the positions of the charges on the circle are specified.An electron with charge e revolves in a circle of radius r. This constitutes a current equivalent to
AevrB2πevCev2πrD2πvev
An electron makes (3×105) revolutions per second in a circle of radius 0.5 angstrom. Find the magnetic field B at the centre of the circle.
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.
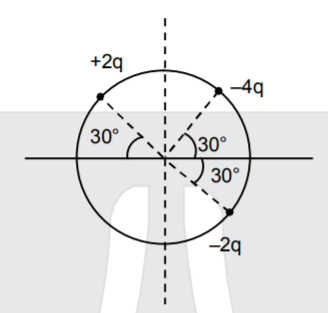
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.

View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.

View SolutionA thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is

View Solution
