[" The equation of a trajectory of a "],[" projectile is "],[[y=3x-0.02x^(2)," where "'x'" and "'y'],[" are in meters,range of projectile is "],[" (A) "150m],[" (B) "300m],[" (C) "450m],[" (D) "600m]]
Step by step video & image solution for [" The equation of a trajectory of a "],[" projectile is "],[[y=3x-0.02x^(2)," where "'x'" and "'y'],[" are in meters,range of projectile is "],[" (A) "150m],[" (B) "300m],[" (C) "450m],[" (D) "600m]] by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The equation of a trajectory of a projectile is y=8x−4x2. The maximum height of the projectile is ?
View Solution[" The equation of trajectory of a projectile is "],[y=ax-bx^(2)" where "x" and "y" axes are along "],[" horizontal and vertical directions "],[" respectively.The range of the projectile is "]
View Solution
Knowledge Check
- Question 1 - Select One
The equation of trajectory of an oblique projectile y=√3x−gx22. The angle of projection is
A30∘B45∘C60∘D15∘ - Question 2 - Select One
The equation of a projectile is y=√3x−(gx22) the horizontal range is
A2g√3B2√3gCg2√3D√3g2 - Question 3 - Select One
The equation of a projectile is y=√3x−gx22
the angle of projection is:-A30∘B60∘C45∘Dnone
Trajectory of particle in projectile motion is y=(x−x2/80), x and y are in metre.
Projectile range is on horizontal plane.
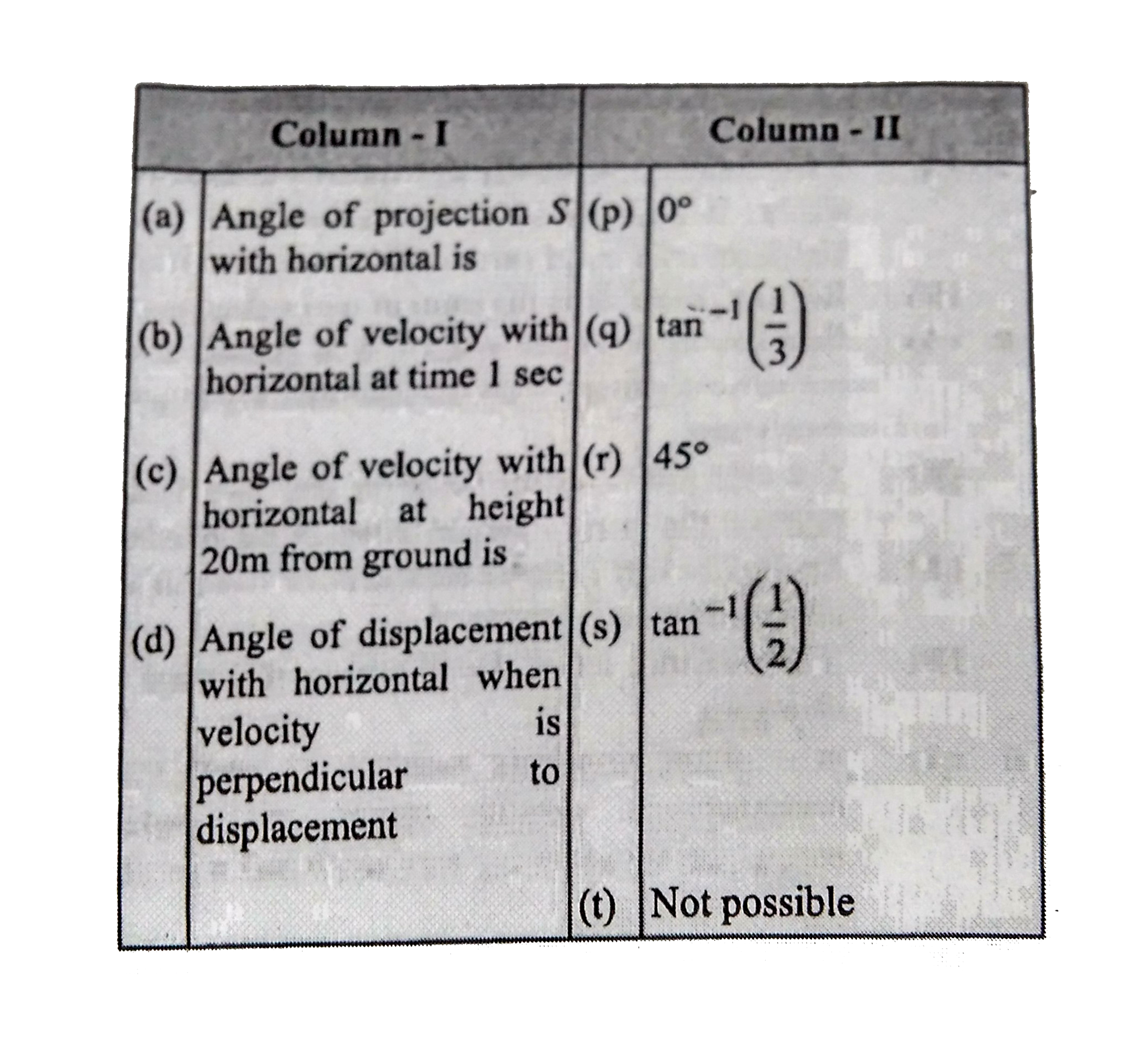 View Solution
View SolutionThe equation of trajectory of a projectile is y=10x-(5/9)x^2 if we assume g=10ms^(-3) then range of projectile (in metre) is
View SolutionThe equation of a trajectory of a projectile is y=8x-4x^(2) The maximum height of the projectile is (A) 8m (B) 6m (C) 2m (D) 4m
View SolutionThe equation of a progressive wave is y=7sin(4t−0.02x), where x and y are in cm time in second. The maximum velocity of a particle is……………
View SolutionThe equation of a projectile is y = ax - bx2. Its horizontal range is
View Solution
