In Fig. , the two light waves that are represented by the rays have wavelength 550.0 nm before entering media 1 and 2. They also have equal amplitudes and are in phase. Medium 1 is now just air, and medium 2 is a transparent plastic layer of index of refraction 1.600 and thickness 2.600 μm.
What is the phase difference of the emerging waves in wavelengths, radians, and degrees? What is their effective phase difference (in wavelengths)?
KEY IDEA
The phase difference of two light waves can change if they travel through different media, with different indexes of refraction. The reason is that their wavelengths are different in the different media. We can calculate the change in phase difference by counting the number of wavelengths that fits into each medium and then subtracting those numbers.
Calculations: When the path lengths of the waves in the two media are identical, Eq. 35-9 gives the result of the subtraction. Here we have n1=1.000 (for the air), n2=1.600, L = 2.600 mm, and λ=550.0nm. Thus, Eq. 35-9 yields
N2−N1=Lλ(n2−n1)
=2.600×10−6m5.500×10−7m(1.600−1.000)
= 2.84. (Answer)
Thus, the phase difference of the emerging waves is 2.84 wavelengths. Because 1.0 wavelength is equivalent to 2π rad and 360∘, you can show that this phase difference is equivalent to
phase difference = 17.8 rad ≈1020∘ (Answer)
The effective phase difference is the decimal part of the actual phase difference expressed in wavelengths. Thus, we have effective phase difference = 0.84 wavelength. (Answer)
You can show that this is equivalent to 5.3 rad and about 300∘. Caution: We do not find the effective phase difference by taking the decimal part of the actual phase difference as expressed in radians or degrees. For example, we do not take 0.8 rad from the actual phase difference of 17.8 rad.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - CHECKPOINT
6 Videos
- INTERFERENCE AND DIFFRACTION BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - PROBLEMS
61 Videos
- HYDROGEN ATOMBOOK - RESNICK AND HALLIDAYCHAPTER - HYDROGEN ATOMEXERCISE - PRACTICE QUESTIONS(Integer Type)
6 Videos
- MAGNETIC FIELDS DUE TO CURRENTSBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Practice Question (Integer Type)
4 Videos
Similar Questions
In Fig. , the two light waves that are represented by the rays have wavelength 550.0 nm before entering media 1 and 2. They also have equal amplitudes and are in phase. Medium 1 is now just air, and medium 2 is a transparent plastic layer of index of refraction 1.600 and thickness 2.600 μm.
If the waves reached the same point on a distant screen, what type of interference would they produce?
View SolutionLight of wavelength 6000Å in air enters a medium of refractive index 1.5. What will be its wavelength in the medium ?
View Solution
Knowledge Check
A ray of light is incident obliquely on medium 1 and then passes through two media namely 2 and 3 before emerging into medium 1 again.
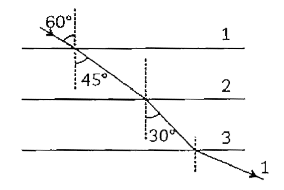
The refractive index of medium 3 respect to medium 1 is:A12B√32C1√2D√3A light of wavelength 6000 in air enters a medium of refractive index 1.5. Inside the medium , its frequency is v and it wavelength is λ.
Av=5×1014HzBv=7.5×1014HzCλ=4000DA=9000
Find the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View SolutionFind the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View SolutionA light wave of frequency 5×1014Hz enters a medium of refractive index 1.5. In the velocity of the light wave is ….. And its wavelength is ……
View SolutionA light wave of frequency 5×1014Hz enters a medium of refractive index 1.5. In the velocity of the light wave is ….. And its wavelength is ……
View SolutionWhat happens to the frequency, wavelength and speed of light that crosses from a medium with index of refraction μ1 to one with index of refraction μ2?
View SolutionWhat happens to the frequency, wavelength and speed of light that crosses from a medium with index of refraction μ1 to one with index of refraction μ2?
View Solution
