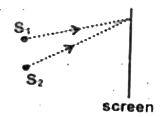
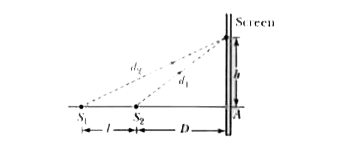
Two point sources oscillating in the same phase are on a straight line perpendicular to a screen. The nearest source is at a distance of D » $λ$ from the screen. What shape will the interference fringes have on the screen? What is the distance on the screen from the perpendicular to the nearest bright fringe if the distance between the sources is $l = n λ$ (n is an integer)? Note that $λ$ « l.

Text Solution

Verified by Experts

KEY IDEA
Here we first try to see why an interference pattern will be obtained. The interference pattern is observed because as seen on the screen, the paths of the waves coming from two sources are not equal.
Calculation: The intensity on the screen will be maximum when $d_{2} - d_{1} = k λ$ . The locus of points on the screen reached by rays from both sources with this difference is a circle with its center at point A. For this reason, the interference fringes will have the form of concentric circle.
When $I = n λ$ the intensity will be maximum at point A (an interference maximum of the nth order). The nearest bright interference fringe circle of the ${(n - 1)}^{th}$ order is at a distance from point A determined from the equation,
$d_{2} - d_{1} = \sqrt{{(n λ + D)}^{2} + h_{n - 1}^{2}} - \sqrt{D^{2} + h_{n - 1}^{2}} = (n - 1) λ$
Bearing in mind the conditions of the problem that h «D and $λ$ « l, we get
$h_{n - 1} ≅ \sqrt{\frac{2 D (D + n λ)}{n}} = \sqrt{2 D λ (\frac{D}{l} + 1)}$
Learn: One interesting aspect of this problem is that the order of maxima at any point other than the center will be less than n. Recall your mathematics classes. In any triangle, the third side is always longer than the difference of the other two sides. Since in the triangle formed by the two sources and point on the screen, the distance between two sources is $n λ$ , the path difference at any arbitrary point should be less than that.

Updated on:21/07/2023