Find the mean of a random variable X, whose probability density function is f(x)={λe−λxfor x≥00otherwise.Solution in Tamil
The correct Answer is:1λ
Step by step video, text & image solution for Find the mean of a random variable X, whose probability density function is f(x)={{:(lambdae^(-lambdax), "for " x ge 0), (0, "otherwise"):}. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER - 1 BOOK - FULL MARKSCHAPTER - SAMPLE PAPER - 1 EXERCISE - PART-III (Answer the questions)
10 Videos
- SAMPLE PAPER - 1 BOOK - FULL MARKSCHAPTER - SAMPLE PAPER - 1 EXERCISE - PART-IV (Answer the questions)
14 Videos
- SAMPLE PAPER - 1 BOOK - FULL MARKSCHAPTER - SAMPLE PAPER - 1 EXERCISE - PART-IV (Answer the questions)
14 Videos
- PROBABILITY DISTRIBUTIONSBOOK - FULL MARKSCHAPTER - PROBABILITY DISTRIBUTIONSEXERCISE - Additional Problems
27 Videos
- SAMPLE PAPER - 14 (UNSOLVED) BOOK - FULL MARKSCHAPTER - SAMPLE PAPER - 14 (UNSOLVED) EXERCISE - PART-IV IV. Answer all the questions.
14 Videos
Similar Questions
Find the inverse of [2−15−2] by Gauss Jorden method.
View SolutionBy using Gaussian elimination method, balance the chemical reaction equation:
C2H6+O2→H2O+CO2.
View SolutionBy using Gaussian elimination method, balance the chemical reaction equation:
C2H6+O2→H2O+CO2.
View SolutionFour defective oranges are accidentally mixed with sixteen good ones . Two oranges are drawn at random from the mixed lot. If the random variable X denotes the number of defective oranges then find the values of X and number of points in its iverse image.
View SolutionFind the condition on a, b and c so that the following system of linear equations has one parameter family of solutions: x + y + z = a, x + 2y + 3z = b, 3x + 5y + 7z = c.
View SolutionLet X is a continuous random variable with probability density function
f(x)={x6+k0≤x≤30 otherwise
The value of k is equal to
View SolutionThe following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Find the expression for c.d.f (Cumulative Distribution Function ) of X
View SolutionThe following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Also, find its value at x = 0.5 and 9.
View SolutionShow that the function f(x) defined by .f(x)=17, for 1≤x≤8 =0, otherwise, is a probability density function for a random variable. Hence find P(3<X<10)
View SolutionIf the probability density function of a random variable X is given as
then F(0) is equal to
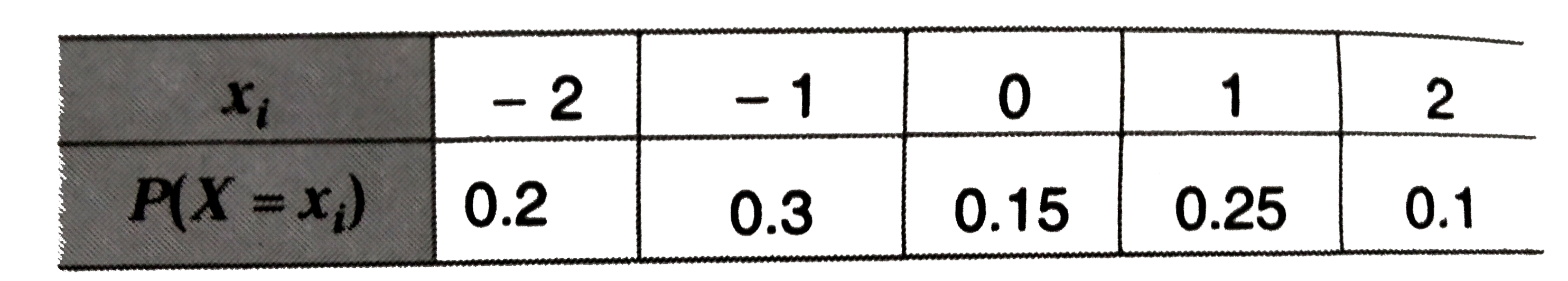
View SolutionIf f(x)= kx, 0 lt x lt 2 = 0 otherwise, is a probability density function of a random variable X , then find (i) value of k, (ii) P(1 lt x lt2).
View SolutionIf f(x)={2x0≤x≤a0otherwise is a probability density function of a random variable, then the value of a is
View SolutionFind the mean of a random variable X, whose probability density function is f(x)={λe−λxfor x≥00otherwise.
View SolutionIf x is the random variable with probability mass function given by
f(x)=⎧⎪⎨⎪⎩x+11≤x<2−x+12≤x<30otherwise
Find The distrubution function find also
View SolutionFind the mean and variance of a random variable X , whose probability function is f(x) = {λe−λxfor x≥00otherwise
View SolutionIf f(x) = {2x0≤x≤a0otherwise is a probability density function of a random variable then the value of a is ……….. .
View Solution
