Question

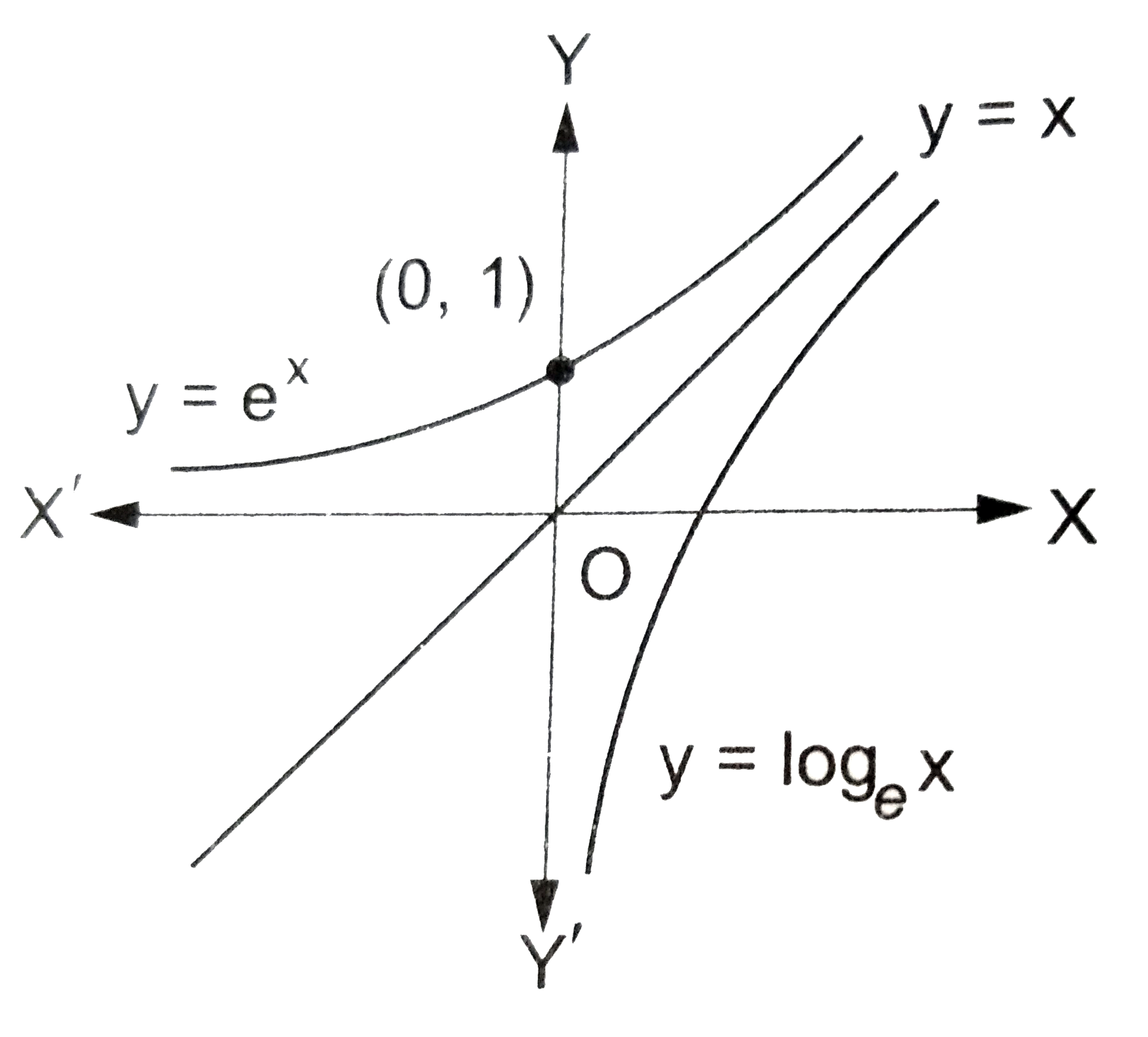
On the same draw the graphs of $e^x\text{and}{\log }_{e}x$.

Explanation

Since e lies between 2 and 3. it follows that ` e gt 1`. So, it is a particular case of ` a^(x`), where ` a gt 1` , the function ` e^(x)` is strictly increasing . Also, its range is R. So, the funcation is one-one onto and therefore invertible.
The graph passes through the point (0,1) and comes closer and closer to the x-axis and the values of x decrease. thus , the grraph of ` e^(x)` may be drawn as shown in the figure. its reflection in the line y=x gives of `log_(e)` x.