Question

Draw the graph of $y={\log }_e\left(\sin x\right).$

Explanation

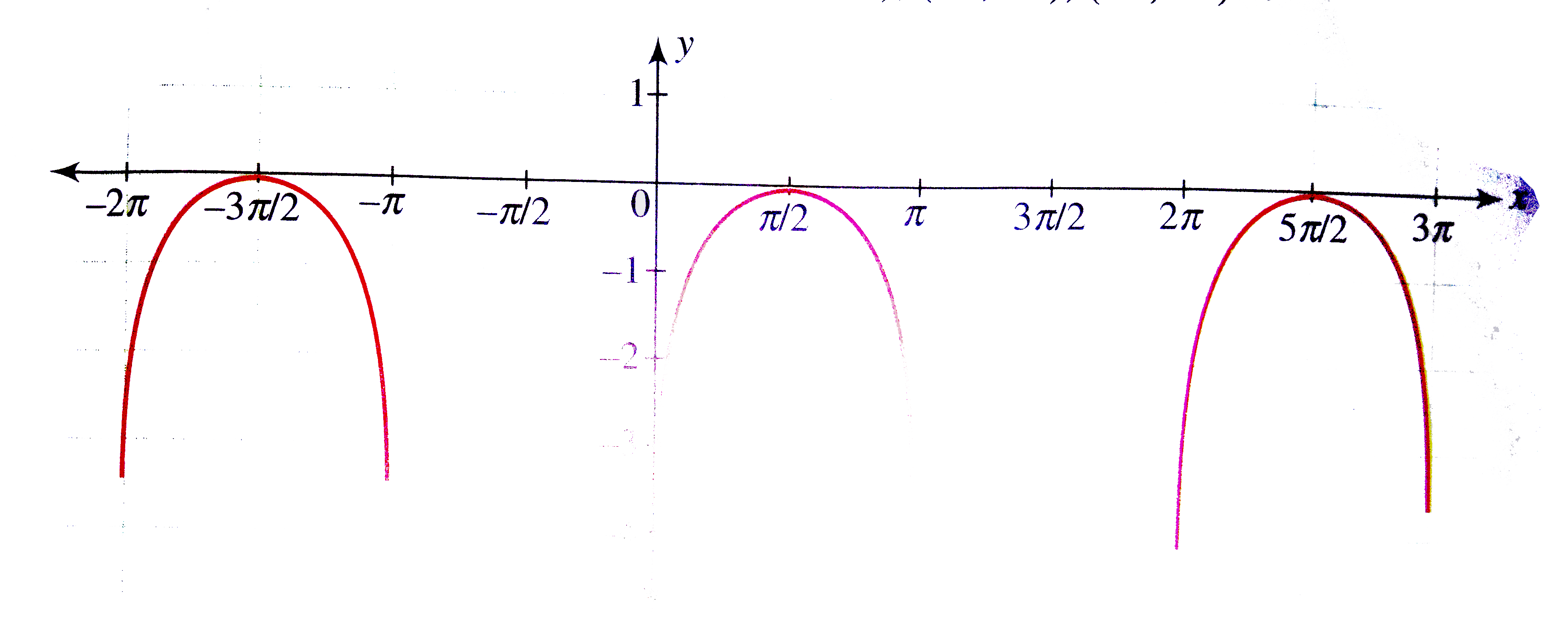
We have `y = f(x) = log_(e) (sin x)`
Clearly, y = f(x) is defined when `sin x gt 0`, i.e. x lies in the `1^(st)` and `2^(nd)` quadrants only expect the quadrant angle.
Also we have `0 lt sin x le 1`
`therefore` `-oo lt log_(e)(sin x) le 0`
Period of y = f(x) is `2pi`. However, the function is not defined in `(pi, 2pi)`. The graph is for `(0, pi)` only.
Now when `x to 0^(+) or x to pi^(-), sin x to 0^(+)`, for which `log_(e)(sin x) to -oo`
Also `f(pi//2) = log_(e)(1) = 0`
`f'(x) = cot x,`
`f''(x) - "cosec"^(2)x lt 0`
Hence the graph is concave downwards.
The graph of the function for `x in (0, pi)` is as shown in the following figure.

We have same graphs of intervals `... (-2pi, - pi), (2pi, 3pi), (4pi, 5pi)...`