The area of the triangle formed by the coordinates axes and $a$ line is $6$ $s q u a r e$ units and the length of the hypotenuse is $5 u n i t s .$ Find the equation of the line.

Video Solution

Text Solution

Verified by Experts

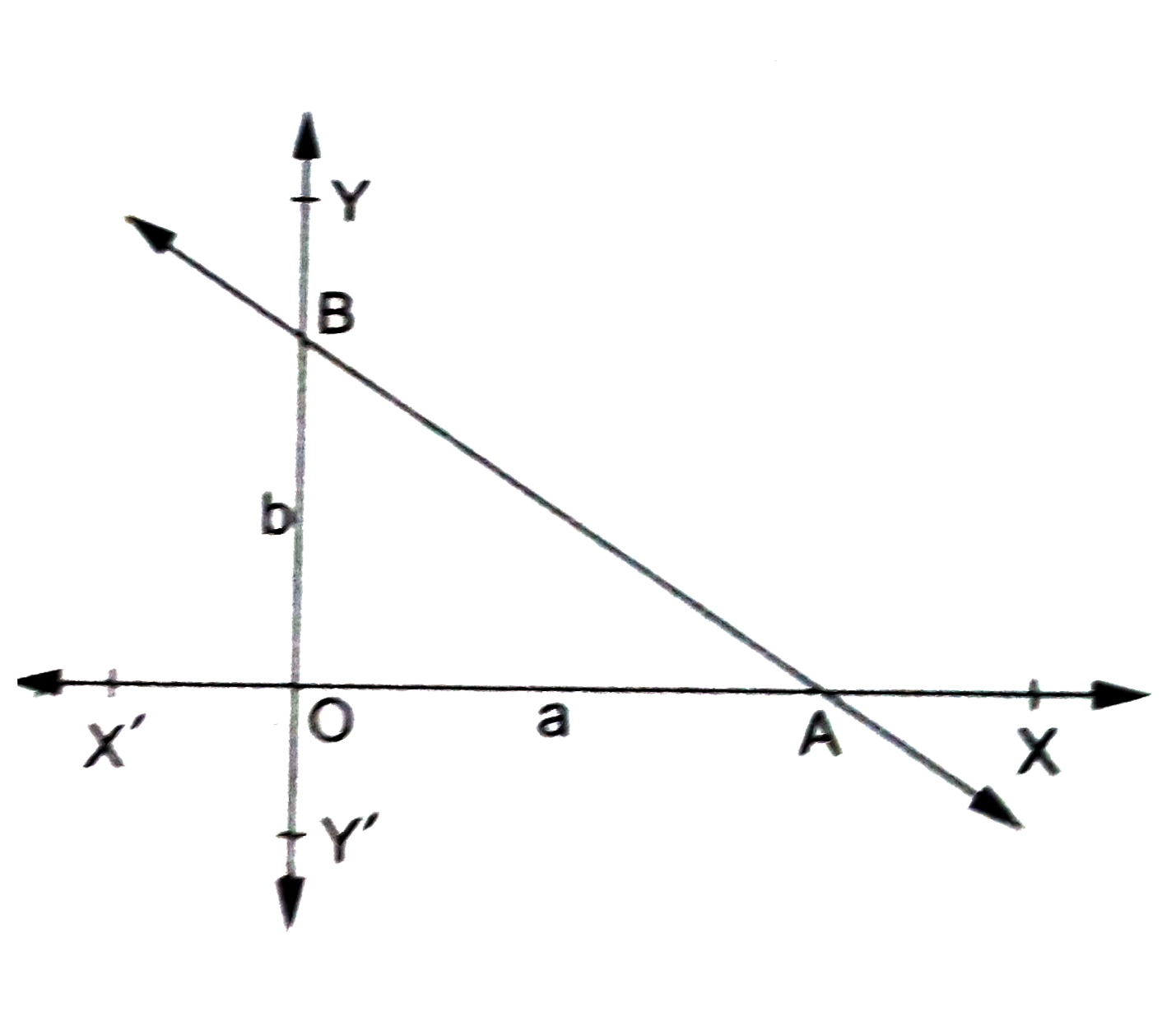
Let X'OX and YOY' be the coordinate axes, and let AB be the part of the given line intercepted by the axes.
Let the OA=a and OB=b
Then, $\frac{1}{2}, a b = 6 \Leftrightarrow a b = 12 \dots . (i)$
and $a^{2} + b^{2} = 25 \dots \dots \dots (i i)$
Putting $b = \frac{12}{a}$ from (i) in (ii), we get $a^{2} + \frac{144}{a^{2}} = 25 \Rightarrow a^{4} - 25 a^{2} + 144 = 0$
$\Rightarrow (a^{2} - 16) (a^{2} - 9) = 0 \Rightarrow a^{2} = 16 or a^{2} = 9$
$\Rightarrow a = 4 or a = 3 [∴ length is always positive]$
Hence, the required equation of the line is
$\frac{x}{4} + \frac{y}{3} = 1 or \frac{x}{3} + \frac{y}{4} = 1$
$i . e . 3 x + 4 y - 12 = 0 or 4 x + 3 y - 12 = 0$

Updated on:21/07/2023