Gives the potential energy function U(r) of a projectile, plotted outward from the surface of a planet of radius Rs. If the projectile is launched radially outward from the surface with a mechanical energy of −2.0×109 J, what are (a) its kinetic energy at radius r=1.15Rs, and (b) its turning point (see Section 8.4) in terms of Rs?
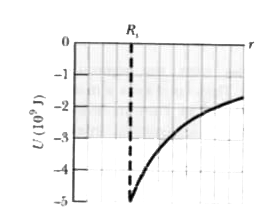
(a) ≈2.3×109J, (b) r = 2.17 (1.15Rs) = 2.5Rs
Topper's Solved these Questions
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
16 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
7 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - CHECKPOINT
5 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(Integers)
4 Videos
- HEAT-MEASUREMENT AND TRANSFERBOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - PRACTICE QUESTIONS( INTEGER TYPE)
5 Videos
Similar Questions
What is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
View SolutionFor a satellite at a distance 11R from the surface of a planet P of radius R. Its time period is 24hrs. Evaluate time period of another satellite at a distance 2R from surface of P
View Solution
Knowledge Check
What is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
A5GmM6RB2GmM3RCGmM2RDGmM3RWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
A5GmM6RB2GmM3RCGmM2RDGmM2RWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 3R ?
A7GmM8RB2GmM3RCGmM2RDGMm2R
What is the potential energy of a body of mass m relative of the surface of Earth of radius R, at a (a) height h=R above its surface (b) depth d=R below its surface.
View SolutionA projectile is projected tangentially from the surface of a planet of radius R. If it is at a height of 3R at the farthest point of its trajectory, then the velocity of projection V0 is given by ( acceleration due to gravity on surface=g)
View SolutionThe potential energy function of a particle is given by U(r)=A2r2−B3r, where A and B are constant and r is the radial distance from the centre of the force. Choose the correct option (s)
View SolutionA projectile is projected tangentially from the surface of a planet of radius R. If it is at a height of 3R at the farthest point of its trajectory, then the velocity of projection V0 is given by ( acceleration due to gravity on surface=g)
View SolutionThe potential energy function of a particle is given by U(r)=A2r2−B3r, where A and B are constant and r is the radial distance from the centre of the force. Choose the correct option (s)
View Solution
